Demostrar trascendencia. - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Álgebra > Polinomios

Reply to this topic

Start new topic

Demostrar trascendencia.

juanpamat Ver Perfil del Miembro Ver los mensajes de este miembro	May 15 2019, 08:13 AM Publicado: #1
Dios Matemático Grupo: Usuario FMAT Mensajes: 373 Registrado: 29-December 08 Desde: santiago Miembro Nº: 41.467 Nacionalidad: Colegio/Liceo: Sexo:	Demostrar que el número 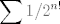$TEX: $a$$ = $TEX: $\displaystyle \sum 1/2^{n!}$$ es trascendente. ----- Algo de Historia: 1844: Liouville showed that certain numbers, now called Liouville numbers, are transcendental. 1873: Hermite showed that $TEX: $e$$ is transcendental. 1874: Cantor showed that the set of algebraic numbers is countable, but that 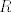$TEX: $R$$ is not countable. Thus most numbers are transcendental (but it is usually very difficult to prove that any particular number is transcendental). 1882: Lindemann showed that 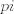$TEX: $\pi$$ is transcendental. 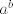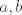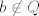1934: Gel’fond and Schneider independently showed that $TEX: $a^b$$ is trascendental if $TEX: $a,b$$ son algebraic with $TEX: $a \not=0,1$$ and $TEX: $b \not \in Q$$ (This was the seventh of Hilbert’s famous problems.) 2004: Euler’s constant has not yet been proven to be transcendental or even irrational. 2004: The numbers 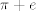$TEX: $\pi + e$$ and $TEX: $e-\pi$$ are surely transcendental, but again they have not even been proved to be irrational Mensaje modificado por juanpamat el May 15 2019, 08:14 AM -------------------- * "Las matemáticas son el alfabeto con el cual Dios ha escrito el Universo" * "Las matemáticas son el lenguaje de la naturaleza." Galileo Galilei.

hermite Ver Perfil del Miembro Ver los mensajes de este miembro	May 15 2019, 10:16 AM Publicado: #2
Maestro Matemático Grupo: Usuario FMAT Mensajes: 128 Registrado: 27-November 15 Miembro Nº: 142.558	CITA(juanpamat @ May 15 2019, 08:13 AM) Demostrar que el número 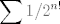$TEX: $a$$ = $TEX: $\displaystyle \sum 1/2^{n!}$$ es trascendente. ----- Algo de Historia: 1844: Liouville showed that certain numbers, now called Liouville numbers, are transcendental. 1873: Hermite showed that $TEX: $e$$ is transcendental. 1874: Cantor showed that the set of algebraic numbers is countable, but that 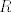$TEX: $R$$ is not countable. Thus most numbers are transcendental (but it is usually very difficult to prove that any particular number is transcendental). 1882: Lindemann showed that 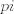$TEX: $\pi$$ is transcendental. 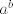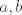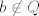1934: Gel’fond and Schneider independently showed that $TEX: $a^b$$ is trascendental if $TEX: $a,b$$ son algebraic with $TEX: $a \not=0,1$$ and $TEX: $b \not \in Q$$ (This was the seventh of Hilbert’s famous problems.) 2004: Euler’s constant has not yet been proven to be transcendental or even irrational. 2004: The numbers 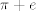$TEX: $\pi + e$$ and $TEX: $e-\pi$$ are surely transcendental, but again they have not even been proved to be irrational la ultima nota historica no esta correcta. No se sabe si ninguno de esos dos es trascendente, lo que se sabe ( y es trivial de demostrar) es que al menos uno de los dos debe ser trascendente. La ultima parte esta correcta,

Uchiha_Madara Ver Perfil del Miembro Ver los mensajes de este miembro	May 15 2019, 11:43 AM Publicado: #3
Matemático Grupo: Usuario FMAT Mensajes: 49 Registrado: 26-May 18 Miembro Nº: 157.415 Nacionalidad: Sexo:	QUOTE(juanpamat @ May 15 2019, 08:13 AM) Demostrar que el número 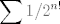$TEX: $a$$ = $TEX: $\displaystyle \sum 1/2^{n!}$$ es trascendente. ----- Algo de Historia: 1844: Liouville showed that certain numbers, now called Liouville numbers, are transcendental. 1873: Hermite showed that $TEX: $e$$ is transcendental. 1874: Cantor showed that the set of algebraic numbers is countable, but that 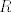$TEX: $R$$ is not countable. Thus most numbers are transcendental (but it is usually very difficult to prove that any particular number is transcendental). 1882: Lindemann showed that 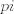$TEX: $\pi$$ is transcendental. 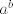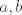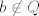1934: Gel’fond and Schneider independently showed that $TEX: $a^b$$ is trascendental if $TEX: $a,b$$ son algebraic with $TEX: $a \not=0,1$$ and $TEX: $b \not \in Q$$ (This was the seventh of Hilbert’s famous problems.) 2004: Euler’s constant has not yet been proven to be transcendental or even irrational. 2004: The numbers 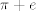$TEX: $\pi + e$$ and $TEX: $e-\pi$$ are surely transcendental, but again they have not even been proved to be irrational Esto es numero de lioville. -------------------- Estudiante de Ingeniería

« Posterior · Polinomios · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 09:11 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.