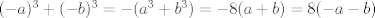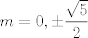|
|
|
|
|
|
|
  |
 Sep 23 2016, 10:37 PM Sep 23 2016, 10:37 PM
Publicado:
#1
|
|
|
Dios Matemático Grupo: Team Ensayos FMAT Mensajes: 274 Registrado: 30-December 09 Miembro Nº: 64.740 Nacionalidad:  Colegio/Liceo:  Sexo:  |
The 32st. Austrian Mathematical Olympiad OEMO 2001 - Begginer's Competition Problema 1. Pruebe que para todo entero positivo impar  el número el número  es divisible por 24. es divisible por 24.Problema 2. Consideremos la ecuación cuadrática 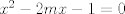 , donde , donde  es un número real arbitrario. Para qué valores de es un número real arbitrario. Para qué valores de  la ecuación tiene dos soluciones reales, de manera que la suma de sus cubos es igual a ocho veces su suma. la ecuación tiene dos soluciones reales, de manera que la suma de sus cubos es igual a ocho veces su suma.Problema 3. Determine todos los números reales  tales que tales que 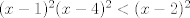 . .Problema 4. Sea 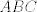 un triángulo cuyos ángulos un triángulo cuyos ángulos 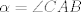 y y 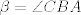 son mayores que son mayores que 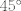 . Por encima del lado . Por encima del lado  se construye un triángulo isósceles rectángulo se construye un triángulo isósceles rectángulo 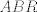 con con 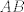 como hipotenusa, tal que como hipotenusa, tal que 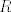 se encuentra en el interior del triángulo se encuentra en el interior del triángulo 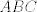 . Análogamente construimos por encima de los lados . Análogamente construimos por encima de los lados 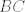 y y 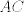 los triángulos isósceles rectángulos los triángulos isósceles rectángulos 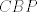 y y 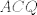 , rectángulos en , rectángulos en 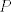 y en y en 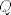 , pero con estos fuera del triángulo , pero con estos fuera del triángulo 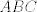 . Demostrar que . Demostrar que 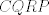 es un paralelogramo. es un paralelogramo.Mensaje modificado por einstenio16 el Oct 7 2016, 12:06 PM -------------------- He vuelto con las pilas cargaditas!!! |
|
|
|
 Sep 25 2016, 09:15 AM Sep 25 2016, 09:15 AM
Publicado:
#2
|
|
 Dios Matemático Supremo Grupo: Super Moderador Mensajes: 1.062 Registrado: 16-May 05 Desde: Industrias (USACH) Miembro Nº: 35 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Hola:
Para los que se motiven, les dejo algunos "hints" Veamos si alguien resuelve alguno! Saludos. -------------------- |
|
|
|
 Sep 28 2016, 08:56 PM Sep 28 2016, 08:56 PM
Publicado:
#3
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 817 Registrado: 21-February 14 Miembro Nº: 127.064 |
The 32st. Austrian Mathematical Olympiad OEMO 2001 - Begginer's Competition Problema 3. Determine todos los números reales  tales que tales que 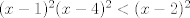 . .Esto es equivalente a resolver 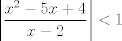 Aplicando propiedad del valor absoluto en una desigualdad 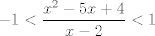 separando 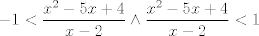 es decir debemos resolver es decir debemos resolver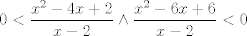 aplicando teorema del signo 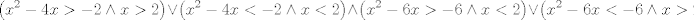 para el primer parentesis 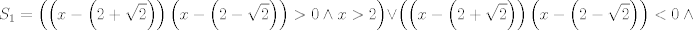 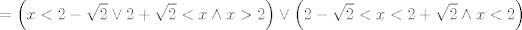 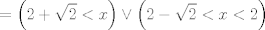 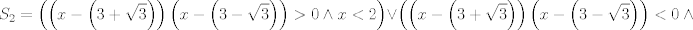 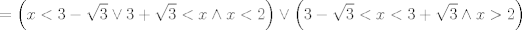 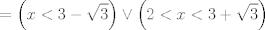 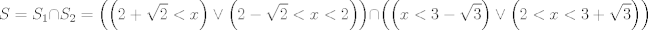 
|
|
|
|
 Sep 28 2016, 09:29 PM Sep 28 2016, 09:29 PM
Publicado:
#4
|
|
 Dios Matemático Supremo Grupo: Super Moderador Mensajes: 1.062 Registrado: 16-May 05 Desde: Industrias (USACH) Miembro Nº: 35 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
-------------------- |
|
|
|
 Sep 29 2016, 07:42 PM Sep 29 2016, 07:42 PM
Publicado:
#5
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 817 Registrado: 21-February 14 Miembro Nº: 127.064 |
era mas rapido hacer
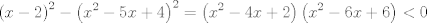
|
|
|
|
 Sep 30 2016, 07:12 AM Sep 30 2016, 07:12 AM
Publicado:
#6
|
|
 Dios Matemático Supremo Grupo: Super Moderador Mensajes: 1.062 Registrado: 16-May 05 Desde: Industrias (USACH) Miembro Nº: 35 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
era mas rapido hacer 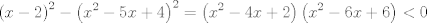 En efecto, esa es la forma más rápida al parecer. Es un buen caso de uso de la suma por diferencia. Saludos! -------------------- |
|
|
|
 Oct 7 2016, 12:01 PM Oct 7 2016, 12:01 PM
Publicado:
#7
|
|
 Maestro Matemático Grupo: Usuario FMAT Mensajes: 110 Registrado: 8-February 16 Miembro Nº: 143.585 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Problema 2
|
|
|
|
 Oct 7 2016, 12:05 PM Oct 7 2016, 12:05 PM
Publicado:
#8
|
|
|
Dios Matemático Grupo: Team Ensayos FMAT Mensajes: 274 Registrado: 30-December 09 Miembro Nº: 64.740 Nacionalidad:  Colegio/Liceo:  Sexo:  |
-------------------- He vuelto con las pilas cargaditas!!! |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 24th October 2025 - 04:08 AM |












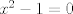 con soluciones 1 y -1 se verifica que:
con soluciones 1 y -1 se verifica que: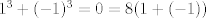
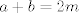

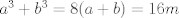
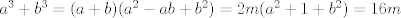
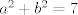
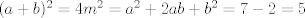
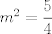
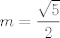
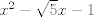 con soluciones:
con soluciones: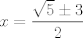
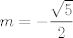 son -a y -b
son -a y -b