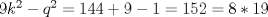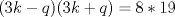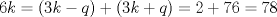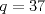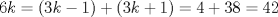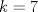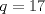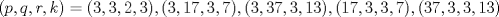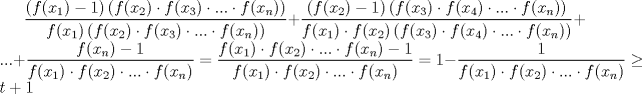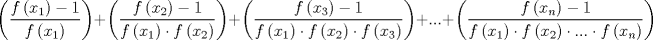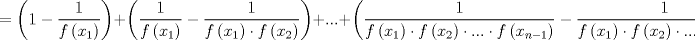|
|
|
|
|
|
|
  |
 Aug 1 2015, 04:06 PM Aug 1 2015, 04:06 PM
Publicado:
#1
|
|
 Maestro Matemático Grupo: Usuario FMAT Mensajes: 81 Registrado: 10-November 12 Miembro Nº: 112.735 Nacionalidad:  Colegio/Liceo:  Universidad: .png) Sexo:  |
P1. Sean
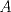 , , 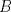 , , 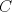 , , 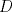 cuatro puntos distintos en una circunferencia tales que las rectas cuatro puntos distintos en una circunferencia tales que las rectas 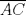 y y 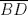 se intersectan en un punto se intersectan en un punto 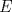 , las rectas , las rectas 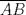 y y 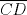 no son paralelas, y las rectas no son paralelas, y las rectas 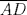 y y 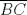 se intersectan en un punto se intersectan en un punto 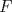 . Pruebe que . Pruebe que 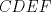 es cíclico, si y solo si las rectas es cíclico, si y solo si las rectas 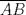 y y 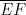 son perpendiculares. son perpendiculares. P2. Sean 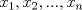 una secuencia de reales no negativos y una secuencia de reales no negativos y  una constante real positiva. Sea una constante real positiva. Sea 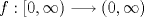 una función que satisface una función que satisface 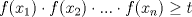 . Encuentre el menor valor para la expresión: . Encuentre el menor valor para la expresión: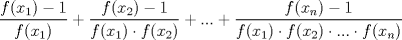 P3. Encuentre todos los primos 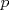 , , 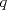 , ,  , y los enteros positivos , y los enteros positivos 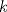 tales que: tales que: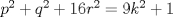 Mensaje modificado por asdayuyi el Aug 1 2015, 04:09 PM |
|
|
|
 Aug 1 2015, 05:46 PM Aug 1 2015, 05:46 PM
Publicado:
#2
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 783 Registrado: 23-April 13 Desde: Constitución Miembro Nº: 118.027 Nacionalidad:  Universidad:  Sexo:  |
P1
--------------------    |
|
|
|
 Aug 1 2015, 10:20 PM Aug 1 2015, 10:20 PM
Publicado:
#3
|
|
 Dios Matemático Supremo Grupo: Colaborador Silver Mensajes: 648 Registrado: 26-October 13 Desde: Tokyo-3 Miembro Nº: 123.749 Nacionalidad:  Sexo:  |
P3:
-------------------- Pro Tip: Es siempre recomendable saltarse los posts de Insanee/Legition I wish, that I could turn back time 'cos now the guilt is all mine can't live without the trust from those you love I know we can't forget the past you can't forget love & pride because of that, it's killing me inside |
|
|
|
 Aug 3 2015, 09:46 PM Aug 3 2015, 09:46 PM
Publicado:
#4
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 325 Registrado: 18-March 14 Miembro Nº: 127.725 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
P2. Sean 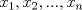 una secuencia de reales no negativos y una secuencia de reales no negativos y  una constante real positiva. Sea una constante real positiva. Sea 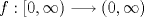 una función que satisface una función que satisface 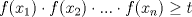 . Encuentre el menor valor para la expresión: . Encuentre el menor valor para la expresión: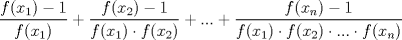 haciendo minimo común multiplo queda: 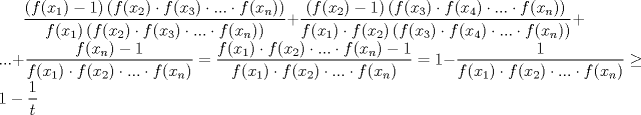 edit:ultimo paso Mensaje modificado por Adrianocor el Aug 4 2015, 11:48 AM |
|
|
|
 Aug 4 2015, 11:39 AM Aug 4 2015, 11:39 AM
Publicado:
#5
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 817 Registrado: 21-February 14 Miembro Nº: 127.064 |
|
|
|
|
 Aug 4 2015, 11:47 AM Aug 4 2015, 11:47 AM
Publicado:
#6
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 325 Registrado: 18-March 14 Miembro Nº: 127.725 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
jaja tienes razon, la vendi en el ultimo paso
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 22nd October 2025 - 03:08 PM |










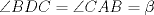 ,
, 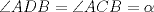 ,
, 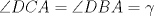 y
y  , entonces, se tiene que
, entonces, se tiene que 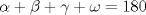 , como se quiere que ECFD sea cíclico, entonces
, como se quiere que ECFD sea cíclico, entonces 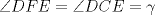 y además
y además 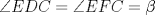 . Sea
. Sea 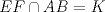 , es fácil ver que
, es fácil ver que 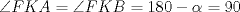 , de donde se concluye que ECFD es cíclico si y solo si
, de donde se concluye que ECFD es cíclico si y solo si 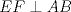 .
. 

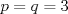 . Tenemos entonces
. Tenemos entonces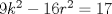
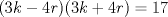
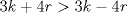 tenemos
tenemos 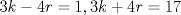 que implica
que implica 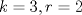 . Tenemos entonces la solución
. Tenemos entonces la solución 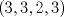
 (el caso
(el caso 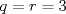 es análogo). Tenemos entonces
es análogo). Tenemos entonces