|
|
|
|
|
|
|
  |
 Oct 26 2013, 07:24 PM Oct 26 2013, 07:24 PM
Publicado:
#11
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 195 Registrado: 26-December 10 Desde: Nuncajamás. Miembro Nº: 82.286 Nacionalidad:  Sexo:  |
Pendiente. (Se agradecen aportes.)
Mensaje modificado por TribalJazz2 el Oct 27 2013, 03:51 PM -------------------- |
|
|
|
 Oct 26 2013, 07:28 PM Oct 26 2013, 07:28 PM
Publicado:
#12
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 195 Registrado: 26-December 10 Desde: Nuncajamás. Miembro Nº: 82.286 Nacionalidad:  Sexo:  |
Ingeniería Matemática Facultad de Ciencias Físicas y Matemáticas Álgebra Lineal 12-2 Control 1 Semestre Primavera 2012
 y y 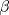 son parámetros reales. son parámetros reales. ![TEX: \[\begin{matrix}<br />2x_1 & +x_2 & +(1-2\alpha)x_3 & (\beta +1)x_4 = \beta -3\\<br /> & x_2 & -x_3 & +(\beta - \alpha)x_4 = -1\\<br /> & -2x_2 & +2x_3 & +(2-2\beta)x_4 = -2\\<br />2x_1 & & +2x_3 & +\alpha x_4 = 4\beta -3\\<br />2x_1 & +x_2 & +x_3 & +(\alpha +\beta -1) x_4 = 0<br />\end{matrix}\]](/tex-image/540eb77411c3afe8844c9d412da05e6e.png)  y y 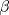 para que el sistema: para que el sistema:
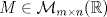 una matriz tal que una matriz tal que 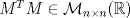 es invertible. Se define la matriz es invertible. Se define la matriz 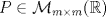 como como ![TEX: \[P=I-M(M^T M)^{-1} M^T\]](/tex-image/c02368fc3d3732564481f4563410ac29.png) donde I es la identidad de dimensión m. Pruebe que
a.2) Las matrices MTM y P son simétricas. a.3) P no es invertible (Ind: Argumente que M no es nula). ![TEX: \[\Pi : \begin{pmatrix}<br />x\\<br />y\\<br />z<br />\end{pmatrix} = \begin{pmatrix}<br />1\\<br />1\\<br />2<br />\end{pmatrix}+s\begin{pmatrix}<br />1\\<br />0\\<br />1<br />\end{pmatrix}+t\begin{pmatrix}<br />0\\<br />1\\<br />1<br />\end{pmatrix}\quad s,t\in\mathbb{R}\]](/tex-image/23e9b2ca84cbd331bff7df46504b2b44.png) ![TEX: \[ L: \begin{pmatrix}<br />x\\<br />y\\<br />z<br />\end{pmatrix} = \begin{pmatrix}<br />1\\<br />0\\<br />2<br />\end{pmatrix}+\lambda\begin{pmatrix}<br />a\\<br />1\\<br />c<br />\end{pmatrix}\]](/tex-image/3ed9e38b79919455904e37c7e7524a9b.png) donde a y c son parámetros. Encuentre condiciones sobre los parámetros  y y  para que para que 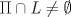 . ¿Existe algún caso en que . ¿Existe algún caso en que 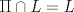 ? ?Tiempo: 2,5 horas Soluciones: P1. P2. P3. Mensaje modificado por TribalJazz2 el Jul 21 2014, 11:19 AM -------------------- |
|
|
|
 Oct 26 2013, 07:28 PM Oct 26 2013, 07:28 PM
Publicado:
#13
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 195 Registrado: 26-December 10 Desde: Nuncajamás. Miembro Nº: 82.286 Nacionalidad:  Sexo:  |
Pendiente. (Se agradecen aportes.)
Mensaje modificado por TribalJazz2 el Oct 27 2013, 03:51 PM -------------------- |
|
|
|
 Oct 26 2013, 07:35 PM Oct 26 2013, 07:35 PM
Publicado:
#14
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 195 Registrado: 26-December 10 Desde: Nuncajamás. Miembro Nº: 82.286 Nacionalidad:  Sexo:  |
Ingeniería Matemática Facultad de Ciencias Físicas y Matemáticas Álgebra Lineal 13-2 Control 1 Semestre Primavera 2013
![TEX: \[\begin{pmatrix}<br />3 & 0 & 2\\<br />\frac{1}{2} & b & 1\\<br />a & 2b & 2a<br />\end{pmatrix}<br />\begin{pmatrix}<br />x\\<br />y\\<br />z<br />\end{pmatrix}=\begin{pmatrix}<br />1\\<br />\frac{1}{2}\\<br />b<br />\end{pmatrix}\]](/tex-image/36bffa79a50a997999f24f4c737d49b5.png) donde 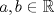 son parámetros. Determine los valores de son parámetros. Determine los valores de  y y  tales que el sistema: tenga solución única, tenga infinitas soluciones, no tenga soluciones. tales que el sistema: tenga solución única, tenga infinitas soluciones, no tenga soluciones.b)
![TEX: <br />\[A=\begin{pmatrix}<br />1 & 0 & 0 & 0\\<br />1 & 1 & 0 & 0\\<br />1 & 1 & 1 & 0\\<br />1 & 1 & 1 & 1<br />\end{pmatrix}\in\mathcal{M}_{4\times 4}\]](/tex-image/273888ecf6ba694f1dada7265f981407.png) Calcule, escalonando, 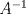 . .b2) (1,0 pto.) Sea 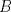 la matriz análoga a la matriz análoga a 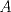 pero de tamaño pero de tamaño 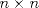 , es decir , es decir![TEX: \[B=\begin{pmatrix}<br />1 & 0 & \cdots & 0\\<br />1 & \ddots & \ddots & \vdots\\<br />\vdots & \ddots & \ddots & 0\\<br />1 & \cdots & 1 & 1<br />\end{pmatrix}\text{ \ \ o bien } b_{ij}=\begin{cases}<br />1 & \text{si }i\geq j\\<br />0 & \text{si no}<br />\end{cases}\]](/tex-image/a81bfd6f757ef86bea54249afc4a66e3.png) Justifique que 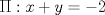 . .
 . Es decir, determinar la intersección de las rectas que pasan por los puntos F y A, F y B, F y C con el plano . Es decir, determinar la intersección de las rectas que pasan por los puntos F y A, F y B, F y C con el plano  . .b) (1,0 pto) Encontrar la ecuación vectorial de la recta que pasa por A' y B'. c) (2,0 ptos.) Determinar la recta de intersección del plano  con el plano que contiene a los puntos A, B y C. con el plano que contiene a los puntos A, B y C.
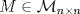 se llama idempotente si M2=M. Si se llama idempotente si M2=M. Si 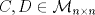 son tales que C=CD y D=DC, demuestre que C y D son idempotentes. son tales que C=CD y D=DC, demuestre que C y D son idempotentes.b) (2,0 ptos.) Demuestre que si A, B y (A+B-1) son matries invertibles, entonces (A-1+B) también es invertible y su inversa es A(A+B-1)-1B-1. c) (2,0 ptos.) Considere las matrices 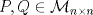 tales que P2=P y Q=I-P. Demuestre que Q3=Q. Si P es iinvertible, use las condiciones dadas en este punto para probar que P=I y Q=0. tales que P2=P y Q=I-P. Demuestre que Q3=Q. Si P es iinvertible, use las condiciones dadas en este punto para probar que P=I y Q=0.Tiempo: 3 horas Soluciones: P1. P2. P3. Mensaje modificado por TribalJazz2 el Jul 18 2014, 07:34 PM -------------------- |
|
|
|
 Jul 18 2014, 07:49 PM Jul 18 2014, 07:49 PM
Publicado:
#15
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 195 Registrado: 26-December 10 Desde: Nuncajamás. Miembro Nº: 82.286 Nacionalidad:  Sexo:  |
Ingeniería Matemática Facultad de Ciencias Físicas y Matemáticas Álgebra Lineal 14-1 Control 1 Semestre Otoño 2014
 es unitaria si UTU=I. es unitaria si UTU=I.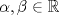 y considere el sistema de ecuaciones y considere el sistema de ecuaciones
 dos matrices que conmutan, es decir que AB=BA. Demuestre que dos matrices que conmutan, es decir que AB=BA. Demuestre que![TEX: \[A=\begin{pmatrix}<br />1&2&3&-1\\<br />-1&1&0&1\\<br />2&1&0&-2\\<br />0&3&3&-3\end{pmatrix}\]](/tex-image/6d9acf3c7cdd10fe43062baf06acf922.png) Calcule mediante el método de Gauss, la inversa de A. Tiempo: 3 horas Soluciones: P1. P2. P3. Mensaje modificado por TribalJazz2 el Jul 21 2014, 11:36 AM -------------------- |
|
|
|
 Oct 17 2014, 10:15 AM Oct 17 2014, 10:15 AM
Publicado:
#16
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 195 Registrado: 26-December 10 Desde: Nuncajamás. Miembro Nº: 82.286 Nacionalidad:  Sexo:  |
Ingeniería Matemática Facultad de Ciencias Físicas y Matemáticas Álgebra Lineal 14-2 Control 1 Semestre Primavera 2014
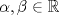 y considere el siguiente sistema de lineal y considere el siguiente sistema de lineal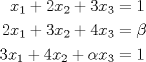
 y y 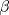 para los cuales el sistema tiene solución única, no tiene solución, tiene infinitas soluciones y en este último caso, determine dichas soluciones. para los cuales el sistema tiene solución única, no tiene solución, tiene infinitas soluciones y en este último caso, determine dichas soluciones.b) (1,5 ptos.) Para 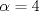 encuentre la inversa de la matriz del sistema. encuentre la inversa de la matriz del sistema.
![TEX: \[L_1 = (x,y,z) = (2,0,1)+t(3,2,1), \quad t\in\mathbb{R}\]](/tex-image/0f0bfe0fc5d5aadbe4df102e267361a1.png) y ![TEX: \[L_2 = \left\{(x,y,z)\in\mathbb{R}^3\mid \frac{x}{-1}=\frac{y-2}{1}=\frac{z}{-2}\right\}\]](/tex-image/2e4f2623d9455f10d3e62c3fdcbf8833.png)
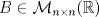 , tal que B es invertible y B5-B=0. Calcule B-1. , tal que B es invertible y B5-B=0. Calcule B-1.b) (2,0 ptos.) Sea ![TEX: \[A=(1)_{n\times n}=\begin{pmatrix}1 & \dots & 1\\ \vdots & \ddots & \vdots\\ 1 & \dots & 1\end{pmatrix}\in\mathcal{M}_{n\times n}(\mathbb{R}),n>1\]](/tex-image/90f342a557ef1e576673523281b6d7d4.png) Encuentre 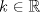 tal que (I-A)-1=I+kA. tal que (I-A)-1=I+kA.c) (2,0 ptos.) Sean 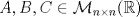 , tales que A y B son simétricas y A es invertible. Demuestre que Ct(A-1+B)C es simétrica. , tales que A y B son simétricas y A es invertible. Demuestre que Ct(A-1+B)C es simétrica.Tiempo: 3 horas Soluciones: P1. P2. P3. -------------------- |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 15th July 2025 - 02:32 PM |





![TEX: \[\forall u,v \in \mathbb{R}^n, (u\cdot v)^2\leq\|u\|^2\|v\|^2\]](/tex-image/283098445a030208c5fa871145b851c6.png) Cauchy y Schwarz... Y Bun...
Cauchy y Schwarz... Y Bun...
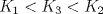 ???[/juice]
???[/juice]


 es invertible
es invertible dada por
dada por![TEX: \[c_{ij}=\begin{cases}<br />1 & \text{si } i=j\\<br />-1 & \text{si } i=j+1\\<br />0 & \text{si no}<br />\end{cases}\]](/tex-image/4ed145530fa0f020cbd570d0a7cf229d.png)
 .
.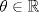 considere la matriz
considere la matriz![TEX: \[G(\theta)=\begin{pmatrix}<br />\cos(\theta)&-\sin(\theta)\\<br />\sin(\theta)&\cos(\theta)<br />\end{pmatrix}\]](/tex-image/49a54f0215aa86bbae31349a4c9ed011.png)
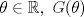 es unitaria y demuestre que
es unitaria y demuestre que  .
. 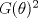 y luego deduzcauna fórmla para
y luego deduzcauna fórmla para 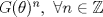 (es decir, incluyendo potencias de inversas).
(es decir, incluyendo potencias de inversas). y
y 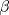 para que el sistema sea compatible (tenga solución).
para que el sistema sea compatible (tenga solución). y
y 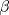 , señalando condiciones sobre estos para que el sistema tenga solución única, y para que tenga infinitas soluciones. Justifique claramente cada caso.
, señalando condiciones sobre estos para que el sistema tenga solución única, y para que tenga infinitas soluciones. Justifique claramente cada caso. , AnB=BAn.
, AnB=BAn. que contiene al punto Q y a la recta L1.
que contiene al punto Q y a la recta L1.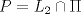 .
.![TEX: \[V=\left\{A\in\mathcal{M}_{3\times 3}(\mathbb{R})\mid A=\begin{pmatrix}a&b&b\\b&a&b\\b&b&a\end{pmatrix}, a,b\in\mathbb{R}\right\}\]](/tex-image/5dc10102da8a9f1806c3ce680e501139.png)
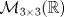 .
.![TEX: $W=\{q(x)\in\mathcal{P}_4[x]\mid q(x)=a_0+a_1 x+a_0x^2+a_1x^3+a_0x^4, a_0,a_1\in\mathbb{R}\}$](/tex-image/d59c8a61f05e05320c1105f6c421f4b5.png) . Pruebe que W es subespacio vectorial de
. Pruebe que W es subespacio vectorial de ![TEX: $\mathcal{P}_4[x]$](/tex-image/a4edee7dddaf476c8a3bbaf4741a0aa3.png) (Polinomios con coeficientes reales de grado menor o igual a cuatro).
(Polinomios con coeficientes reales de grado menor o igual a cuatro).