Suma / S.Ramanujan - O.Schlömilch

Suma / S.Ramanujan - O.Schlömilch

Opciones

master_c	Mar 15 2013, 10:23 PM Publicado: #1
Invitado	Sean 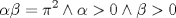$TEX: $$\alpha \beta = \pi ^2 \wedge \alpha > 0 \wedge \beta > 0$$$ Probar que 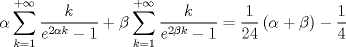$TEX: $$<br />\alpha \sum\limits_{k = 1}^{ + \infty } {\frac{k}<br />{{e^{2\alpha k} - 1}}} + \beta \sum\limits_{k = 1}^{ + \infty } {\frac{k}<br />{{e^{2\beta k} - 1}}} = \frac{1}<br />{{24}}\left( {\alpha + \beta } \right) - \frac{1}<br />{4}<br />$$$

black-lotus Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 8 2020, 12:16 PM Publicado: #2
Matemático Grupo: Usuario FMAT Mensajes: 34 Registrado: 14-May 18 Miembro Nº: 157.192	Hint, esto se puede demostrar usando la función eta

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Fecha y Hora actual: 22nd October 2025 - 01:10 AM