Dominio del operador adjunto

Foro fmat.cl > Programa Enseñanza Universitaria > Consultas > Banco de Problemas Resueltos > Análisis

Dominio del operador adjunto

Opciones

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 29 2011, 10:12 PM Publicado: #1
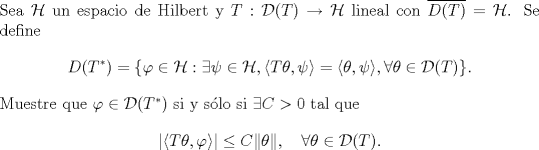
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent Sea $\mathcal H$ un espacio de Hilbert y $T:\mathcal D(T)\to\mathcal H$ lineal con $\overline{D(T)}=\mathcal H$. Se define <br />$$D(T^)=\{\varphi\in\mathcal H:\exists\psi\in\mathcal H,\langle T\theta,\psi\rangle =\langle \theta,\psi\rangle,\forall \theta\in\mathcal D(T)\}.$$<br />Muestre que $\varphi\in\mathcal D(T^)$ si y sólo si $\exists C>0$ tal que $$\left\|\langle T\theta,\varphi\rangle\right\|\le C\\|\theta\\|,\quad \forall \theta\in\mathcal D(T).$$<br />$ -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

nmg1302 Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 16 2012, 11:46 AM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 832 Registrado: 11-September 07 Desde: París, Francia Miembro Nº: 10.056 Nacionalidad: Sexo:	$TEX: <br />Creo que la definición debiese ser así<br />$$\mathcal D(T^)=\{\varphi\in\mathcal H:\exists\psi\in\mathcal H,\langle T\theta,\varphi\rangle =\langle \theta,\psi\rangle,\forall \theta\in\mathcal D(T)\}.$$<br />Si es el caso:\\<br />Si $\varphi \in \mathcal D(T^)$, entonces existe $\psi\in\mathcal H$ tal que $\|\langle T\theta,\varphi\rangle\| =\|\langle \theta,\psi\rangle\|\leq \\|\phi\\| \\| \theta\\|,\forall \theta\in\mathcal D(T)$\\<br />Recíprocamente, si existe $C>0$ que cumple eso, tenemos que $\ell(\cdot ):=\langle \varphi, T\cdot \rangle$ es una funcion lineal continua en $\mathcal D(T)$, y como este ultimo es denso, podemos extender $\ell$ continuamente a $\mathcal H$. Por Riesz, existe $\psi \in \mathcal H$ tal que $\ell(x)=\langle \psi, x\rangle , \forall x \in \mathcal H$, en particular $\langle T\theta,\varphi\rangle=\langle \theta,\psi\rangle,\forall \theta\in\mathcal D(T)$\\<br />$\therefore \varphi\in \mathcal D(T^)$<br />$ Mensaje modificado por nmg1302* el Feb 16 2012, 12:27 PM

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 16 2012, 12:29 PM Publicado: #3
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(nmg1302 @ Feb 16 2012, 01:46 PM) $TEX: <br />Creo que la definición debiese ser así<br />$$\mathcal D(T^)=\{\varphi\in\mathcal H:\exists\psi\in\mathcal H,\langle T\theta,\varphi\rangle =\langle \theta,\psi\rangle,\forall \theta\in\mathcal D(T)\}.$$<br />Si es el caso:\\<br />Si $\varphi \in \mathcal H$, entonces existe $\psi\in\mathcal H$ tal que $\|\langle T\theta,\varphi\rangle\| =\|\langle \theta,\psi\rangle\|\leq \\|\phi\\| \\| \theta\\|,\forall \theta\in\mathcal D(T)$\\<br />Recíprocamente, si existe $C>0$ que cumple eso, tenemos que $\ell(\cdot ):=\langle \varphi, T\cdot \rangle$ es una funcion lineal continua en $\mathcal D(T)$, y como este ultimo es denso, podemos extender $\ell$ continuamente a $\mathcal H$. Por Riesz, existe $\psi \in \mathcal H$ tal que $\ell(x)=\langle \psi, x\rangle , \forall x \in \mathcal H$, en particular $\langle T\theta,\varphi\rangle=\langle \theta,\psi\rangle,\forall \theta\in\mathcal D(T)$\\<br />$\therefore \varphi\in \mathcal D(T^)$<br />$ Sí, me pifié cuando tipié la definición pero se entiende que era esa jaja. Tu prueba está bien, salvo la parte en la que extiendes 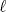$TEX: $\ell$$ . 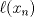Digo esto porque no es directo que si $TEX: $x_n\to x$$ , el límite de $TEX: $\ell(x_n)$$ exista. -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

Krebante Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 16 2012, 12:42 PM Publicado: #4
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 618 Registrado: 8-June 08 Desde: Paris Miembro Nº: 26.525 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Abu-Khalil @ Feb 16 2012, 12:29 PM) Sí, me pifié cuando tipié la definición pero se entiende que era esa jaja. Tu prueba está bien, salvo la parte en la que extiendes 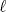$TEX: $\ell$$ . 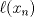Digo esto porque no es directo que si $TEX: $x_n\to x$$ , el límite de $TEX: $\ell(x_n)$$ exista. Supongo que usó el teorema que dice eso XD. -------------------- ¡Por más representación, vota Riesz!

nmg1302 Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 16 2012, 12:45 PM Publicado: #5
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 832 Registrado: 11-September 07 Desde: París, Francia Miembro Nº: 10.056 Nacionalidad: Sexo:	puede ser por HB o por que es una función uniformemente continua en un denso de un espacio completo o por esto

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 16 2012, 12:49 PM Publicado: #6
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	De acuerdo, cualquiera sirve. Sólo era para dejarlo claro a cualquiera que agarre el post y tenga toda la materia tan fresca -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

« Posterior · Análisis · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 05:21 PM