|
|
|
|
|
|
|
  |
 Oct 8 2011, 08:26 AM Oct 8 2011, 08:26 AM
Publicado:
#1
|
|
|
Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 16 Registrado: 24-August 11 Miembro Nº: 93.351 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Suponga que A, B y D son tres sucesos tales que A y B son excluyentes, que A y D son independientes
y que B y D son independientes. Suponga por otro lado que 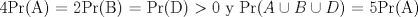 . Determine la probabilidad de A. . Determine la probabilidad de A.Pr= probabilidad Mensaje modificado por Gianni128 el Oct 10 2011, 03:30 PM |
|
|
|
 Oct 10 2011, 11:55 AM Oct 10 2011, 11:55 AM
Publicado:
#2
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Como
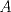 y y 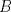 son excluyentes, tienes que son excluyentes, tienes que 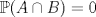 . Luego, . Luego,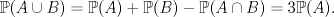 Así, como 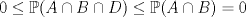 , tienes que , tienes que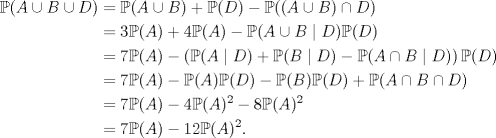 Finalmente, tienes que 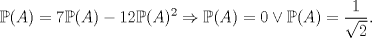
-------------------- |
|
|
|
 Oct 10 2011, 03:29 PM Oct 10 2011, 03:29 PM
Publicado:
#3
|
|
|
Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 16 Registrado: 24-August 11 Miembro Nº: 93.351 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
muchas Gracias Mensaje modificado por Gianni128 el Oct 10 2011, 03:30 PM |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 24th October 2025 - 08:00 AM |











![TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]](./tex/e0e88dba678ca3ead120b088f41191b2.png)

