 Facilito :D , Binomio + PG |
|
|
|
|
|
|
Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Álgebra > Progresiones: Aritmética y Geométrica  |
 Jul 3 2011, 11:28 PM
Publicado:
#1
|
|
 Dios Matemático  Grupo: Usuario FMAT Mensajes: 396 Registrado: 26-October 08 Desde: Quilpue Miembro Nº: 37.052 Nacionalidad:  Universidad:  Sexo:   |
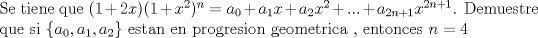 Lo encontré entretenido este problema que me salio en una ayudantia 
-------------------- Estudiante de Segundo Año
Ingeniería Civil en Matemáticas Universidad Técnica Federico Santa María "Dudar de todo o creerlo todo son dos opciones igualmente cómodas, pues tanto una como otra nos eximen de reflexionar" Henri Poincaré |
 |
 
|
|
Jul 3 2011, 11:48 PM
Publicado:
#2
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 2.039 Registrado: 4-October 09 Desde: Valparaíso Miembro Nº: 59.794 Nacionalidad: Colegio/Liceo:  Universidad: Sexo: |
Pucha el titulo es el hintazo D:
--------------------   |
|
|
|
|
Jul 4 2011, 04:36 PM
Publicado:
#3
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 382 Registrado: 2-January 09 Miembro Nº: 41.601 Nacionalidad: Universidad:  Sexo: |
![TEX: \[<br />\begin{gathered}<br /> {\text{Usando el teorema del binomio se tiene que }} \hfill \\<br /> \left( {1 + x^2 } \right)^n = \sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right)x^{2k} } \hfill \\<br /> {\text{Y multiplicando se obtiene }} \hfill \\<br /> \left( {1 + 2x} \right)\left( {1 + x^2 } \right)^n = (1 + 2x)\sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right)x^{2k} } = \sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right)x^{2k} } + 2\sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right)x^{2k + 1} } \hfill \\<br /> {\text{Entonces notamos que }} \hfill \\<br /> a_0 = \left( \begin{gathered}<br /> n \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right) \hfill \\<br /> a_1 = 2\left( \begin{gathered}<br /> n \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right) \hfill \\<br /> a_2 = \left( \begin{gathered}<br /> n \hfill \\<br /> 1 \hfill \\ <br />\end{gathered} \right) \hfill \\<br /> {\text{Como dichos elementos estan en PG}}{\text{, se cumple que }} \hfill \\<br /> \frac{{a_1 }}<br />{{a_0 }} = \frac{{a_2 }}<br />{{a_1 }} \Rightarrow \frac{{2\left( \begin{gathered}<br /> n \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right)}}<br />{{\left( \begin{gathered}<br /> n \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right)}} = \frac{{\left( \begin{gathered}<br /> n \hfill \\<br /> 1 \hfill \\ <br />\end{gathered} \right)}}<br />{{2\left( \begin{gathered}<br /> n \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right)}} \Rightarrow 4 = \frac{{n!}}<br />{{(n - 1)!}} = \frac{{(n - 1)!n}}<br />{{(n - 1)!}} = n \hfill \\<br /> {\text{Asi}}{\text{, }}n = 4. \hfill \\<br /> \hfill \\ <br />\end{gathered} <br />\]<br />](/tex-image/25b0d992535a0cf34b9e4d8c551e1603.png)
|
|
|
|
 Nov 7 2013, 08:12 PM
Publicado:
#4
|
|
|
Principiante Matemático Grupo: Usuario FMAT Mensajes: 3 Registrado: 28-October 13 Miembro Nº: 123.836 Nacionalidad: Colegio/Liceo:  Universidad:  Sexo: |
CITA(inakamono @ Jul 4 2011, 04:36 PM)  Sé qué el post es del año de la Cocoa pero tengo una duda, no veo de donde salen los términos a0, a1 y a2 
|
|
|
|
|
Nov 7 2013, 08:26 PM
Publicado:
#5
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 427 Registrado: 5-October 10 Miembro Nº: 78.264 Nacionalidad: Universidad:  Sexo: |
CITA(Diego chamaca @ Nov 7 2013, 08:12 PM) Sé qué el post es del año de la Cocoa pero tengo una duda, no veo de donde salen los términos a0, a1 y a2 del enunciado a0 es el término independiente, a1 el que acompaña a x y a2 el que acompaña a x^2. por ejemplo antes del a0 = (n 0) hay 2 sumatorias entonces en la primera sumatoria (el que tiene x^2k)hay algún k, para que de un termino independiente? si, para k=0, y para la otra sumatoria(el que tiene x^2k+1)? no existe k entero que forme un termino independiente entonces a0 = al coeficiente de la primera sumatoria cuando k = 0, que seria (n k) = (n 0) espero se entienda xd 
Mensaje modificado por Coto-kun el Nov 7 2013, 08:27 PM -------------------- |
|
|
|
|
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 23rd November 2024 - 05:52 PM |
