|
|
|
|
|
|
|
  |
| Gastón Burrull |
 Jul 6 2011, 08:12 PM Jul 6 2011, 08:12 PM
Publicado:
#91
|
|
Invitado |
Para que se motiven, daré un problema muy similar al anterior como complemento. Espero que te motives tochalo.
Problema. Evalúe para  la integral dada por la integral dada por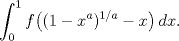 Donde 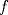 es una función par. es una función par.
|
|
|
|
 Jul 7 2011, 03:31 PM Jul 7 2011, 03:31 PM
Publicado:
#92
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 426 Registrado: 13-January 08 Desde: desde la pieza de tu hermana Miembro Nº: 14.612 Nacionalidad:  Colegio/Liceo:  Sexo:  |
Tendré fe de que esta bien, pues no sí si tu procedimiento es correcto o no, pues tengo nulo conocimiento de funciones especiales. En todo caso la solución podía salir en pocos pasos de manera muy elemental Hola Gastón Sin ánimos de discusión, no le veo mucho sentido dar un problema por resuelto bajo ese argumento. Por otro lado, las “funciones especiales” son pan de cada día en el foro, si ataqué el problema de esta forma es porque lo he aprendido de otros usuarios como danielomalmsteen, crush, abu, etc. Ese “tendré fe” es mejor reemplazarlo por un “le preguntaré a otro usuario”. De todas formas sería valioso que postearas la solución que tienes del problema, así podemos tener más ideas para atacar tu otro propuesto Saludos |
|
|
|
| Gastón Burrull |
 Jul 7 2011, 04:58 PM Jul 7 2011, 04:58 PM
Publicado:
#93
|
|
Invitado |
ok, en un rato mas pongo la solucion, en todo caso te toca proponer si quieres
|
|
|
|
 Jul 7 2011, 05:50 PM Jul 7 2011, 05:50 PM
Publicado:
#94
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 426 Registrado: 13-January 08 Desde: desde la pieza de tu hermana Miembro Nº: 14.612 Nacionalidad:  Colegio/Liceo:  Sexo:  |
|
|
|
|
 Jul 7 2011, 06:37 PM Jul 7 2011, 06:37 PM
Publicado:
#95
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad:  Sexo:  |
Bien, uno facilito:
Halle 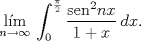
|
|
|
|
 Jul 10 2011, 08:57 PM Jul 10 2011, 08:57 PM
Publicado:
#96
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad:  Sexo:  |
Iba a hacer esto ayer pero se me olvidó.
Primero escribamos 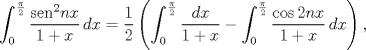 (1) para la integral restante, la hacemos por partes y queda (1) para la integral restante, la hacemos por partes y queda 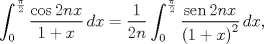 luego luego 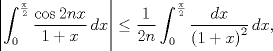 luego la integral del sustraendo de (1) tiende a cero para luego la integral del sustraendo de (1) tiende a cero para 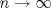 y el límite es simplemente y el límite es simplemente 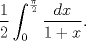 Dejo libre para que cualquiera proponga, ojalá se motiven. |
|
|
|
 Jul 10 2011, 09:17 PM Jul 10 2011, 09:17 PM
Publicado:
#97
|
|
 Dios Matemático Supremo Grupo: Team Ensayos FMAT Mensajes: 1.294 Registrado: 16-March 09 Desde: ancud Miembro Nº: 45.100 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
propongo una facilita haber si alguien "joven" se motiva xDD
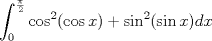 saludos --------------------   Estudiante de 4° año ing civil mec utfsm |
|
|
|
 Jul 10 2011, 10:41 PM Jul 10 2011, 10:41 PM
Publicado:
#98
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[\begin{gathered}<br /> I = \int\limits_0^{\frac{\pi }{2}} {\left[ {{{\cos }^2}\left( {\cos x} \right) + {{\sin }^2}\left( {\sin x} \right)} \right]dx} \hfill \\<br /> {\text{Por paridad:}} \hfill \\<br /> I = \int\limits_{ - \frac{\pi }{2}}^0 {\left[ {{{\cos }^2}\left( {\cos x} \right) + {{\sin }^2}\left( {\sin x} \right)} \right]dx} \hfill \\<br /> sea\;t = x + \frac{\pi }{2} \hfill \\<br /> I = \int\limits_0^{\frac{\pi }{2}} {\left[ {{{\cos }^2}\left( {\sin t} \right) + {{\sin }^2}\left( {\cos t} \right)} \right]dt} \hfill \\<br /> {\text{sumanos con el primer termino y tenemos que}} \hfill \\<br /> I = \frac{\pi }{2} \hfill \\ <br />\end{gathered} \]](/tex-image/b4fbebf66b09cf98debbbdee0a54e3eb.png) Y nosé que proponer. Corregí y aprendi a corregir las imagenes xD Me faltó agregar que alguien mas propusiera algo. Mensaje modificado por Crash! el Jul 10 2011, 10:59 PM --------------------  Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
| Gastón Burrull |
 Jul 11 2011, 12:24 AM Jul 11 2011, 12:24 AM
Publicado:
#99
|
|
Invitado |
Pondré un nivel intermedio entre la primera que propuse y la última, a ver si se animan.
Problema. Evalúe para  la integral dada por la integral dada por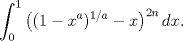 Donde 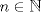 . .
|
|
|
|
 Jul 11 2011, 01:56 AM Jul 11 2011, 01:56 AM
Publicado:
#100
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[\begin{gathered}<br /> {\text{sea }}f{\text{ funcion par}}{\text{.}} \hfill \\<br /> sea\;\alpha > 0 \hfill \\<br /> {I_f} = \int\limits_0^1 {f\left( {{{\left[ {1 - {x^\alpha }} \right]}^{\frac{1}{\alpha }}} - x} \right)dx} \hfill \\<br /> sea \hfill \\<br /> 1 - {x^\alpha } = {t^\alpha } \Rightarrow x = {\left( {1 - {t^\alpha }} \right)^{\frac{1}{\alpha }}} \Rightarrow dx = - {\left( {1 - {t^\alpha }} \right)^{\frac{1}{\alpha } - 1}}{t^{\alpha - 1}}dt \hfill \\<br /> x = 0 \Rightarrow t = 1 \hfill \\<br /> x = 1 \Rightarrow t = 0 \hfill \\<br /> {I_f} = \int\limits_0^1 {f\left( {t - {{\left[ {1 - {t^\alpha }} \right]}^{\frac{1}{\alpha }}}} \right){{\left( {1 - {t^\alpha }} \right)}^{\frac{1}{\alpha } - 1}}{t^{\alpha - 1}}dt} \hfill \\<br /> {\text{como la variable es muda y f es par lo sumamos a la primera expresion de la integral y}}... \hfill \\<br /> 2{I_f} = \int\limits_0^1 {f\left( {{{\left[ {1 - {x^\alpha }} \right]}^{\frac{1}{\alpha }}} - x} \right)\left( {1 + {{\left( {1 - {x^\alpha }} \right)}^{\frac{1}{\alpha } - 1}}{x^{\alpha - 1}}} \right)dx} \hfill \\<br /> {\text{basta hacer }}z = {\left[ {1 - {x^\alpha }} \right]^{\frac{1}{\alpha }}} - x \Rightarrow - dz = \left( {1 + {{\left( {1 - {x^\alpha }} \right)}^{\frac{1}{\alpha } - 1}}{x^{\alpha - 1}}} \right)dx \hfill \\<br /> \Rightarrow {I_f} = \frac{1}{2}\int\limits_{ - 1}^1 {f\left( z \right)dz} = \int\limits_0^1 {f\left( z \right)dz} \hfill \\<br /> {\text{luego la integral pedida es }} \hfill \\<br /> {I_{{x^{2n}}}} = \int\limits_0^1 {{x^{2n}}dx} = \frac{1}{{2n + 1}} \hfill \\ <br />\end{gathered} \]](/tex-image/4a35583e64564d6d34a71ea1d59c63d0.png) Propuestin: Ojalá se haga sin el método obvio (de residuos) ![TEX: \[\begin{gathered}<br /> {\text{Calcule:}} \hfill \\<br /> \int\limits_0^{2\pi } {\frac{{dx}}{{1 + 2a\cos \left( x \right) + {a^2}}}} \hfill \\ <br />\end{gathered} \]](/tex-image/89633e46aa138b0d2922a503fac9538b.png)
Mensaje modificado por Crash! el Jul 11 2011, 02:11 AM --------------------  Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 10th July 2025 - 06:30 PM |









![TEX: $\sqrt[3]{\dfrac{1}{\cos\left(\frac{\pi}{7}\right)}}-\sqrt[3]{\dfrac{1}{\cos\left(\frac{2\pi}{7}\right)}}+\sqrt[3]{\dfrac{1}{\cos\left(\frac{3\pi}{7}\right)}}=\sqrt[3]{6\sqrt[3]{7}-8}$](./tex/c5b49cf6584e89b12a82db6b29f2cd73.png)