FMAT Integral Bee! - Foro fmat.cl

14 Páginas:

« < 4 5 6 7 8 > »

FMAT Integral Bee!

Opciones

Gastón Burrull	Jun 21 2011, 08:58 PM Publicado: #51
Invitado	CITA(master_c @ Jun 18 2011, 11:02 PM) aun asi quede picao por la serie, alguuuuun dia la podre calcular xd de echo ya sabes como calcular la serie en base a la integral

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 21 2011, 10:26 PM Publicado: #52
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	Te toca proponer ahora, master_c.

master_c	Jun 21 2011, 11:33 PM Publicado: #53
Invitado	CITA(Krizalid @ Jun 21 2011, 11:26 PM) Te toca proponer ahora, master_c. propongo considere 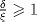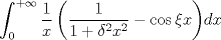$TEX: $\frac{\delta }{\xi } \geqslant 1$$ $TEX: $$<br />\int_0^{ + \infty } {\frac{1}<br />{x}\left( {\frac{1}<br />{{1 + \delta ^2 x^2 }} - \cos \xi x} \right)} dx<br />$$$ cmo estamos en paro he tenido mucho tiempo para investigar cosas, he revisado casi todos los post del banco resuelto y no lo he visto xd espero que no me salgas con un link diciendome que ya ha sido postiado sajsjksk xd slds!

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 25 2011, 12:18 AM Publicado: #54
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	Pon tu solución y coloca otro problema, uno como para seguir enganchando la Integral Bee porque se nos anduvo quedando pegada.

Gastón Burrull	Jun 25 2011, 12:48 AM Publicado: #55
Invitado	La integral propuesta por vivanco vale: 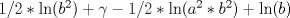$TEX: $1/2\ln(b^2)+\gamma-1/2\ln(a^2b^2)+\ln(b)$$ Donde a y b son esas letras raras con a>b. Ni me pregunten por qué... Mensaje modificado por Gastón Burrull* el Jun 25 2011, 12:49 AM

master_c	Jun 25 2011, 11:12 AM Publicado: #56
Invitado	CITA(Gastón Burrull @ Jun 25 2011, 01:48 AM) La integral propuesta por vivanco vale: $TEX: $1/2\ln(b^2)+\gamma-1/2\ln(a^2b^2)+\ln(b)$$ Donde a y b son esas letras raras con a>b. Ni me pregunten por qué... casi $TEX: $$<br />I = ctr\left( {\frac{1}<br />{{1 + \delta ^2 x^2 }}} \right) - ctr\left( {\cos \left( {\xi x} \right)} \right) = ctr\left( {\frac{1}<br />{{1 + x^2 }}} \right) - \log \delta - \left( {ctr\left( {\cos x} \right) - \log \xi } \right)<br />$$$ $TEX: $$<br /> = \frac{1}<br />{2}crt\left( {\frac{1}<br />{{1 + x}}} \right) - ctr\left( {\cos x} \right) - \log \frac{\delta }<br />{\xi } = \frac{1}<br />{2} \cdot 0 - \left( { - \gamma } \right) - \log \frac{\delta }<br />{\xi } = \gamma - \log \frac{\delta }<br />{\xi }<br />$$$ propongo una hermosa integral putnam 1997* $TEX: $$<br />\int_0^{ + \infty } {\left( {x - \frac{{x^3 }}<br />{2} + \frac{{x^5 }}<br />{{2 \cdot 4}} - \frac{{x^7 }}<br />{{2 \cdot 4 \cdot 6}} + ...} \right)\left( {1 + \frac{{x^2 }}<br />{{2^2 }} + \frac{{x^4 }}<br />{{2^2 \cdot 4^2 }} + \frac{{x^6 }}<br />{{2^2 \cdot 4^2 \cdot 6^2 }} + ...} \right)} dx<br />$$$ Mensaje modificado por master_c el Jun 25 2011, 11:13 AM

Gastón Burrull	Jun 29 2011, 07:50 PM Publicado: #59
Invitado	CITA(master_c @ Jun 29 2011, 09:42 PM) qe paso con la integral bee ? Probablemente nadie conteste porque: "Finalmente, asegúrense de que su problema sea interesante, con resultado elemental " y al igual que con tu problema anterior, con el que no tengo idea que es ese CTR Mensaje modificado por Gastón Burrull el Jun 29 2011, 07:51 PM

walatoo Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 29 2011, 07:50 PM Publicado: #60
Dios Matemático Supremo Grupo: Team Ensayos FMAT Mensajes: 1.294 Registrado: 16-March 09 Desde: ancud Miembro Nº: 45.100 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(master_c @ Jun 25 2011, 01:12 PM) propongo una hermosa integral putnam 1997 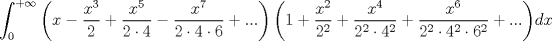$TEX: $$<br />\int_0^{ + \infty } {\left( {x - \frac{{x^3 }}<br />{2} + \frac{{x^5 }}<br />{{2 \cdot 4}} - \frac{{x^7 }}<br />{{2 \cdot 4 \cdot 6}} + ...} \right)\left( {1 + \frac{{x^2 }}<br />{{2^2 }} + \frac{{x^4 }}<br />{{2^2 \cdot 4^2 }} + \frac{{x^6 }}<br />{{2^2 \cdot 4^2 \cdot 6^2 }} + ...} \right)} dx<br />$$$ la primera serie la pude sacar pero está no se me ocurrió xDD 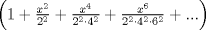$TEX: $\left( {1 + \frac{{x^2 }}<br />{{2^2 }} + \frac{{x^4 }}<br />{{2^2 \cdot 4^2 }} + \frac{{x^6 }}<br />{{2^2 \cdot 4^2 \cdot 6^2 }} + ...} \right)$$ -------------------- Estudiante de 4° año ing civil mec utfsm $TEX: $\sqrt[3]{\dfrac{1}{\cos\left(\frac{\pi}{7}\right)}}-\sqrt[3]{\dfrac{1}{\cos\left(\frac{2\pi}{7}\right)}}+\sqrt[3]{\dfrac{1}{\cos\left(\frac{3\pi}{7}\right)}}=\sqrt[3]{6\sqrt[3]{7}-8}$$

14 Páginas:

« < 4 5 6 7 8 > »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Fecha y Hora actual: 4th April 2025 - 02:22 PM