FMAT Integral Bee! - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Cálculo > Integrales

14 Páginas:

< 1 2 3 4 > »

Closed Topic

Start new topic

FMAT Integral Bee!

Crash! Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 12:36 AM Publicado: #11
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[I = \int\limits_0^1 {\frac{{\ln \left( x \right)}}{{\sqrt x }}dx} = 4\int\limits_0^1 {\frac{{\ln \left( {\sqrt x } \right)}}{{2\sqrt x }}dx} = 4\left( {\sqrt x \ln \left( {\sqrt x } \right) - \sqrt x } \right)_0^1 = - 4\]$ pido 5 minutos xd aqui va $TEX: \[\begin{gathered}<br /> {\text{sea }}g:\mathbb{R} \to \mathbb{R}{\text{ biyectiva}}{\text{, diferenciable tal que }}g\left( 0 \right) = 0. \hfill \\<br /> f:\mathbb{R} \to \left( { - 1,1} \right){\text{ diferenciable y satisfacen que:}} \hfill \\<br /> {\text{g}}\left( x \right) = \int\limits_0^{g\left( x \right)} {{f^2}\left[ {{g^{ - 1}}\left( x \right)} \right]dx} + f\left( x \right) \hfill \\<br /> {\text{Pruebe que:}} \hfill \\<br /> f\left( x \right) = \tanh \left( {g\left( x \right)} \right) \hfill \\ <br />\end{gathered} \]$ Mensaje modificado por Crash! el Jun 17 2011, 12:40 AM -------------------- Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed.

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 01:01 AM Publicado: #12
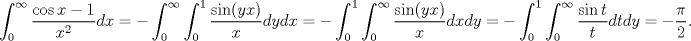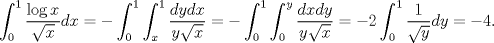Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	También se podían hacer $TEX: $$\int_0^\infty\frac{\cos x-1}{x^2}dx=-\int_0^\infty\int_0^1\frac{\sin(yx)}{x}dydx=-\int_0^1\int_0^\infty\frac{\sin(yx)}{x}dxdy=-\int_0^1\int_0^\infty\frac{\sin t}{t}dtdy=-\frac\pi 2.$$$ $TEX: $$\int_0^1\frac{\log x}{\sqrt x}dx=-\int_0^1\int_x^1\frac{dydx}{y\sqrt x}=-\int_0^1\int_0^y\frac{dxdy}{y\sqrt x}=-2\int_0^1\frac 1{\sqrt y}dy=-4.$$$ -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

Crash! Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 01:20 AM Publicado: #13
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	en verdad me he fijado que a la mayoria de las cosas que se le puede poner apellido para derivar, tambien se pueden expresar como integral doble y ocupar fubini xD -------------------- Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed.

nmg1302 Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 09:50 AM Publicado: #14
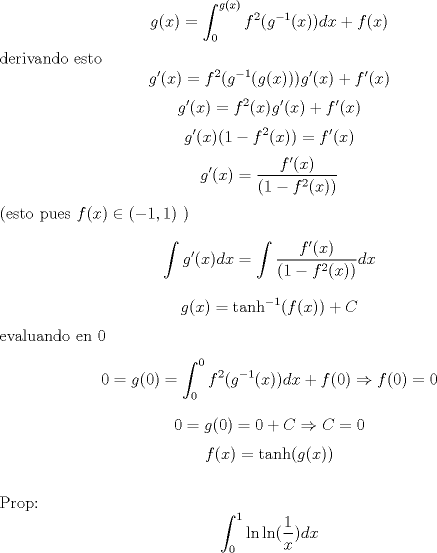
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 832 Registrado: 11-September 07 Desde: París, Francia Miembro Nº: 10.056 Nacionalidad: Sexo:	$TEX: <br />$$g(x)=\int_{0}^{g(x)} f^2(g^{-1}(x)) dx+f(x)$$<br />derivando esto<br />$$g'(x)=f^2(g^{-1}(g(x))) g'(x)+f'(x)$$<br />$$g'(x)=f^2(x) g'(x)+f'(x)$$<br />$$g'(x)(1-f^2(x))=f'(x)$$<br />$$g'(x)=\frac{f'(x)}{(1-f^2(x))}$$<br />(esto pues $f(x)\in (-1,1)$ )<br />$$\int g'(x)dx=\int \frac{f'(x)}{(1-f^2(x))}dx$$<br />$$g(x)=\tanh^{-1}(f(x))+C$$<br />evaluando en 0<br />$$0=g(0)=\int_{0}^{0} f^2(g^{-1}(x)) dx+f(0)\Rightarrow f(0)=0$$<br />$$0=g(0)=0+C \Rightarrow C=0$$<br />$$f(x)=\tanh(g(x))$$<br />\\<br />Prop:<br />$$\int_{0}^{1} \ln \ln(\frac 1 x ) dx$$<br />$ Mensaje modificado por nmg1302 el Jun 17 2011, 10:10 AM

master_c	Jun 17 2011, 02:39 PM Publicado: #15
Invitado	mediante un simple cambio tenemos 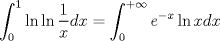$TEX: $$<br />\int_0^1 {\ln \ln \frac{1}<br />{x}} dx = \int_0^{ + \infty } {e^{ - x} \ln xdx} <br />$$<br /><br />$ definamos la funcion y notese que 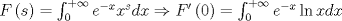$TEX: $F\left( s \right) = \int_0^{ + \infty } {e^{ - x} x^s dx} \Rightarrow F'\left( 0 \right) = \int_0^{ + \infty } {e^{ - x} \ln xdx}$$ ahora 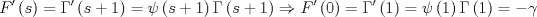$TEX: $$<br />F'\left( s \right) = \Gamma '\left( {s + 1} \right) = \psi \left( {s + 1} \right)\Gamma \left( {s + 1} \right) \Rightarrow F'\left( 0 \right) = \Gamma '\left( 1 \right) = \psi \left( 1 \right)\Gamma \left( 1 \right) = - \gamma <br />$$$ entonces $TEX: $$<br />\int_0^1 {\ln \ln \frac{1}<br />{x}} dx = \int_0^{ + \infty } {e^{ - x} \ln xdx} = - \gamma <br />$$$ tambien la integral es equivalente a esta integral y es directo 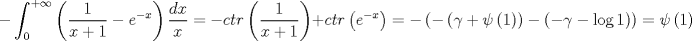$TEX: $$<br /> - \int_0^{ + \infty } {\left( {\frac{1}<br />{{x + 1}} - e^{ - x} } \right)\frac{{dx}}<br />{x}} = - ctr\left( {\frac{1}<br />{{x + 1}}} \right) + ctr\left( {e^{ - x} } \right) = - \left( { - \left( {\gamma + \psi \left( 1 \right)} \right) - \left( { - \gamma - \log 1} \right)} \right) = \psi \left( 1 \right) = - \gamma <br />$$$ $TEX: $$<br />\int_0^1 {\ln \ln \left( {\frac{1}<br />{x}} \right)dx = } - \int_0^{ + \infty } {\left( {\frac{1}<br />{{x + 1}} - e^{ - x} } \right)\frac{{dx}}<br />{x}} = \psi \left( 1 \right) = - \gamma <br />$$$ propongo 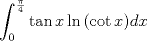$TEX: $$<br />\int_0^{\frac{\pi }<br />{4}} {\tan x\ln \left( {\cot x} \right)} dx<br />$$$ Mensaje modificado por master_c el Jun 17 2011, 03:03 PM

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 07:00 PM Publicado: #16
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	QUOTE(master_c @ Jun 17 2011, 03:39 PM) propongo 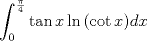$TEX: $$<br />\int_0^{\frac{\pi }<br />{4}} {\tan x\ln \left( {\cot x} \right)} dx<br />$$$ Okay, no es la mejor solución pero omití algunos cálculos: 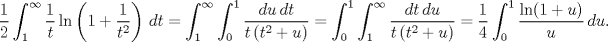$TEX: $$\frac{1}{2}\int_{1}^{\infty }{\frac{1}{t}\ln \left( 1+\frac{1}{{{t}^{2}}} \right)\,dt}=\int_{1}^{\infty }{\int_{0}^{1}{\frac{du\,dt}{t\left( {{t}^{2}}+u \right)}}}=\int_{0}^{1}{\int_{1}^{\infty }{\frac{dt\,du}{t\left( {{t}^{2}}+u \right)}}}=\frac{1}{4}\int_{0}^{1}{\frac{\ln (1+u)}{u}\,du}.$$$ Entonces, $TEX: $$\int_{0}^{\frac{\pi }{4}}{\operatorname{tg}(x)\ln (\cot x)\,dx}=\int_{0}^{\frac{\pi }{4}}{\int_{1}^{\cot x}{\frac{\operatorname{tg}x}{t}\,dt}\,dx}=\int_{1}^{\infty }{\int_{0}^{\arccos t}{\frac{\operatorname{tg}x}{t}\,dx}\,dt}=\frac{1}{2}\int_{1}^{\infty }{\frac{1}{t}\ln \left( 1+\frac{1}{{{t}^{2}}} \right)\,dt},$$$ por tanto el valor de la integral es uno ya calculado acá. Dejo libre la opción de que alguien postee cualquier cosa, porque voy saliendo.

nmg1302 Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 07:34 PM Publicado: #17
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 832 Registrado: 11-September 07 Desde: París, Francia Miembro Nº: 10.056 Nacionalidad: Sexo:	otra forma podria ser $TEX: <br />\noindent con $u=\tan x$<br />$$\int_{0}^{\frac{\pi}{4}} \tan \ln \left (\frac{1}{\tan x}\right ) dx=-\int_{0}^{1} \frac{u \ln u}{1+u^2}du<br />=-\frac 1 2 \int_{0}^{1} (\ln (1+u^2))' \ln u du$$<br />$$=-\frac 1 2 \left [ (\ln(1+u^2)\ln u \mid_0^1 -\int_0^1 \frac{\ln (1+u^2)}{u}du \right ]=\frac 1 2 \int_0^1 \frac{\ln (1+u^2)}{u}du$$<br />$$=\frac 1 4 \int_0^1 \frac{\ln(1+u^2)}{u^2} 2udu=\frac 1 4 \int_0^1 \frac{\ln(1+v)}{v} dv=\frac{\pi^2}{48}$$<br />$

walatoo Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 07:46 PM Publicado: #18
Dios Matemático Supremo Grupo: Team Ensayos FMAT Mensajes: 1.294 Registrado: 16-March 09 Desde: ancud Miembro Nº: 45.100 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	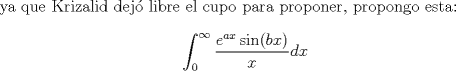$TEX: ya que Krizalid dejó libre el cupo para proponer, propongo esta: $$\displaystyle\int_{0}^{\infty}\dfrac{e^{ax}\sin(bx)}{x}dx$$$ saludos PD: a<0 -------------------- Estudiante de 4° año ing civil mec utfsm $TEX: $\sqrt[3]{\dfrac{1}{\cos\left(\frac{\pi}{7}\right)}}-\sqrt[3]{\dfrac{1}{\cos\left(\frac{2\pi}{7}\right)}}+\sqrt[3]{\dfrac{1}{\cos\left(\frac{3\pi}{7}\right)}}=\sqrt[3]{6\sqrt[3]{7}-8}$$

nmg1302 Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 07:59 PM Publicado: #19
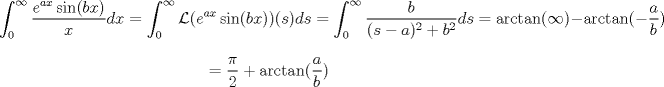
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 832 Registrado: 11-September 07 Desde: París, Francia Miembro Nº: 10.056 Nacionalidad: Sexo:	$TEX: <br />$$\int_{0}^{\infty} \frac{e^{ax}\sin (bx)}{x} dx=\int_{0}^{\infty} \mathcal L(e^{ax}\sin(bx))(s) ds<br />=\int_{0}^{\infty} \frac{b}{(s-a)^2+b^2} ds=\arctan (\infty)- \arctan(-\frac a b)$$<br />$$=\frac \pi 2 +\arctan (\frac a b)$$<br />$ $TEX: <br />Prop:<br /><br />$$\int_{-\infty}^{\infty} \frac{\cos x}{e^x+e^{-x}}dx$$<br />$ Mensaje modificado por nmg1302 el Jun 17 2011, 08:07 PM

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 17 2011, 08:01 PM Publicado: #20
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	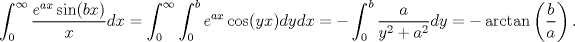$TEX: \begin{equation}\begin{aligned}<br />\int_0^\infty \frac{e^{ax}\sin(bx)}xdx&=\int_0^\infty\int_{0}^b e^{ax}\cos(yx)dydx=-\int_0^b\frac{a}{y^2+a^2}dy=-\arctan\left(\frac ba\right).<br />\end{aligned}\end{equation}$ Me ganaron ): -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

« Posterior · Integrales · Anterior »

14 Páginas:

< 1 2 3 4 > »

Closed Topic

Start new topic

2 usuario(s) está(n) leyendo esta discusión (2 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 04:32 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.