|
|
|
|
|
|
|
  |
| master_c |
 Jun 1 2011, 09:33 PM Jun 1 2011, 09:33 PM
Publicado:
#1
|
|
Invitado |
Si
 comprobar 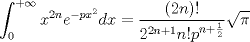 |
|
|
|
 Jun 1 2011, 11:00 PM Jun 1 2011, 11:00 PM
Publicado:
#2
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[\begin{gathered}<br /> I = \int\limits_0^\infty {{x^{2n}}{e^{ - p{x^2}}}dx} \underbrace = _{p{x^2} = t}\frac{1}<br />{{2{p^{n + \frac{1}<br />{2}}}}}\int\limits_0^\infty {{t^{n - \frac{1}<br />{2}}}{e^{ - t}}dt} = \frac{1}<br />{{2{p^{n + \frac{1}<br />{2}}}}}\Gamma \left( {n + \frac{1}<br />{2}} \right) \hfill \\<br /> I = \frac{{\Gamma \left( n \right)\Gamma \left( {n + \frac{1}<br />{2}} \right)}}<br />{{2{p^{n + \frac{1}<br />{2}}}\Gamma \left( n \right)}} = \frac{{\sqrt \pi \Gamma \left( {2n} \right)}}<br />{{{2^{2n}}{p^{n + \frac{1}<br />{2}}}\left( {n - 1} \right)!}} = \frac{{\sqrt \pi \left( {2n - 1} \right)!}}<br />{{{2^{2n}}{p^{n + \frac{1}<br />{2}}}\left( {n - 1} \right)!}} = \frac{{\sqrt \pi \left( {2n} \right)!}}<br />{{{2^{2n + 1}}{p^{n + \frac{1}<br />{2}}}n!}}\begin{array}{*{20}{c}}<br /> {} \\<br /> \square \\<br /><br /> \end{array} \hfill \\ <br />\end{gathered} \]<br />](./tex/e6c8379bd65e46e515095142529e9f9c.png)
--------------------  Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
| master_c |
 Jun 6 2011, 08:10 PM Jun 6 2011, 08:10 PM
Publicado:
#3
|
|
Invitado |
justo en el blanco, podrias exponer otra solucion ?
slds!. |
|
|
|
 Jun 6 2011, 10:11 PM Jun 6 2011, 10:11 PM
Publicado:
#4
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[\begin{gathered}<br /> I\left( p \right) = \int\limits_0^\infty {{e^{ - p{x^2}}}dx} = \frac{1}{{\sqrt p }}\int\limits_0^\infty {{e^{ - {t^2}}}dt} = \frac{{\sqrt \pi }}{{2\sqrt p }} = \sqrt \pi {\left( {\sqrt p } \right)^\prime } \hfill \\<br /> {I^{\left( n \right)}}\left( p \right) = {\left( { - 1} \right)^n}\int\limits_0^\infty {{x^{2n}}{e^{ - p{x^2}}}dx} = \sqrt \pi {\left( {\sqrt p } \right)^{\left( {n + 1} \right)}} = \sqrt \pi \prod\limits_{i = 0}^n {\left( {\frac{1}{2} - i} \right)} {p^{\frac{1}{2} - \left( {n + 1} \right)}} \hfill \\<br /> \Rightarrow I = \int\limits_0^\infty {{x^{2n}}{e^{ - p{x^2}}}dx} = {\left( { - 1} \right)^n}\frac{{\sqrt \pi }}{{{p^{n + \frac{1}{2}}}}}\prod\limits_{i = 0}^n {\left( {\frac{{1 - 2i}}{2}} \right)} \hfill \\<br /> I = {\left( { - 1} \right)^n}\frac{{\sqrt \pi }}{{{2^{n + 1}}{p^{n + \frac{1}{2}}}}}\prod\limits_{i = 0}^n {\left( {1 - 2i} \right)} \hfill \\<br /> I = \frac{{ - \sqrt \pi }}{{{2^{n + 1}}{p^{n + \frac{1}{2}}}}}\prod\limits_{i = 0}^n {\left( {2i - 1} \right)} \hfill \\ <br />\end{gathered} \]](./tex/10538138e7f39621c504ad29b1f7411f.png) ![TEX: \[\begin{gathered}<br /> I = \frac{{ - \sqrt \pi }}{{{2^{n + 1}}{p^{n + \frac{1}{2}}}}}\left( { - 1} \right)\left( 1 \right)\left( 3 \right)\left( 5 \right) \cdots \left( {2n - 3} \right)\left( {2n - 1} \right) \hfill \\<br /> I = \frac{{\sqrt \pi }}{{{2^{n + 1}}{p^{n + \frac{1}{2}}}}}\frac{{\left( 1 \right)\left( 2 \right)\left( 3 \right)\left( 4 \right)\left( 5 \right) \cdots \left( {2n - 2} \right)\left( {2n - 1} \right)\left( {2n} \right)}}{{\left( 2 \right)\left( 4 \right) \cdots \left( {2n - 2} \right)\left( {2n} \right)}} \hfill \\<br /> I = \frac{{\sqrt \pi \left( {2n} \right)!}}{{{2^{n + 1}}{p^{n + \frac{1}{2}}}}}\frac{1}{{{2^n}\left( 1 \right)\left( 2 \right) \cdots \left( {n - 1} \right)\left( n \right)}} \hfill \\<br /> I = \frac{{\sqrt \pi \left( {2n} \right)!}}{{{2^{2n + 1}}{p^{n + \frac{1}{2}}}n!}} \hfill \\ <br />\end{gathered} \]](./tex/b12dbbc4ba30ba15913d3fa3e815a761.png)
--------------------  Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
| master_c |
 Jun 6 2011, 10:34 PM Jun 6 2011, 10:34 PM
Publicado:
#5
|
|
Invitado |
linda solucion, slds
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 22nd October 2025 - 08:40 PM |







