Integrales Impropias de 1er tipo

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Federico Santa María > Mat 022

Integrales Impropias de 1er tipo, Ejemplos.

Opciones

Crasslenton Ver Perfil del Miembro Ver los mensajes de este miembro	Mar 5 2007, 10:36 AM Publicado: #1
Maestro Matemático Grupo: Usuario FMAT Mensajes: 76 Registrado: 7-December 06 Miembro Nº: 3.164 Nacionalidad: Universidad: Sexo:	aqui integrales impropias.... y una ayudantia Archivo(s) Adjunto(s) Integrales_impropias_materia.pdf ( 60.41k ) Número de descargas: 486 Integrales_impropias_eje_res.pdf ( 103.87k ) Número de descargas: 506 Integrales_impropias_eje_prop.pdf ( 66.63k ) Número de descargas: 328 Ayudantia_calculo.pdf ( 79.61k ) Número de descargas: 355 Ayudantia_calculo_sol.pdf ( 104.27k ) Número de descargas: 344 -------------------- La práctica hace al maestro... 100 Sansanos en Fmat...aumentando, Clik aqui

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Mar 25 2007, 04:39 PM Publicado: #2
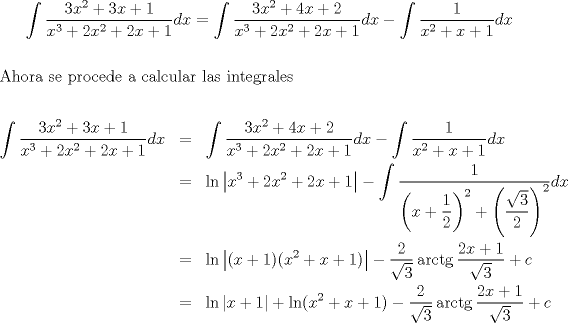Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	Haré el 1ero. de la ayudantía ^^ $TEX: $$\int {\frac{{3x^2 + 3x + 1}}<br />{{x^3 + 2x^2 + 2x + 1}}dx} = \int {\frac{{3x^2 + 4x + 2}}<br />{{x^3 + 2x^2 + 2x + 1}}dx} - \int {\frac{1}<br />{{x^2 + x + 1}}dx}$$\\<br />\noindent Ahora se procede a calcular las integrales<br /><br />\begin{eqnarray}<br /> \int {\frac{{3x^2 + 3x + 1}}<br />{{x^3 + 2x^2 + 2x + 1}}dx} &=& \int {\frac{{3x^2 + 4x + 2}}<br />{{x^3 + 2x^2 + 2x + 1}}dx} - \int {\frac{1}<br />{{x^2 + x + 1}}dx} \hfill \\<br /> &=& \ln \left\| {x^3 + 2x^2 + 2x + 1} \right\| - \int {\frac{1}<br />{{\left( {x + \dfrac{1}<br />{2}} \right)^2 + \left( {\dfrac{{\sqrt 3 }}<br />{2}} \right)^2 }}dx} \hfill \\<br /> &=& \ln \left\| {(x + 1)(x^2 + x + 1)} \right\| - \frac{2}<br />{{\sqrt 3 }}\operatorname{arctg} \frac{{2x + 1}}<br />{{\sqrt 3 }} + c \hfill \\<br /> &=& \ln \left\| {x + 1} \right\| + \ln (x^2 + x + 1) - \frac{2}<br />{{\sqrt 3 }}\operatorname{arctg} \frac{{2x + 1}}<br />{{\sqrt 3 }} + c \hfill \\ <br />\end{eqnarray}$

Makbo Ver Perfil del Miembro Ver los mensajes de este miembro	Mar 25 2007, 07:20 PM Publicado: #3
Dios Matemático Supremo Grupo: Colaborador Silver Mensajes: 743 Registrado: 23-November 05 Desde: Mi Humilde Hogar! Miembro Nº: 394 Nacionalidad: Universidad: Sexo:	CITA(Krizalid @ Mar 25 2007, 03:39 PM) Haré el 1ero. de la ayudantía ^^ esa estaba resuelta xD -------------------- © Ingeniero Civil Metalurgico. $TEX: [<br />psi psi psi .oint {mathfrak{M}alpha dag .mathbb{C}ell } <br />]$ "...La Felicidad es una mariposa que, si la persigues, siempre está justo más alla de tu alcance; Sin embargo, si te sentaras en silencio, podria posarse sobre ti..."

Jeyfix Ver Perfil del Miembro Ver los mensajes de este miembro	May 5 2008, 08:08 PM Publicado: #4
Principiante Matemático Grupo: Usuario FMAT Mensajes: 1 Registrado: 5-May 08 Miembro Nº: 22.231 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	uf.... maravilloso este foro... llegue por google aqui... muchas gracias por los aportes -------------------- _______________________________________________________________________ Canon 10D + sigma 24-70f/2.8EX w/macro + 430EX TTL-2 mi galeria de fotos Mi galeria

Pedro² Ver Perfil del Miembro Ver los mensajes de este miembro	May 5 2008, 10:21 PM Publicado: #5
Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	El primero de los ejercicios propuestos de la ayudantía (no lo vi resuelto xD): $TEX: <br />\[<br />\begin{gathered}<br /> \int_0^\pi {\frac{{dx}}<br />{{a + b\cos x}}} = \frac{\pi }<br />{{\sqrt {a^2 - b^2 } }}\;,{\textsf{ donde }}a > \left\| b \right\| \hfill \\<br /> {\textsf{Primero calculamos la integral indefinida:}} \hfill \\<br /> I = \int {\frac{{dx}}<br />{{a + b\cos x}}} \hfill \\<br /> {\textsf{Mediante la sustitución }}u = \tan \left( {\frac{x}<br />{2}} \right) \hfill \\<br /> dx = \frac{2}<br />{{1 + u^2 }}\;du,\;\cos x = \frac{{1 - u^2 }}<br />{{1 + u^2 }} \hfill \\<br /> I = 2\int {\frac{{du}}<br />{{a + au^2 + b - bu^2 }} = } 2\int {\frac{{du}}<br />{{a + b + u^2 (a - b)}} = } 2\int {\frac{{du}}<br />{{\left( {\sqrt {a + b} } \right)^2 + \left( {u\sqrt {a - b} } \right)^2 }}} \hfill \\<br /> I = \frac{2}<br />{{\sqrt {a + b} \cdot \sqrt {a - b} }}\arctan \left( {\frac{{u\sqrt {a - b} }}<br />{{\sqrt {a + b} }}} \right) + C \hfill \\<br /> \int {\frac{{dx}}<br />{{a + b\cos x}}} = \frac{2}<br />{{\sqrt {a^2 - b^2 } }}\arctan \left( {\tan \left( {\frac{x}<br />{2}} \right)\sqrt {\frac{{a - b}}<br />{{a + b}}} } \right) + C \hfill \\<br /> {\textsf{Evaluando la integral definida:}} \hfill \\<br /> \int_0^\pi {\frac{{dx}}<br />{{a + b\cos x}}} = \left. {\frac{2}<br />{{\sqrt {a^2 - b^2 } }}\arctan \left( {\tan \left( {\frac{x}<br />{2}} \right)\sqrt {\frac{{a - b}}<br />{{a + b}}} } \right)} \right]_0^\pi = \frac{2}<br />{{\sqrt {a^2 - b^2 } }} \cdot \frac{\pi }<br />{2} = \frac{\pi }<br />{{\sqrt {a^2 - b^2 } }} \hfill \\<br /> \hfill \\<br /> {\textsf{Saludos}}{\text{.}} \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática* - Mechón 2009* "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy

John Fullenkamp Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 22 2008, 07:21 PM Publicado: #6
Principiante Matemático Grupo: Usuario FMAT Mensajes: 13 Registrado: 17-April 07 Desde: X Región Miembro Nº: 5.268 Nacionalidad: Universidad: Sexo:	Se Agradece----

homero_492 Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 14 2008, 03:27 PM Publicado: #7
Principiante Matemático Grupo: Usuario FMAT Mensajes: 3 Registrado: 7-June 08 Miembro Nº: 26.320 Nacionalidad: Sexo:	excelente estan muy wenos gracias mestro

nezpatrik Ver Perfil del Miembro Ver los mensajes de este miembro	May 30 2017, 10:52 PM Publicado: #8
Principiante Matemático Grupo: Usuario FMAT Mensajes: 3 Registrado: 15-May 16 Miembro Nº: 145.638 Nacionalidad: Sexo:	excelente aporte, gracias

« Posterior · Mat 022 · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 06:41 PM