C1 Calculo I - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Católica > Cálculo I

Reply to this topic

Start new topic

C1 Calculo I, 1S - 2011

Kura Ver Perfil del Miembro Ver los mensajes de este miembro	Mar 29 2011, 08:50 PM Publicado: #1
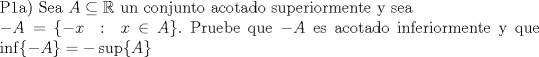Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.918 Registrado: 14-May 08 Desde: The Tower of God Miembro Nº: 23.100 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Animense a hacerlo. $TEX: $\\$P1a) Sea $A \subseteq \mathbb{R}$ un conjunto acotado superiormente y sea \\ $-A = \{-x\ :\ x \in A\}.$ Pruebe que $-A$ es acotado inferiormente y que $\inf\{-A\} = - \sup\{A\}$$ $TEX: $\\$P1b) Sea $A \subseteq \mathbb{R}$ un conjunto acotado superiormente y sea \\ $cA = \{c x\ : \ x\in A \}.$ Pruebe que $cA$ es acotado superiormente y que $\sup\{cA\} = c\sup\{A\}$$ $TEX: $\\$P2) Sea $a>0$ y definimos la siguiente sucesion $$x_1= 1 \qquad , \qquad x_{n+1} = \frac{1}{2} \left(x_n + \frac{a}{x_n} \right)$$ Demuestre que la sucesión es decreciente, acotada inferiormente y que $$\lim_{n\to \infty} = \sqrt{a}$$$ Saludos -------------------- Far over... the misty mountains cold. To dungeons deep and caverns old. We must away ere break of day. To find our long-forgotten gold. The pines were roaring on the height. The winds were moaning in the night. The fire was red, it flaming spread. The trees like torches blazed with light. Apunte: Sistemas de Ecuaciones Cuadráticas! Apunte: Series de Fourier! Problemas Resueltos: EDO! OMG! Soy el ñoño de eléctrica.

Shine Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 14 2011, 12:56 AM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.255 Registrado: 22-February 08 Miembro Nº: 15.777 Sexo:	Quizá esté escribiendo pescadas (bueno, este control tiene mucho desde que se posteo xD ), pero, la P2 no me da decreciente...

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 14 2011, 08:47 PM Publicado: #3
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: % MathType!MTEF!2!1!+-<br />% feaagaart1ev2aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn<br />% hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr<br />% 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq-Jc9<br />% vqaqpepm0xbba9pwe9Q8fs0-yqaqpepae9pg0FirpepeKkFr0xfr-x<br />% fr-xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGceaqabeaadaqjEa<br />% qaaiaabofacaqGUaGaaeiuaiaabgdaaaaabaGaae4qaiaab+gacaqG<br />% TbGaae4BaiaabccacaqGbbGaaeiiaiaabwgacaqGZbGaaeiiaiaabg<br />% gacaqGJbGaae4BaiaabshacaqGHbGaaeizaiaab+gacaqGGaGaae4C<br />% aiaabwhacaqGWbGaaeyzaiaabkhacaqGPbGaae4BaiaabkhacaqGTb<br />% Gaaeyzaiaab6gacaqG0bGaaeyzaiaabYcacaqGGaGaaeyzaiaab6ga<br />% caqG0bGaae4Baiaab6gacaqGJbGaaeyzaiaabohacaqGGaGaaeiCai<br />% aab+gacaqGYbGaaeiiaiaabggacaqG4bGaaeyAaiaab+gacaqGTbGa<br />% aeyyaiaabccacaqGKbGaaeyzaiaabYgacaqGGaGaae4Caiaabwhaca<br />% qGWbGaaeOCaiaabwgacaqGTbGaae4BaiaabccacaqGZbGaaeyzaiaa<br />% bccacaqG0bGaaeyAaiaabwgacaqGUbGaaeyzaiaabccacaqGZbGaae<br />% yDaiaabccacaqGLbGaaeiEaiaabMgacaqGZbGaaeiDaiaabwgacaqG<br />% UbGaae4yaiaabMgacaqGHbaabaGaaeyEaiaabccacaqGWbGaae4Bai<br />% aabkhacaqGGaGaaeyzaiaab6gacaqGKbGaaeyzaiaabQdacaqGGaGa<br />% ci4CaiaacwhacaGGWbWaaeWaaeaacaWGbbaacaGLOaGaayzkaaGaey<br />% yzImRaamiEaiaabYcacaqGGaGaeyiaIiIaamiEaiabgIGiolaadgea<br />% caqGGaGaaeilaiaabccacaqGSbGaaeyDaiaabwgacaqGNbGaae4Bai<br />% aabQdacaqGGaGaeyOeI0Iaci4CaiaacwhacaGGWbWaaeWaaeaacaWG<br />% bbaacaGLOaGaayzkaaGaeyizImQaeyOeI0IaamiEaiaab6cacaqGGa<br />% Gaaeiuaiaab+gacaqGYbGaaeiiaiaabshacaqGHbGaaeOBaiaabsha<br />% caqGVbGaaeilaiaabccacaqGLbGaaeiBaiaabccacaqGJbGaae4Bai<br />% aab6gacaqGQbGaaeyDaiaab6gacaqG0bGaae4BaiaabccacqGHsisl<br />% caWGbbGaaeiiaiaabwgacaqGZbGaaeiiaaqaaiaabggacaqGJbGaae<br />% 4BaiaabshacaqGHbGaaeizaiaab+gacaqGGaGaaeyAaiaab6gacaqG<br />% MbGaaeyzaiaabkhacaqGPbGaae4BaiaabkhacaqGTbGaaeyzaiaab6<br />% gacaqG0bGaaeyzaiaab6caaeaaaeaacaqGtbGaaeyAaiaabccaciGG<br />% ZbGaaiyDaiaacchadaqadaqaaiaadgeaaiaawIcacaGLPaaacqGH9a<br />% qpcqGHsislcaWGHbGaeyO0H4TaeyiaIiIaeqyTduMaeyOpa4JaaGim<br />% aiaacYcacqGHdicjcaWGJbGaeyicI4SaamyqaiaacQdacqGHsislca<br />% WGHbGaeyOeI0IaeqyTduMaeyipaWJaam4yaiaabccacaqGSaGaaeii<br />% aiaabohacaqGLbGaaeiiaiaabohacaqGPbGaae4zaiaabwhacaqGLb<br />% GaaeOoaiaabccacaWGHbGaey4kaSIaeqyTduMaeyypa0JaeyOeI0Ya<br />% aeWaaeaacqGHsislcaWGHbaacaGLOaGaayzkaaGaey4kaSIaeqyTdu<br />% MaeyOpa4JaeyOeI0Iaam4yaiaabccacaqGSaGaaeiiaaqaaiaab6ga<br />% caqGVbGaaeiDaiaabggacaqGYbGaaeiiaiaabghacaqG1bGaaeyzai<br />% aabccacqGHsislcaWGHbGaeyicI4SaeyOeI0IaamyqaiaabccacaqG<br />% 5bGaaeiiaiabgkHiTiaadogacqGHiiIZcqGHsislcaWGbbGaaeilai<br />% aabccacaqGKbGaaeyzaiaabccacaqGHbGaaeiAaiaabMgacaqGGaGa<br />% ae4CaiaabwgacaqGGaGaae4yaiaab+gacaqGUbGaae4yaiaabYgaca<br />% qG1bGaaeyEaiaabwgacaqGGaGaaeyCaiaabwhacaqGLbGaaeiiaiGa<br />% cMgacaGGUbGaaiOzamaabmaabaGaeyOeI0IaamyqaaGaayjkaiaawM<br />% caaiabg2da9iaadggacaGGUaaabaGaeyO0H4Taci4CaiaacwhacaGG<br />% WbWaaeWaaeaacaWGbbaacaGLOaGaayzkaaGaeyypa0JaeyOeI0Iaci<br />% yAaiaac6gacaGGMbWaaeWaaeaacqGHsislcaWGbbaacaGLOaGaayzk<br />% aaaaaaa!577A!<br />\[<br />\begin{gathered}<br /> \boxed{{\text{S}}{\text{.P1}}} \hfill \\<br /> {\text{Como A es acotado superiormente}}{\text{, entonces por axioma del supremo se tiene su existencia}} \hfill \\<br /> {\text{y por ende: }}\sup \left( A \right) \geqslant x{\text{, }}\forall x \in A{\text{ }}{\text{, luego: }} - \sup \left( A \right) \leqslant - x{\text{. Por tanto}}{\text{, el conjunto }} - A{\text{ es }} \hfill \\<br /> {\text{acotado inferiormente}}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{Si }}\sup \left( A \right) = - a \Rightarrow \forall \varepsilon > 0,\exists c \in A: - a - \varepsilon < c{\text{ }}{\text{, se sigue: }}a + \varepsilon = - \left( { - a} \right) + \varepsilon > - c{\text{ }}{\text{, }} \hfill \\<br /> {\text{notar que }} - a \in - A{\text{ y }} - c \in - A{\text{, de ahi se concluye que }}\inf \left( { - A} \right) = a. \hfill \\<br /> \Rightarrow \sup \left( A \right) = - \inf \left( { - A} \right) \hfill \\ <br />\end{gathered} <br />\]$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

El Geek Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 17 2011, 11:33 PM Publicado: #4
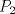Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 2.818 Registrado: 3-October 09 Miembro Nº: 59.773 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	El $TEX: $P_2$$ me suena a los tiempos remotos de los babilonios -------------------- Me voy, me jui.

DeadLock Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 29 2011, 12:24 AM Publicado: #5
Principiante Matemático Grupo: Usuario FMAT Mensajes: 1 Registrado: 11-January 10 Miembro Nº: 65.018 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: Es relativamente sencillo demostrar su límite: \\<br />Dado que $ a_n \to l $, también ocurrirá que $ a_{n+1} \to l $, por lo que se tendrá que: <br />$$ l = \frac{1}{2} \left( l + \frac{a}{l}\right) $$<br />Lo que implica que, \\<br />$$ 2l = l + \frac{a}{l} \hspace{2mm} \Longleftrightarrow \hspace{2mm} l^{2} = a $$<br />Llegando así a que $ l = \sqrt{a} $, lo cual es válido ya que se definió a $ a $ como una constante mayor a cero por lo que su raíz existe en los reales. \\<br />El único problema es que no me da que es decreciente, sino que creciente. Alguien podría exponer su desarrollo? gracias <img src="style_emoticons/default/biggrin.gif" style="vertical-align:middle" emoid=":D" border="0" alt="biggrin.gif" /><br />$

Lichiel Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 14 2014, 11:02 AM Publicado: #6
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 736 Registrado: 3-December 12 Miembro Nº: 113.971 Nacionalidad: Universidad: Sexo:	$TEX: P1-b. si $A$ es no vacío y acotado superiormente entonces existe un M real tal que $\forall x; x\leq M $, luego <br />$cM$ es cota superior de $cA$.<br /><br /> $\sup{A}$ es la menor cota superior de $A$ entonces $ x \leq \sup{A}$, \\ Luego $ cx \leq c \sup{A}$, $cx \in cA $, Entonces la menor cota superior de $cA$ es $\sup{cA} $ y $ cx \leq \sup{cA} \leq c\sup{A}$ eso implica que $ x \leq \frac{\sup{cA}}{c} \leq \sup{A}$ con $x \in A$ luego $ \sup{A} \leq \frac{\sup{cA}}{c} \leq \sup{A}$. Por lo tanto $c\sup{A}=\sup{cA}$. $\blacksquare$$ -------------------- $TEX: \begin{center} $ \aleph_0$ $<$ $\|?\|$ $< \aleph_1 $ \end{center}$ $TEX: Teorema: Si 2 personas tienen el mismo RUT entonces son la misma o existe un delito o el registro civil cometió un error, Denuncie.$ Quiero plata

« Posterior · Cálculo I · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 06:02 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.