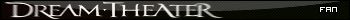Segunda PEP (2-2010) - Foro fmat.cl

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Santiago de Chile > Tópicos II

Segunda PEP (2-2010), variable compleja

Opciones

Misplaced Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 15 2011, 01:25 AM Publicado: #1
Dios Matemático Grupo: Usuario FMAT Mensajes: 223 Registrado: 10-October 06 Miembro Nº: 2.459 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Segunda Prueba Parcial Tópicos de Matemática II Ingeniería Civil $TEX: <br />\[<br />\begin{gathered}<br /> {\text{1) Considere la trayectoria }}C{\text{ formada por la recta que une los puntos }}P = (0,0) \hfill \\<br /> {\text{con }}Q(1,1){\text{ y el semicirculo con centro }}M = (0.5,0.5){\text{ y radio r = }}\frac{{\sqrt 2 }}<br />{2}{\text{ en direccion}} \hfill \\<br /> {\text{antihorario para calcular el valor de la integral de linea }}\oint_C {\overline z dz} \hfill \\<br /> \hfill \\<br /> {\text{2) Calcular el valor de la integral}} \hfill \\<br /> \int\limits_{\left\| {z - (1 + i)} \right\| = \sqrt {18} } {\left( {\frac{{e^{ - z} \sin (\pi z)dz}}<br />{{z^3 - z^2 - 8z + 12}}} \right)} \hfill \\<br /> \hfill \\<br /> {\text{3) Considere la serie de Laurent}} \hfill \\<br /> f(z) = \sum\limits_{n = 1}^\infty {\left( {\frac{{( - 1)^n }}<br />{{2^{n - 1} }} \cdot (z - 1)^{ - n} + ( - 1)^{2n + 1} \cdot (z - 1)^n } \right)} \hfill \\<br /> {\text{Encuentre el dominio anular de convergencia de la funcion }}f(z) \hfill \\ <br />\end{gathered} <br />\]<br />$ Tiempo: 120 minutos Mensaje modificado por Misplaced el Jan 15 2011, 09:01 PM -------------------- Eduardo Purin M. Estudiante de Ingenieria Civil en Industria, USACH 6to Año

Misplaced Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 15 2011, 10:42 PM Publicado: #2
Dios Matemático Grupo: Usuario FMAT Mensajes: 223 Registrado: 10-October 06 Miembro Nº: 2.459 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Control#2 Tópicos Matemáticos II Carrera: Ingeniería Civil $TEX: <br />\[<br />\begin{gathered}<br /> {\text{1}}{\text{. - Calcular el valor de las siguientes integrales}} \hfill \\<br /> {\text{a) }}\int\limits_{ - \infty }^\infty {\frac{{dx}}<br />{{(1 + x^2 )^3 }}} \hfill \\<br /> {\text{b) }}\int\limits_0^\pi {\frac{{dx}}<br />{{1 + a\cos x}}{\text{ con }}a^2 < 1} \hfill \\<br /> \hfill \\<br /> {\text{2}}{\text{. - Hacer un desarrollo en serie de Laurent() de la funci\'on }}f(z) = \frac{{2z + 1}}<br />{{z^2 + 3z - 4}} \hfill \\<br /> {\text{en la regi\'on anular }}1 < \left\| z \right\| < 4. \hfill \\<br /> \hfill \\<br /> {\text{3}}{\text{. - Calcular el valor de la integral }}\int\limits_\gamma {(1 + z + z^2 )e^{\frac{1}<br />{z}} dz} \hfill \\<br /> {\text{donde }}\gamma :{\text{ }}z(t) = 5e^{it} {\text{ }}0 \leqslant t \leqslant 2\pi \hfill \\ <br />\end{gathered} <br />\]<br />$ Comentario: () en la prueba no se explicita el desarrollo pedido, puede ser en serie de potencia o laurent, pero Gómez tenía en la pauta con serie de Laurent... - La pregunta 3 yo la pregunté en el foro cuando estaba desesperadamente estudiando esta materia por ahi debe estar... Mensaje modificado por Misplaced el Jan 15 2011, 10:44 PM -------------------- Eduardo Purin M. Estudiante de Ingenieria Civil en Industria, USACH 6to Año

master_c	May 26 2011, 03:40 PM Publicado: #3
Invitado	pregunta dos de la prueba Es facil notar la factorizacion 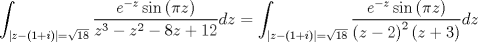$TEX: $$<br />\int_{\left\| {z - \left( {1 + i} \right)} \right\| = \sqrt {18} } {\frac{{e^{ - z} \sin \left( {\pi z} \right)}}<br />{{z^3 - z^2 - 8z + 12}}dz} = \int_{\left\| {z - \left( {1 + i} \right)} \right\| = \sqrt {18} } {\frac{{e^{ - z} \sin \left( {\pi z} \right)}}<br />{{\left( {z - 2} \right)^2 \left( {z + 3} \right)}}dz} <br />$$<br />$ ahora $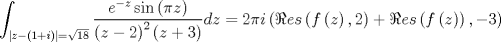TEX: $$<br />\int_{\left\| {z - \left( {1 + i} \right)} \right\| = \sqrt {18} } {\frac{{e^{ - z} \sin \left( {\pi z} \right)}}<br />{{\left( {z - 2} \right)^2 \left( {z + 3} \right)}}dz} = 2\pi i\left( {\Re es\left( {f\left( z \right),2} \right) + \Re es\left( {f\left( z \right)} \right), - 3} \right)<br />$$$ ya que ambos son polos interiores con 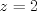$TEX: $z = 2$$ orden dos de aqui es directo que 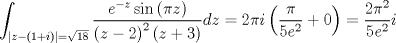$TEX: $$<br />\int_{\left\| {z - \left( {1 + i} \right)} \right\| = \sqrt {18} } {\frac{{e^{ - z} \sin \left( {\pi z} \right)}}<br />{{\left( {z - 2} \right)^2 \left( {z + 3} \right)}}dz} = 2\pi i\left( {\frac{\pi }<br />{{5e^2 }} + 0} \right) = \frac{{2\pi ^2 }}<br />{{5e^2 }}i<br />$$$ Mensaje modificado por master_c el May 26 2011, 03:41 PM

« Posterior · Tópicos II · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 10:08 PM