 Primera PEP (2-2010), variable compleja |
|
|
|
|
|
|
Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Santiago de Chile > Tópicos II  |
 Jan 15 2011, 01:02 AM
Publicado:
#1
|
|
 Dios Matemático  Grupo: Usuario FMAT Mensajes: 223 Registrado: 10-October 06 Miembro Nº: 2.459 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:   |
Para que se diviertan
 Primera Prueba Parcial Tópicos de Matemática II Carrera: Ingeniería Civil ![TEX: <br /> \[<br />\begin{gathered}<br /> {\text{1) a) Resuelva la ecuaci\'on: }}\cosh (z) - \sinh(z) = 1 \hfill \\<br /> {\text{b) Encuentre los valores de z que satisfacen la identidad }}(z - 1)^4 = \frac{{16 + 16i}}<br />{{\sqrt 2 }} \hfill \\<br /> \hfill \\<br /> {\text{2) Sea }}A = \{ z/\left| {2z - 6} \right| = 4\} {\text{ Calcular el valor de }}f(A){\text{ si }}f(z) = \frac{1}<br />{{iz}} + 1 - i \hfill \\<br /> \hfill \\<br /> 3){\text{ Considere la funci\'on }}u(x,y) = \cosh (y) \cdot senh(x){\text{ con }}(x,y) \in \mathbb{R}^2 \hfill \\<br /> {\text{a) Determine si existe la funci\'on }}v(x,y){\text{ tal que }}f(x,y,c) = u(x,y) + i(v(x,y) + c){\text{ es anal\'itica}} \hfill \\<br /> {\text{b) Determine la constante c tal que }}f(1 + 2i) = - 3 + 4i{\text{ }} \hfill \\<br /> {\text{ Si la respuesta es afirmativa escriba la funci\'on en terminos de la variable compleja z}} \hfill \\<br /> \hfill \\<br /> {\text{4) Considere la funci\'on }}f(z) = arc\csc(z) \hfill \\<br /> {\text{a) Calcule el valor de }}f(3){\text{ para la rama tal que }}f(2) = - i\ln \left( {\frac{{\sqrt 3 + i}}<br />{2}} \right) \hfill \\<br /> {\text{b) Calcule para esta rama }}H'(0){\text{ donde }}H(z) = arc\csc(\cosh (z)) \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/67cc075357bddc38b33017aff25f5f1a.png) Tiempo: 90 minutos Mensaje modificado por Misplaced el Jan 15 2011, 10:18 PM --------------------    Eduardo Purin M. Estudiante de Ingenieria Civil en Industria, USACH 6to Año |
 |
 
|
|
Jan 15 2011, 10:04 PM
Publicado:
#2
|
|
|
Dios Matemático Grupo: Usuario FMAT Mensajes: 223 Registrado: 10-October 06 Miembro Nº: 2.459 Nacionalidad: Colegio/Liceo: Universidad: Sexo: |
Control#1 Tópicos Matemáticos II Carrera: Ingeniería Civil ![TEX: <br />\[<br />\begin{gathered}<br /> {\text{1}}{\text{. - Sea }}f:\mathbb{C} \to \mathbb{C}{\text{ anal\'itica tal que }}f(0) = 6{\text{ y }}\operatorname{Re} (f'(z)) = 2e^{2x} \cos (2y) + 3 \hfill \\<br /> {\text{a) Determine }}f(x,y) \hfill \\<br /> {\text{b) Expresar en t\'erminos de la variable compleja z}} \hfill \\<br /> \hfill \\<br /> {\text{2}}{\text{. - a) Caracterice el valor de }}\arctan h(2){\text{ en t\'erminos de la funci\'on logaritmo}}{\text{.}} \hfill \\<br /> {\text{Considere la rama tal que }}\arctan h(0) = 0. \hfill \\<br /> {\text{b) Calcule el valor de }} - 3 \cdot \arctan h'(2) + (0,5 \cdot \ln ( - 3))^{ - 1} \cdot \arctan h(2) \hfill \\<br /> \hfill \\<br /> {\text{3}}{\text{. - Dada la funci\'on }}f(x,y) = y^2 x - \frac{2}<br />{3}y^3 + i\left( {x^2 y - \frac{2}<br />{3}x^3 } \right) \hfill \\<br /> {\text{a) Calcular la derivada en los puntos en que ella exista}}{\text{.}} \hfill \\<br /> {\text{b) ¿Se puede construir una funci\'on anal\'itica }}g(z){\text{ en el plano complejo tal que}} \hfill \\<br /> \operatorname{Im} (g(z)) = x^2 y - \frac{2}{3}x^3 {\text{? En caso afirmativo determinar }}g(z). \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/2a0deb6a6ad18b56d0eb0afcee31694c.png)
-------------------- Eduardo Purin M. Estudiante de Ingenieria Civil en Industria, USACH 6to Año |
|
|
|
|
Apr 14 2014, 05:52 PM
Publicado:
#3
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 817 Registrado: 21-February 14 Miembro Nº: 127.064 |
1)
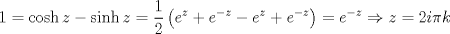 Otro dia lo resuelvo entero, ojala alguien se motive 
|
|
|
|
|
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 26th October 2025 - 10:54 PM |
