Putnam 1999 - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Sector Preparación Olimpiadas > Competencias y Olimpiadas > Competencias Universitarias

Reply to this topic

Start new topic

Putnam 1999

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 29 2010, 03:02 PM Publicado: #1
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	Putnam_1999.pdf ( 31.21k ) Número de descargas: 86

~Fatal_Collapse~ Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 29 2010, 07:47 PM Publicado: #2
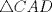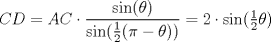Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.564 Registrado: 12-November 07 Desde: La Union, XIV Region de los Rios Miembro Nº: 12.607 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	B1: Como $TEX: $AC=AD=1$$ , se sigue por el Teorema del Seno aplicado al $TEX: $\triangle CAD$$ se tiene que $TEX: $CD=AC\cdot \dfrac{\sin (\theta)}{\sin (\frac{1}{2}({\pi-\theta}))}=2\cdot \sin (\frac{1}{2}\theta)$$ Ahora veamos que 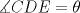$TEX: $\measuredangle {ECD}=\frac{1}{2}\pi - \measuredangle {DCA}=\frac{1}{2}\theta$$ . 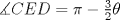Como $TEX: $\measuredangle CDE=\theta$$ , se sigue que $TEX: $\measuredangle CED=\pi-\frac{3}{2}\theta$$ . 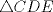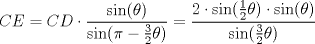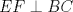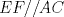Ahora, aplicando el Teorema del Seno al $TEX: $\triangle CDE$$ , obtenemos que $TEX: $CE=CD\cdot \dfrac{\sin (\theta)}{\sin (\pi-\frac{3}{2}\theta)}=\dfrac{2\cdot \sin (\frac{1}{2}\theta)\cdot \sin(\theta)}{\sin (\frac{3}{2}\theta)}$$ Por otra parte, como $TEX: $EF\perp BC$$ y $TEX: $AC\perp BC$$ , se cumple que $TEX: $EF//AC$$ . 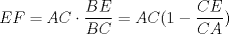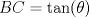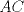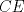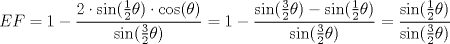En consecuencia, por el Teorema de Thales, $TEX: $EF=AC\cdot \dfrac{BE}{BC}=AC(1-\dfrac{CE}{CA})$$ Como $TEX: $BC=\tan (\theta)$$ y sabemos las longitudes de $TEX: $AC$$ y $TEX: $CE$$ , obtenemos finalmente que $TEX: $EF=1-\dfrac {2\cdot \sin(\frac{1}{2}\theta)\cdot \cos(\theta)}{\sin (\frac{3}{2}\theta)}=1-\dfrac{\sin (\frac{3}{2}\theta)-\sin(\frac{1}{2}\theta)}{\sin (\frac{3}{2}\theta)}=\dfrac{\sin (\frac{1}{2}\theta)}{\sin (\frac{3}{2}\theta)}$$ Recordando que 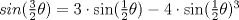$TEX: $sin (\frac{3}{2}\theta)=3\cdot \sin (\frac{1}{2}\theta)-4\cdot \sin (\frac{1}{2}\theta)^3$$ , podemos concluir que $TEX: $\displaystyle\lim_{\theta\to 0} EF= \displaystyle \lim_{\theta\to 0}\dfrac{\sin (\frac{1}{2}\theta)}{\sin (\frac{3}{2}\theta)}=\displaystyle \lim_{x\to 0}\dfrac{x}{3x-4x^3}=\dfrac{1}{3} $$ -------------------- Ricardo Vargas Obando Ex-alumno Deutsche Schule La Unión (Generación 2010, de los 150 años). Novato de Licenciatura en Matemática/Estadística, en la Pontificia Universidad Católica de Chile. Grupo de facebook de Novatos Matemática y Estadística PUC 2011 Currículum Olímpico: Medalla de Plata, XIX Olimpiada Nacional de Matemática Nivel Menor (2007) Medalla de Oro, XXI Olimpiada Nacional de Matemática Nivel Mayor (2009) Medalla de Bronce, XXV Olimpiada Iberoamericana de Matemática (2010) Medalla de Oro, XXII Olimpiada Nacional de Matemática Nivel Mayor (2010) "What we learned as children, that one plus one equals two, we know to be false. One plus one equals one. We even have a word when you plus another, equals one. That word is love." "Todos piensan en cambiar el mundo, pero nadie piensa en cambiarse a sí mismo."

OckUC Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 31 2010, 06:59 PM Publicado: #3
Dios Matemático Grupo: Usuario FMAT Mensajes: 288 Registrado: 25-August 09 Desde: Por ahí Miembro Nº: 57.644 Nacionalidad: Universidad: Sexo:	A2 Sea el conjunto de los polinomios no negativos: $TEX: $$P=\left\{ p\left( x \right)\text{ polinomio}\|p\left( x \right)\ge 0,\forall x\in \mathbb{R} \right\}$$$ P se puede construir de la siguiente manera: $TEX: $$\bullet x^{2n}\in P,\text{ con }n\in \mathbb{Z}_{0}^{+}$$$ $TEX: $$\bullet p\left( x \right)\in P\Rightarrow q\left( x \right)=p\left( x-c \right)\in P,\text{ con }c\in \mathbb{R}$$$ $TEX: $$\bullet p\left( x \right)\in P\Rightarrow \alpha \cdot p\left( x \right)\in P,\text{ con }\alpha \ge 0$$$ $TEX: $$\bullet p\left( x \right)\in P\wedge q\left( x \right)\in P\Rightarrow p\left( x \right)+q\left( x \right)\in P$$$ Por demostrar: $TEX: $$\forall p\left( x \right)\in P\Rightarrow \exists k\in \mathbb{N}\text{ y existen polinomios }f_{1}\left( x \right),...,f_{k}\left( x \right)\text{ tal que }p\left( x \right)=\sum\limits_{j=1}^{k}{\left( f_{j}\left( x \right) \right)^{2}}$$$ Usando inducción sobre la construcción de P: $TEX: $$B.I.:\text{ Sea }n\in \mathbb{Z}_{0}^{+},\text{ entonces }x^{2n}=\left( x^{n} \right)^{2}=\sum\limits_{j=1}^{1}{\left( f_{j}\left( x \right) \right)^{2}}$$$ $TEX: $$\text{Como }f_{1}\left( x \right)=x^{n}\text{ es polinomio}\text{, queda demostrado para el caso base}\text{.}$$$ $TEX: $$H.I.:\text{ Supongamos que }p_{1}\left( x \right)\in P\text{ y }p_{2}\left( x \right)\in P\text{ son tales que }p_{1}\left( x \right)=\sum\limits_{j=1}^{k}{\left( f_{j}\left( x \right) \right)^{2}}$$$ $TEX: $$y\text{ }p_{2}\left( x \right)=\sum\limits_{i=1}^{m}{\left( g_{i}\left( x \right) \right)^{2}}\text{ con }f_{j}\left( x \right)\text{ y }g_{i}\left( x \right)\text{ polinomios}\text{.}$$$ $TEX: $$T.I.:\text{ Por demostrar: }q_{1}\left( x \right)=p_{1}\left( x-c \right)\text{, }q_{2}\left( x \right)=\alpha \cdot p_{1}\left( x \right)\text{ y }q_{3}\left( x \right)=p_{1}\left( x \right)+p_{2}\left( x \right)$$$ $TEX: $$\text{son sumas de cuadrados de polinomios}\text{.}$$$ $TEX: $$\text{Sea }c\in \mathbb{R}\text{ y }q_{1}\left( x \right)=p_{1}\left( x-c \right).\text{ Por H}\text{.I}\text{. se tiene q}\left( x \right)=p_{1}\left( x-c \right)=\sum\limits_{j=1}^{k}{\left( f_{j}\left( x-c \right) \right)^{2}}$$$ $TEX: $$\text{ y dado que }f_{j}\left( x-c \right)\text{ son polinomios}\text{, (composicion de polinomios es polinomio)}$$$ $TEX: $$\text{se completa la demostracion}\text{.}$$$ $TEX: $$\text{Sea }\alpha \ge \text{0 y }q_{2}\left( x \right)=\alpha \cdot p_{1}\left( x \right).\text{ Por H}\text{.I}\text{. se tiene }q_{2}\left( x \right)=\alpha \cdot p_{1}\left( x \right)=\alpha \cdot \sum\limits_{j=1}^{k}{\left( f_{j}\left( x \right) \right)^{2}}$$$ $TEX: $$\text{Como }\alpha \ge 0\Rightarrow q_{2}\left( x \right)=\sum\limits_{j=1}^{k}{\alpha \cdot \left( f_{j}\left( x \right) \right)^{2}}=\sum\limits_{j=1}^{k}{\left( \sqrt{\alpha }\cdot f_{j}\left( x \right) \right)^{2}}$$$ $TEX: $$\text{Y como }\sqrt{\alpha }\cdot f_{j}\left( x \right)\text{ es polinomio}\text{, se completa la demostracion}\text{.}$$$ $TEX: $$\text{Sea }q_{3}\left( x \right)=p_{1}\left( x \right)+p_{2}\left( x \right).\text{ Por H}\text{.I}\text{. se tiene }q_{3}\left( x \right)=\sum\limits_{j=1}^{k}{\left( f_{j}\left( x \right) \right)^{2}}+\sum\limits_{i=1}^{m}{\left( g_{i}\left( x \right) \right)^{2}}=\sum\limits_{i=1}^{k+m}{\left( h_{i}\left( x \right) \right)^{2}}$$$ $TEX: $$\text{donde }h_{i}\left( x \right)=\left\{ \begin{matrix}<br /> f_{i}\left( x \right) & \text{si }1\le i\le k \\<br /> g_{i-k}\left( x \right) & \text{si }k+1\le i\le k+m \\<br />\end{matrix} \right.\text{ es polinomio}\text{. Luego se completa la demostracion}\text{.}$$$ $TEX: $$\text{Se concluye que si }p\left( x \right)\text{ es polinomio no negativo}\text{, }$$$ $TEX: $$\text{existen polinomios }f_{1}\left( x \right),...,f_{k}\left( x \right)\text{ tal que }p\left( x \right)=\sum\limits_{j=1}^{k}{\left( f_{j}\left( x \right) \right)^{2}}$$$ -------------------- RECURSIÓN: Si no lo entiende, vea RECURSIÓN $TEX: Conjunto $R$:$ $TEX: <br />$$R=\{X:X\notin X\}$$<br />$ $TEX: <br />$$R\in R\Leftrightarrow R\notin R$$<br />$

mamboraper Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 20 2023, 04:59 PM Publicado: #4
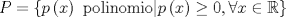
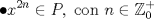
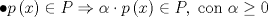
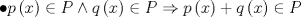
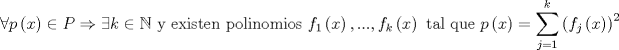
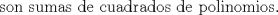
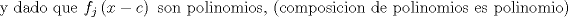
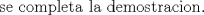
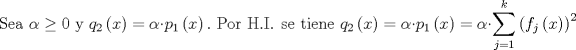
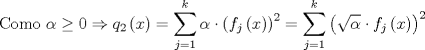
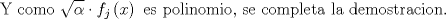
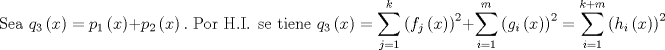
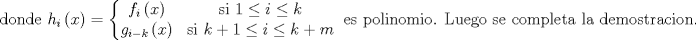
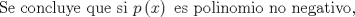
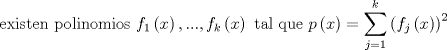
Maestro Matemático Grupo: Usuario FMAT Mensajes: 134 Registrado: 28-March 14 Miembro Nº: 128.100 Nacionalidad: Sexo:	A5 $TEX: Sea $\mathcal{P}_{1999}$ el espacio vectorial de los polinomios de grado menor o igual a 1999 definidos en $[-1,1]$. Este es un espacio de dimensión finita. Definimos la función lineal $T\colon \mathcal{P}_{1999}\to \mathbb{R}$ tal que $T(p) = p(0)$. Sobre $\mathcal{P}_{1999}$, consideramos las normas $\\|p\\|_\infty := \max_{x\in [-1,1]}\|p(x)\|$ y $\\|p\\|_1=\int_{-1}^{1} \|p(x)\|dx$, como $\mathcal{P}_{1999}$ es de dimensión finita, estas normas son equivalentes, por lo tanto existe $C>0$ tal que $\\|p\\|_\infty\leq C\\|p\\|_1$ para todo $p\in \mathcal{P}_{1999}$, luego $$\|p(0)\|\leq C\\|p\\|_1=C\int_{-1}^{1} \|p(x)\|dx, \ \forall p\in \mathcal{P}_{1999}.$$$ -------------------- Hago clases particulares (activo 2024). Cualquier consulta por MP.

« Posterior · Competencias Universitarias · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 3rd April 2025 - 10:15 AM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.