|
|
|
|
|
|
|
  |
 Jun 26 2010, 12:58 AM Jun 26 2010, 12:58 AM
Publicado:
#21
|
|
|
Principiante Matemático Grupo: Usuario FMAT Mensajes: 1 Registrado: 1-June 09 Miembro Nº: 52.878 Nacionalidad:  Colegio/Liceo:  Sexo:  |
|
|
|
|
 Jun 27 2010, 01:21 AM Jun 27 2010, 01:21 AM
Publicado:
#22
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 314 Registrado: 14-March 09 Desde: San Miguel Miembro Nº: 44.908 Nacionalidad:  Universidad:  Sexo:  |
--------------------         |
|
|
|
 Jun 27 2010, 05:00 PM Jun 27 2010, 05:00 PM
Publicado:
#23
|
|
 Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 15 Registrado: 24-March 09 Desde: Valparaíso Miembro Nº: 45.904 Nacionalidad:  Universidad:  Sexo:  |
b){\text{z}}_{\text{1}} {\text{z}}_2 {\text{ + z}}_2 {\text{z}}_3 {\text{ + z}}_3 {\text{z}}_1 = \hfill \\ (1 + 2i)( - 2 + 3i) + ( - 2 + 3i)(1 - i) + (1 + 2i)(1 - i) = \hfill \\ 2 + 3i - 4i + 3i \bullet 2i + - 2 + 2i + 3i - 3i \bullet i + 1 - i + 2i - 2i \bullet i = \hfill \\ 1 + 5i + 6i^2 - 3i^2 - 2i^2 = \hfill \\ 1 + 5i - 6 + 3 + 2 = 5i \hfill \\ \hfill \\ En la b me da -4+5i |
|
|
|
 Jun 27 2010, 10:25 PM Jun 27 2010, 10:25 PM
Publicado:
#24
|
|
 Maestro Matemático Grupo: Usuario FMAT Mensajes: 87 Registrado: 2-November 09 Miembro Nº: 61.366 Nacionalidad:  Universidad:  Sexo:  |
Gracias por la guia Infiltrandome nuevamente dx... ![TEX: \[<br />\begin{gathered}<br /> {\text{1}}{\text{.Considere los complejos }}z_1 = 1 + 2i,{\text{ }}z_2 = - 2 + 3i{\text{ y }}z_3 {\text{ = }}1 - i.{\text{ }} \hfill \\<br /> {\text{Calcular}} \hfill \\<br /> {\text{a) z}}_{\text{1}} {\text{ + z}}_2 {\text{ + z}}_3 = \hfill \\<br /> (1 + 2i) + ( - 2 + 3i) + (1 - i) = 4i \hfill \\<br /> \hfill \\<br /> b){\text{z}}_{\text{1}} {\text{z}}_2 {\text{ + z}}_2 {\text{z}}_3 {\text{ + z}}_3 {\text{z}}_1 = \hfill \\<br /> (1 + 2i)( - 2 + 3i) + ( - 2 + 3i)(1 - i) + (1 + 2i)(1 - i) = \hfill \\<br /> 2 + 3i - 4i + 3i \bullet 2i + - 2 + 2i + 3i - 3i \bullet i + 1 - i + 2i - 2i \bullet i = \hfill \\<br /> 1 + 5i + 6i^2 - 3i^2 - 2i^2 = \hfill \\<br /> 1 + 5i - 6 + 3 + 2 = 5i \hfill \\<br /> \hfill \\<br /> c)\frac{{{\text{z}}_1^2 {\text{ + z}}_2^2 }}<br />{{{\text{z}}_2^2 + {\text{z}}_3^2 }} = \frac{{(1 + 2i)^2 + ( - 2 + 3i)^2 }}<br />{{( - 2 + 3i)^2 + (1 - i)^2 }} \hfill \\<br /> = \frac{{(1 + 4i + 4i^2 ) + (4 - 12i + 9i^2 )}}<br />{{(4 - 12i + 9i^2 ) + (1 - 2i + i^2 )}} \hfill \\<br /> = \frac{{5 - 8i + 13i^2 }}<br />{{5 - 14i + 10i^2 }} \hfill \\<br /> = \frac{{5 - 13 - 8i}}<br />{{5 - 10 - 14i}} \hfill \\<br /> = \frac{{ - 8 - 8i}}<br />{{ - 5 - 14i}} = \frac{{ - (8 + 8i)}}<br />{{ - (5 + 14i)}} = \frac{{8 + 8i}}<br />{{5 + 14i}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/2ebaf9615c9edfb56861cac629200d54.png) La b no deberia ser -4+5i? xD z1*z2=-8-i z2*z3=1+5i z3*z1=3+i Todo eso da -4+5i, diganme donde esta mi error si yo me equivoque, siempre se me pasan puras idioteces :B xD -------------------- Bazinga!
|
|
|
|
 Jun 27 2010, 11:54 PM Jun 27 2010, 11:54 PM
Publicado:
#25
|
|
 Matemático Grupo: Usuario FMAT Mensajes: 49 Registrado: 17-April 09 Desde: Valparaíso Miembro Nº: 48.536 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
|
|
|
|
 Jun 27 2010, 11:54 PM Jun 27 2010, 11:54 PM
Publicado:
#26
|
|
 Matemático Grupo: Usuario FMAT Mensajes: 49 Registrado: 17-April 09 Desde: Valparaíso Miembro Nº: 48.536 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
b){\text{z}}_{\text{1}} {\text{z}}_2 {\text{ + z}}_2 {\text{z}}_3 {\text{ + z}}_3 {\text{z}}_1 = \hfill \\ (1 + 2i)( - 2 + 3i) + ( - 2 + 3i)(1 - i) + (1 + 2i)(1 - i) = \hfill \\ 2 + 3i - 4i + 3i \bullet 2i + - 2 + 2i + 3i - 3i \bullet i + 1 - i + 2i - 2i \bullet i = \hfill \\ 1 + 5i + 6i^2 - 3i^2 - 2i^2 = \hfill \\ 1 + 5i - 6 + 3 + 2 = 5i \hfill \\ \hfill \\ En la b me da -4+5i D:!!!!, nu entendi |
|
|
|
 Jun 28 2010, 12:59 PM Jun 28 2010, 12:59 PM
Publicado:
#27
|
|
 Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 15 Registrado: 24-March 09 Desde: Valparaíso Miembro Nº: 45.904 Nacionalidad:  Universidad:  Sexo:  |
|
|
|
|
 Jun 28 2010, 05:19 PM Jun 28 2010, 05:19 PM
Publicado:
#28
|
|
|
Principiante Matemático Grupo: Usuario FMAT Mensajes: 9 Registrado: 1-June 08 Desde: Afta. Miembro Nº: 25.354 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
|
|
|
|
 Jun 28 2010, 05:23 PM Jun 28 2010, 05:23 PM
Publicado:
#29
|
|
 Dios Matemático Supremo Grupo: Team Ensayos FMAT Mensajes: 1.294 Registrado: 16-March 09 Desde: ancud Miembro Nº: 45.100 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
es lo mismo.
hagan el dibujito i les kedará claro. PD: entiendase por dibujito = representacion grafica xd --------------------   Estudiante de 4° año ing civil mec utfsm |
|
|
|
 Jun 28 2010, 07:50 PM Jun 28 2010, 07:50 PM
Publicado:
#30
|
|
|
Matemático Grupo: Usuario FMAT Mensajes: 49 Registrado: 26-March 10 Miembro Nº: 67.223 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
[quote name='O.K' date='Jun 22 2010, 11:38 PM' post='442764']
una miradita a estos porfavor 9. a) ![TEX: \[\begin{gathered}<br /> {\left( {\frac{{1 + i}}<br />{{1 - i}}} \right)^{16}} + {\left( {\frac{{1 - i}}<br />{{1 + i}}} \right)^8} \hfill \\<br /> {\left[ {\frac{{(1 + i)(1 + i)}}<br />{{(1 + i)(1 - i)}}} \right]^{16}}{\left[ {\frac{{(1 - i)(1 - i)}}<br />{{(1 + i)(1 - i)}}} \right]^8} \hfill \\<br /> z = {i^{16}} + {( - i)^8} = 1 + 1 = 2 \hfill \\ <br />\end{gathered} \]<br />](./tex/de0c97ed365263f5712f8990614b8deb.png) Ojo que piden el resultado como a+bi, y el 2 es el módulo solamente, a mi me quedó -1 + raiz(3)i |
|
|
|
  |
2 usuario(s) está(n) leyendo esta discusión (2 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 18th September 2025 - 12:52 PM |











![TEX: \[\begin{gathered}<br /> \theta = \frac{{ - \pi }}<br />{3} + 2k\pi \hfill \\<br /> \cos \left( {\frac{{ - \pi }}<br />{3}} \right) + sen\left( {\frac{{ - \pi }}<br />{3}} \right) = \cos \left( {\frac{{5\pi }}<br />{3}} \right) + sen\left( {\frac{{5\pi }}<br />{3}} \right) \hfill \\ <br />\end{gathered} \]<br />](./tex/5f92e17dfbd449df179befccdac42d74.png)

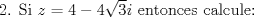
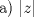
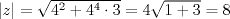
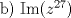
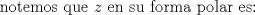
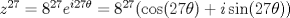

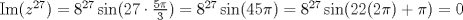
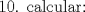
![TEX: $\sqrt[3]{\dfrac{1}{\cos\left(\frac{\pi}{7}\right)}}-\sqrt[3]{\dfrac{1}{\cos\left(\frac{2\pi}{7}\right)}}+\sqrt[3]{\dfrac{1}{\cos\left(\frac{3\pi}{7}\right)}}=\sqrt[3]{6\sqrt[3]{7}-8}$](./tex/c5b49cf6584e89b12a82db6b29f2cd73.png)