 Control 2 CVV 2010/1 Otoño, Juan Dávila / Manuel del Pino |
|
|
|
|
|
|
Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Chile > Cálculo en Varias Variables  |
 Jun 9 2010, 10:21 PM
Publicado:
#1
|
|
 Dios Matemático Supremo  Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:   |
![TEX: \[\begin{gathered}<br /> \left. {\underline {\, <br /> {P1} \,}}\! \right| \hfill \\<br /> a){\text{ Sea }}f:{\mathbb{R}^2} \to \mathbb{R}{\text{ de clase }}{C^2}{\text{ y definamos }}g\left( {u,v} \right) = f\left( {x,y} \right) \hfill \\<br /> {\text{donde }}x = u + v,\;y = u{v^2}.{\text{ Suponiendo que}} \hfill \\<br /> {\text{ }}\frac{{\partial f}}<br />{{\partial y}} = 2,\;\frac{{{\partial ^2}f}}<br />{{\partial {x^2}}} = \frac{{{\partial ^2}f}}<br />{{\partial x\partial y}} = \frac{{{\partial ^2}f}}<br />{{\partial {y^2}}} = 1 \hfill \\<br /> {\text{en }}x = 2,\;y = 1,{\text{ calcule}} \hfill \\<br /> {\text{ }}\frac{{{\partial ^2}g}}<br />{{\partial {u^2}}}\left( {1,1} \right),\;\frac{{{\partial ^2}g}}<br />{{\partial {v^2}}}\left( {1,1} \right) \hfill \\<br /> \hfill \\<br /> b){\text{ Suponga que }}f:{\mathbb{R}^n}\backslash \left\{ 0 \right\} \to \mathbb{R},\;n \geqslant 2{\text{ es una funcion }}{C^1}{\text{ tal}} \hfill \\<br /> {\text{que para cierta constante }}M \hfill \\<br /> {\text{ }}\left| {\frac{{\partial f}}<br />{{\partial {x_j}}}\left( x \right)} \right| \leqslant M\;\;\forall x \ne 0,\;\;\;\forall j \in \left\{ {1, \ldots ,n} \right\} \hfill \\<br /> {\text{ Demuestre que }}f{\text{ es lipschitz en }}{\mathbb{R}^n}\backslash \left\{ 0 \right\}{\text{, es decir}}{\text{, existe }}C{\text{ tal que}} \hfill \\<br /> {\text{ }}\left| {f\left( x \right) - f\left( y \right)} \right| \leqslant C\left\| {x - y} \right\|\;\;\;\forall x,y \in {\mathbb{R}^n}\backslash \left\{ 0 \right\}. \hfill \\ <br />\end{gathered} \]<br />](./tex/daf61ff873d012c56a40c3aa18bd6437.png) ![TEX: \[\begin{gathered}<br /> \left. {\underline {\, <br /> {P2} \,}}\! \right| \hfill \\<br /> a){\text{ Muestre que cerca del punto }}\left( {{x_0},{y_0},{u_0},{v_0}} \right) = \left( {0,1,1,1} \right){\text{ se}} \hfill \\<br /> {\text{puede resolver}} \hfill \\<br /> {\text{ }}\sin \left( x \right)u + y{v^2}u = 1,\;\;\;{u^2}v + xy{v^4} = 1 \hfill \\<br /> {\text{de manera unica para }}u,\;v{\text{ como funciones }}{C^1}{\text{ de }}x,\;y.{\text{ Calcule }}\frac{{\partial u}}<br />{{\partial x}}\;{\text{y}}\;\frac{{\partial v}}<br />{{\partial x}} \hfill \\<br /> {\text{en }}\left( {0,1} \right). \hfill \\<br /> \hfill \\<br /> b){\text{ Encuentre la expansion de Taylor de orden 2 de }} \hfill \\<br /> {\text{ }}f\left( {x,y} \right) = x\ln \left( {1 + y} \right) + \sin \left( {x + y} \right) \hfill \\<br /> {\text{en torno de }}\left( {0,0} \right). \hfill \\<br /> {\text{ Pruebe que para }}{x^2} + {y^2} \leqslant \frac{1}<br />{4}{\text{ se tiene}} \hfill \\<br /> {\text{ }}\left| {f\left( {x,y} \right) - {P_2}\left( {x,y} \right)} \right| \leqslant \frac{3}<br />{2}{\left( {\left| x \right| + \left| y \right|} \right)^3} \hfill \\<br /> {\text{donde }}{P_2}{\text{ es la expansion de orden 2 encontrada anteriormente}}{\text{.}} \hfill \\ <br />\end{gathered} \]<br />](./tex/02f4374d8738c7fe6c38ca8da614e137.png) ![TEX: \[\begin{gathered}<br /> \left. {\underline {\, <br /> {P3} \,}}\! \right| \hfill \\<br /> a){\text{ Encuentre los puntos criticos de}} \hfill \\<br /> {\text{ }}f\left( {x,y} \right) = xy{e^{ - {x^2} - {y^2}}} \hfill \\<br /> {\text{y determine si son maximos locales}}{\text{, minimos locales o puntos silla}}{\text{.}} \hfill \\<br /> \hfill \\<br /> b){\text{ Para }}f{\text{ la funcion de la parte anterior}}{\text{, pruebe que}} \hfill \\<br /> {\text{ }}\mathop {\lim }\limits_{\left\| {\left( {x,y} \right)} \right\| \to + \infty } f\left( {x,y} \right) = 0 \hfill \\<br /> {\text{y deduzca que}} \hfill \\<br /> {\text{ }}\mathop {\max }\limits_{\left( {x,y} \right) \in {\mathbb{R}^2}} f\left( {x,y} \right),\;\;\mathop {\min }\limits_{\left( {x,y} \right) \in {\mathbb{R}^2}} f\left( {x,y} \right) \hfill \\<br /> {\text{existen}}{\text{. Calcule estos valores}}{\text{.}} \hfill \\<br /> \left. {\underline {\, <br /> {{\text{Tiempo: 3 hrs}}{\text{.}}} \,}}\! \right| \hfill \\ <br />\end{gathered} \]<br />](./tex/3b4cfb99b846259683cb8914b5f12df8.png)
--------------------  Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
 |
 
|
|
May 19 2012, 01:46 AM
Publicado:
#2
|
|
|
Dios Matemático Grupo: Usuario FMAT Mensajes: 476 Registrado: 15-November 10 Miembro Nº: 80.334 Nacionalidad: Universidad:  Sexo:  |
P1: (b)
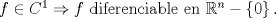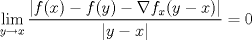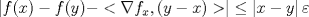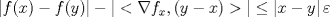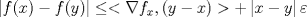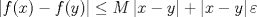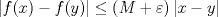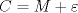         Espero no haberla embarrado en algo x.x |
|
|
|
|
May 19 2012, 03:24 AM
Publicado:
#3
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad: Colegio/Liceo: Universidad: Sexo: |
loko no te entendi nada xD
-------------------- Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
|
May 21 2012, 04:33 PM
Publicado:
#4
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 2.847 Registrado: 3-October 09 Miembro Nº: 59.760 Nacionalidad: Colegio/Liceo:  Universidad: Sexo: |
alguien me puede explicar como se sacan las segundas derivadas parciales de g(u,v) en la p1, entiendo como sacar la primera usando regla de la cadena, pero para las segundas no entendi como se hace
 se agradece desde ya 
|
|
|
|
|
May 21 2012, 05:52 PM
Publicado:
#5
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad: Colegio/Liceo: Universidad: Sexo: |
CITA(gamby @ May 21 2012, 05:33 PM)  alguien me puede explicar como se sacan las segundas derivadas parciales de g(u,v) en la p1, entiendo como sacar la primera usando regla de la cadena, pero para las segundas no entendi como se hace se agradece desde ya es un parto, en general cada derivada parcial por si sola depende de dos variables tambien, entonces tienes que aplicar la regla de la cadena para era derivada por si sola, así cuando tienes hartas funciones, el puro hecho de llegar a la 2º derivada se hace eterno xD -------------------- Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
|
May 22 2012, 05:40 PM
Publicado:
#6
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 2.847 Registrado: 3-October 09 Miembro Nº: 59.760 Nacionalidad: Colegio/Liceo: Universidad: Sexo: |
CITA(Crash! @ May 21 2012, 06:52 PM) es un parto, en general cada derivada parcial por si sola depende de dos variables tambien, entonces tienes que aplicar la regla de la cadena para era derivada por si sola, así cuando tienes hartas funciones, el puro hecho de llegar a la 2º derivada se hace eterno xD si xD creo que le encontré el patrón para resolverlas, aunque la p2 (a) me mato, 
|
|
|
|
|
May 22 2012, 09:48 PM
Publicado:
#7
|
|
|
Dios Matemático Grupo: Usuario FMAT Mensajes: 476 Registrado: 15-November 10 Miembro Nº: 80.334 Nacionalidad: Universidad: Sexo: |
CITA(Crash! @ May 19 2012, 04:24 AM) loko no te entendi nada xD ni yo xD |
|
|
|
|
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 4th April 2025 - 10:03 PM |
