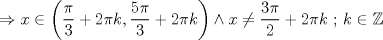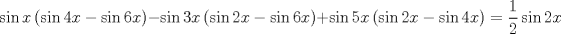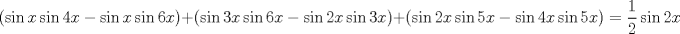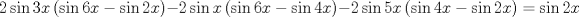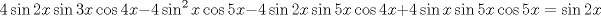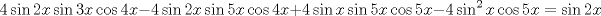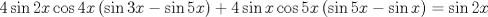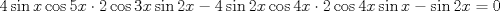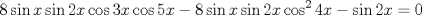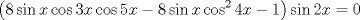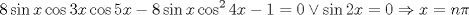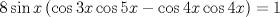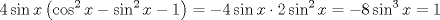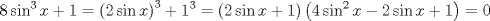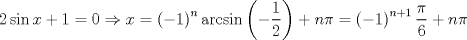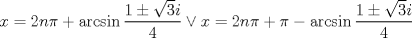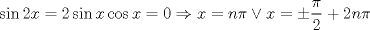|
|
|
|
|
|
|
  |
 May 24 2013, 12:08 AM May 24 2013, 12:08 AM
Publicado:
#61
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[\begin{gathered}<br /> {\text{obviamente tiene que ser un }}x{\text{ tal que:}} \hfill \\<br /> {\text{sin}}\left( {\pi \sqrt x } \right) = \sin \left( {\pi \sqrt {x - 6} } \right) = \pm 1 \hfill \\<br /> {\text{sean }}m,n \in \mathbb{Z}{\text{ tal que}} \hfill \\<br /> \pi \sqrt x = \pm \frac{\pi }{2} + 2n\pi \hfill \\<br /> \pi \sqrt {x - 6} = \pm \frac{\pi }{2} + 2m\pi \hfill \\<br /> \Leftrightarrow \hfill \\<br /> \sqrt x = \pm \frac{1}{2} + 2n \hfill \\<br /> \sqrt {x - 6} = \pm \frac{1}{2} + 2m \hfill \\<br /> x - 6 = \frac{1}{4} \pm 2m + 4{m^2} \hfill \\<br /> x = \frac{{25}}{4} \pm 2m + 4{m^2} = \frac{1}{4} \pm 2n + 4{n^2} \hfill \\<br /> 6 \pm 2m + 4{m^2} = \pm 2n + 4{n^2} \hfill \\<br /> 4\left( {{n^2} - {m^2}} \right) \pm 2\left( {n - m} \right) = 6 \hfill \\<br /> 4\left( {n - m} \right)\left( {n + m} \right) \pm 2\left( {n - m} \right) = 6 \hfill \\<br /> 2\left( {n - m} \right)\left\{ {2\left( {n + m} \right) \pm 1} \right\} = 6 \hfill \\<br /> \left( {n - m} \right)\left\{ {2\left( {n + m} \right) \pm 1} \right\} = 3 \hfill \\<br /> {\text{caso 1:}}\left( {n - m} \right) = 1\;\;\;;\;\;2\left( {n + m} \right) + 1 = 3 \hfill \\<br /> n = m + 1 \Rightarrow 2\left( {1 + 2m} \right) + 1 = 3 \Leftrightarrow 3 + 4m = 3 \Rightarrow \left( {n,m} \right) = \left( {1,0} \right) \hfill \\<br /> \Rightarrow x = \frac{{25}}{4} \hfill \\ <br />\end{gathered} \]](/tex-image/9ca93bb3f1aa49413c9588b207c10e04.png) ![TEX: \[\begin{gathered}<br /> {\text{caso 2:}}\left( {n - m} \right) = - 1\;\;\;;\;\;2\left( {n + m} \right) + 1 = - 3 \hfill \\<br /> n = m - 1 \hfill \\<br /> \;2\left( {2m - 1} \right) + 1 = - 3 \hfill \\<br /> 4m - 1 = - 3 \Rightarrow 4m = - 2 \Rightarrow m = - \frac{1}{2}{\text{ }}\left( {{\text{no sirve}}} \right) \hfill \\<br /> {\text{caso 3:}}\left( {n - m} \right) = 1\;\;\;;\;\;2\left( {n + m} \right) - 1 = 3 \hfill \\<br /> n = m + 1 \hfill \\<br /> 2\left( {2m + 1} \right) - 1 = 3 \Leftrightarrow 4m = 2 \Rightarrow m = \frac{1}{2}\;\left( {{\text{tampoco}}} \right) \hfill \\<br /> {\text{caso 4:}}\left( {n - m} \right) = - 1\;\;\;;\;\;2\left( {n + m} \right) - 1 = - 3 \hfill \\<br /> n = m - 1 \hfill \\<br /> \Rightarrow \;2\left( {2m - 1} \right) - 1 = - 3 \hfill \\<br /> 4m - 3 = - 3 \Rightarrow 4m = 0 \Rightarrow \left( {n,m} \right) = \left( { - 1,0} \right) \hfill \\<br /> x = \frac{{25}}{4} \hfill \\<br /> {\text{caso 5:}}\left( {n - m} \right) = 3\;\;\;;\;\;2\left( {n + m} \right) + 1 = 1 \hfill \\<br /> \Rightarrow n = - m \Rightarrow 2n = 3{\text{ }}\left( {{\text{no nos sirve}}} \right) \hfill \\<br /> {\text{caso 6:}}\left( {n - m} \right) = - 3\;\;\;;\;\;2\left( {n + m} \right) + 1 = - 1 \hfill \\<br /> \Rightarrow \left( {n + m} \right) = - 1{\text{ sumando}} \hfill \\<br /> 2n = - 4 \Rightarrow \left( {n,m} \right) = \left( { - 2,1} \right) \Rightarrow x = \frac{{49}}{4} \hfill \\<br /> {\text{caso 7:}}\left( {n - m} \right) = 3\;\;\;;\;\;2\left( {n + m} \right) - 1 = 1 \hfill \\<br /> \Rightarrow n + m = 1 \hfill \\<br /> 2n = 4 \Rightarrow \left( {n,m} \right) = \left( {2, - 1} \right) \Rightarrow x = \frac{{49}}{4} \hfill \\<br /> {\text{caso 8:}}\left( {n - m} \right) = - 3\;\;\;;\;\;2\left( {n + m} \right) - 1 = - 1 \hfill \\<br /> n = - m \hfill \\<br /> 2n = - 3{\text{ }}\left( {{\text{tampoco sirve}}} \right) \hfill \\<br /> {\text{asi}} \hfill \\<br /> x = \frac{{25}}{4} \vee x = \frac{{49}}{4} \hfill \\ <br />\end{gathered} \]](/tex-image/12535e81afef3453bf5947e71bb98c05.png) Que master_c proponga que de seguro tendrá un problema mejor que yo Mensaje modificado por Crash! el May 24 2013, 12:20 AM --------------------  Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed. |
|
|
|
| master_c |
 May 24 2013, 09:55 AM May 24 2013, 09:55 AM
Publicado:
#62
|
|
Invitado |
proppongo 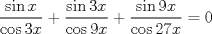 |
|
|
|
| master_c |
 May 25 2013, 01:35 PM May 25 2013, 01:35 PM
Publicado:
#63
|
|
Invitado |
ya que la maraton esta detenida dare un hint, si no lo quieres ver no lo veas
Mensaje modificado por master_c el May 25 2013, 01:37 PM |
|
|
|
 May 25 2013, 09:36 PM May 25 2013, 09:36 PM
Publicado:
#64
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 427 Registrado: 5-October 10 Miembro Nº: 78.264 Nacionalidad:  Universidad:  Sexo:  |
proppongo 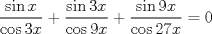 No te voy a mentir, la verdad ni se me ocurrió como hacer el problema, solo llegue a "algo" usando la forma de Euler, pero es bastante fea, y fue la única forma en que pude hacerlo xd Me falta más practica para las identidades y todo eso, la verdad me cuesta verlo en los ejercicios. Aquí va lo que "hice". Solución solo para los Reales, nada de complejos. con u = e^(ix) ![TEX: \[\frac{\frac{u-u^{-1}}{2i}}{\frac{u^{3}+u^{-3}}{2}}+\frac{\frac{u^{3}-u^{-3}}{2i}}{\frac{u^{9}+u^{-9}}{2}}+\frac{\frac{u^{9}-u^{-9}}{2i}}{\frac{u^{27}+u^{-27}}{2}}=\frac{u-u^{-1}}{i(u^{3}+u^{-3})}+\frac{u^{3}-u^{-3}}{i(u^{9}+u^{-9})}+\frac{u^{9}-u^{-9}}{i(u^{27}+u^{-27})}=0\]](/tex-image/595c25cb591cdbd21dbfc3d4e08dd1aa.png) Desarrollando las fracciones un poco: ![TEX: \[\frac{u^{4}-u^{2}}{i(u^{6}+1)}+\frac{u^{12}-u^{6}}{i(u^{18}+1)}+\frac{u^{36}-u^{18}}{i(u^{54}+1)}=0\]](/tex-image/d2b2f21ab05beeca92714ba8c1eff8b7.png) ![TEX: \[u^{2}\left (\frac{u^{2}-1}{i(u^{6}+1)}+\frac{u^{10}-u^{4}}{i(u^{18}+1)}+\frac{u^{34}-u^{16}}{i(u^{54}+1)} \right )=0\]](/tex-image/0188d5c196ef5513ce0b390a7ab6e4ac.png) No hay solución para u = 0. Tomando la parte "Real", los denominadores nunca son negativos para que puedan restarse y sumarse (ya que solo se suman las fracciones), entonces los numeradores deben ser 0, y eso pasa cuando ![TEX: \[u=\pm 1\]](/tex-image/0a292f3209d0a35d8a0b98c91a33f99a.png) ![TEX: \[e^{ix}=1\;\;\;\vee \;\;\;e^{ix}=-1\]](/tex-image/689c99b640287d987364dc8bed502328.png) ![TEX: \[ix=0\;\;\;\vee \;\;\;ix=i\pi \]<br />](/tex-image/a9942624cc0d2b074fa3095f8e094c2c.png) ![TEX: \[x=2\pi k\;\;\;\vee \;\;\;x=\pi +2\pi k\]](/tex-image/f52b3e3c696f55c34c31137d19ee4c2b.png) ![TEX: \[x=\pi k\]](/tex-image/6e5ae6c4ff71fd8dd9b6ac89cf49ba39.png) k en Z A eso llegué, espero ver la forma más sencilla xD con las identidades y eso... ---------------------------------------- Propongo dos inecuaciones facilines: i) ![TEX: \[2\, sen^{2}(x)\geq sen(x)\]](/tex-image/843ad7c666214dcb7d18168e9929a968.png) ii) ![TEX: \[2\, sen(x)cos(x)-sen(x)+2\,cos(x)-1< 0\]](/tex-image/56c82c3cc2e912441804e6307307ed26.png)
Mensaje modificado por Coto-kun el May 25 2013, 09:37 PM -------------------- |
|
|
|
 May 26 2013, 02:06 AM May 26 2013, 02:06 AM
Publicado:
#65
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 147 Registrado: 11-March 12 Miembro Nº: 102.157 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
La i.
La ii. Propongo: i. Resuelva la ecuacion 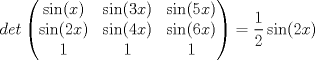 para x en los reales. para x en los reales.Saludos --------------------   "La libertad de uno, termina donde empieza la de otro..." Estudiante de Ingeniería Civil en Mecánica (III año) -> Ayudante de Calculo II 2°sem. 2013 -> Ayudante Ecuaciones diferenciales 1° sem. 2014 Generador de codigo Latex |
|
|
|
| master_c |
 May 26 2013, 11:10 AM May 26 2013, 11:10 AM
Publicado:
#66
|
|
Invitado |
coto-kun te falta una solucion
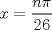 usa el hint >< saludos Mensaje modificado por master_c el May 26 2013, 11:11 AM |
|
|
|
 May 26 2013, 12:17 PM May 26 2013, 12:17 PM
Publicado:
#67
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 146 Registrado: 6-November 09 Desde: El Perla negra Miembro Nº: 61.590 Colegio/Liceo:  |
Usando el hint se tiene:
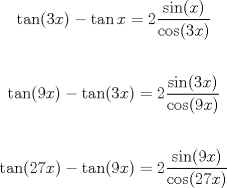 Luego 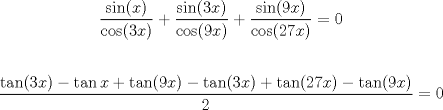 
|
|
|
|
| master_c |
 May 26 2013, 12:26 PM May 26 2013, 12:26 PM
Publicado:
#68
|
|
Invitado |
|
|
|
|
| master_c |
 May 26 2013, 12:27 PM May 26 2013, 12:27 PM
Publicado:
#69
|
|
Invitado |
|
|
|
|
| master_c |
 May 26 2013, 12:38 PM May 26 2013, 12:38 PM
Publicado:
#70
|
|
Invitado |
Propongo
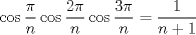
Mensaje modificado por master_c el May 26 2013, 08:19 PM |
|
|
|
  |
3 usuario(s) está(n) leyendo esta discusión (3 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 18th September 2025 - 11:34 AM |








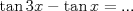


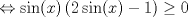
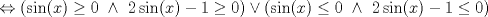
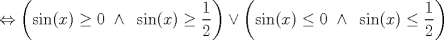
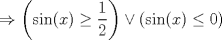
![TEX: $$\Rightarrow\left ( x\in [\frac{\pi}{6}+2\pi k,\frac{5\pi}{6}+2\pi k] \right )\vee \left ( x\in [\pi+2\pi k,2\pi+2\pi k] \right )\textup{ ; }k\in \mathbb{Z}$$](/tex-image/5b7f02fc8b77cf65d15a4742b2e0c184.png)
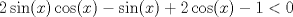
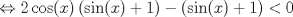
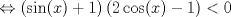
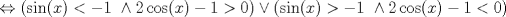
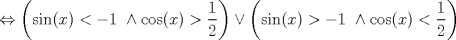
![TEX: $$\Rightarrow\left ( x\in \O \wedge x\in ]a+2\pi k,b+2\pi k[ \right )\vee \left ( x\neq \frac{3\pi}{2}+2\pi k\textup{ } \wedge \textup{ }x\in ]\frac{\pi}{3}+2\pi k,\frac{5\pi}{3}+2\pi k[\right )$$](/tex-image/64880eb46eea6b52807c885f919da38d.png)