ECT-Maratón - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Álgebra > Trigonometría

9 Páginas:

< 1 2 3 4 > »

Reply to this topic

Start new topic

ECT-Maratón, Maratón de ecuaciones trigonométricas

caf_tito Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 06:16 PM Publicado: #11
Dios Matemático Supremo Grupo: Colaborador Silver Mensajes: 1.605 Registrado: 25-June 05 Miembro Nº: 123 Colegio/Liceo: Universidad: Sexo:	bueno... de igual forma se debería especificar entonces que las soluciones que se quieran eran no sólo para ángulos cuadrantes. Me cabecié harto con esta Solución 3 $TEX: \[<br />\begin{gathered}<br /> 4\cos 2x + 3\cos x = 1 \hfill \\<br /> 4\cos \left( {x + x} \right) + 3\cos x = 1 \hfill \\<br /> 4\left( {\cos ^2 x - \sin ^2 x} \right) + 3\cos x = 1 \hfill \\<br /> 4\left( {2\cos ^2 x - 1} \right) + 3\cos x = 1 \hfill \\<br /> 8\cos ^2 x - 4 + 3\cos x = 1 \hfill \\<br /> 8\cos ^2 x + 3\cos x - 5 = 0 \hfill \\<br /> \left( {8\cos x - 5} \right)\left( {\cos x + 1} \right) = 0 \hfill \\<br /> \cos x = \frac{5}<br />{8}{\text{ /}}\arccos {\text{ }}\cos x = - 1{\text{ /}}\arccos \hfill \\<br /> x = \arccos \frac{5}<br />{8} \to x = 360 - \arccos \frac{5}<br />{8} \hfill \\<br /> x = 180 \hfill \\<br /> \boxed{x_1 = \arccos \frac{5}<br />{8} + 360k{\text{ ; }}x_2 = 360 - \arccos \frac{5}<br />{8} + 360k{\text{ ; }}x_3 = 180} \hfill \\ <br />\end{gathered} <br />\]$ Solución 4 $TEX: \[<br />\begin{gathered}<br /> \sin x = \sin 2x \hfill \\<br /> \sin x = 2\sin x\cos x \hfill \\<br /> \sin x = 2\sin x\cos x \hfill \\<br /> \sin x = 2\sin x\sqrt {1 - \sin ^2 x} \hfill \\<br /> \sin ^2 x = 4\sin ^2 x\left( {1 - \sin ^2 x} \right) \hfill \\<br /> \sin ^2 x = 4\sin ^2 x - 4\sin ^4 x \hfill \\<br /> 4\sin ^4 x - 3\sin ^2 x = 0 \hfill \\<br /> \sin ^2 x\left( {4\sin ^2 x - 3} \right) = 0 \hfill \\<br /> \sin ^2 x = 0{\text{ /}}\arcsin {\text{ }}4\sin ^2 x - 3 = 0 \hfill \\<br /> x = 0{\text{ }}\sin ^2 x = \frac{3}<br />{4} \hfill \\<br /> x = 0 \to x = 180;x = 360{\text{ }}\sin x = \pm \frac{{\sqrt 3 }}<br />{2}{\text{ /}}\arcsin \hfill \\<br /> x_1 = 0{\text{ }}x_2 = 180{\text{ }}x_3 = 360{\text{ }}x = \pm 60 \hfill \\<br /> x_1 = 0{\text{ }}x_2 = 180{\text{ }}x_3 = 360{\text{ }}x = 60 \to x = 120{\text{ }}x = - 60 \to x = 240 \wedge x = 30 \hfill \\<br /> x_1 = 0 + 360k{\text{ }}x_2 = 60 + 360k{\text{ }}x_3 = 120 + 360k{\text{ }}x_4 = 180 + 360k;{\text{ }}x_5 = 240 + 360k;{\text{ }}x_6 = 300 + 360k{\text{ ;}}x_7 = 360 + 360k \hfill \\ <br />\end{gathered} <br />\]$ Mensaje modificado por caf_tito el Dec 5 2006, 06:49 PM --------------------

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 06:25 PM Publicado: #12
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	Bueno, se están trabajando con ángulos positivos para mayor comodidad y estética. Otra cosa $TEX: $\tan (180^\circ + \alpha ) = \tan \alpha$$ $TEX: $\cos (360^\circ - \alpha ) = \cos \alpha$$ CITA se debería especificar entonces que las soluciones que se quieran eran no sólo para ángulos cuadrantes Solamente se piden expresadas positivas. olvidé añadir eso. CITA(caf_tito @ Dec 5 2006, 08:16 PM) Solución 4 $TEX: \[<br />\begin{gathered}<br /> \sin x = \sin 2x \hfill \\<br /> \sin x = 2\sin x\cos x \hfill \\<br /> \sin x = 2\sin x\sqrt {1 - \sin ^2 x} \hfill \\<br /> \sin ^2 x = 4\sin ^2 x\left( {1 - \sin ^2 x} \right) \hfill \\<br /> 1 = 4 - 4\sin ^2 x \hfill \\<br /> 4\sin ^2 x = 3 \hfill \\<br /> \sin x = \pm \frac{{\sqrt 3 }}<br />{2}{\text{ arcsin}} \hfill \\<br /> x = 60 \hfill \\<br /> x_1 = 360k + 60{\text{ }}x_2 = 360k + 120 \hfill \\ <br />\end{gathered} <br />\]$ Hay un paso fatal ahí al dividir por seno...

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 06:44 PM Publicado: #13
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	CITA(caf_tito @ Dec 5 2006, 08:16 PM) Solución 3 $TEX: \[<br />\begin{gathered}<br /> 4\cos 2x + 3\cos x = 1 \hfill \\<br /> 4\cos \left( {x + x} \right) + 3\cos x = 1 \hfill \\<br /> 4\left( {\cos ^2 x - \sin ^2 x} \right) + 3\cos x = 1 \hfill \\<br /> 4\left( {2\cos ^2 x - 1} \right) + 3\cos x = 1 \hfill \\<br /> 8\cos ^2 x - 4 + 3\cos x = 1 \hfill \\<br /> 8\cos ^2 x + 3\cos x - 5 = 0 \hfill \\<br /> \left( {8\cos x - 5} \right)\left( {\cos x + 1} \right) = 0 \hfill \\<br /> \cos x = \frac{5}<br />{8}{\text{ /}}\arccos {\text{ }}\cos x = - 1{\text{ /}}\arccos \hfill \\<br /> x = \arccos \frac{5}<br />{8} \to x = 360 - \arccos \frac{5}<br />{8} \hfill \\<br /> x = 180 \hfill \\<br /> \boxed{x_1 = \arccos \frac{5}<br />{8} + 360k{\text{ ; }}x_2 = 360 - \arccos \frac{5}<br />{8} + 360k{\text{ ; }}x_3 = 180} \hfill \\ <br />\end{gathered} <br />\]$ Solución correcta P.D.: no sea flojo y exprese la solución con grados y minutos si es necesario (solamente para que se vea "más mejor"; ya sé, no está especificado )

caf_tito Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 07:01 PM Publicado: #14
Dios Matemático Supremo Grupo: Colaborador Silver Mensajes: 1.605 Registrado: 25-June 05 Miembro Nº: 123 Colegio/Liceo: Universidad: Sexo:	Solución 7 $TEX: \[<br />\begin{gathered}<br /> \cos x + \cos 2x + \cos 3x = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \cos \left( {2x + x} \right) = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \cos 2x\cos x + \sin 2x\sin x = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \left( {\cos ^2 x - \sin ^2 x} \right)\cos x + 2\sin x\cos x\sin x = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \cos ^3 x - \sin ^2 x\cos x + 2\sin ^2 x\cos x = 0 \hfill \\<br /> \cos x^3 + \cos ^2 x + \sin ^2 x\cos x + \cos x = 0 \hfill \\<br /> \cos x^3 + \cos ^2 x + \left( {1 - \cos ^2 x} \right)\cos x + \cos x = 0 \hfill \\<br /> \cos x^3 + \cos ^2 x + \cos x - \cos ^3 x + \cos x = 0 \hfill \\<br /> \cos ^2 x + 2\cos x = 0 \hfill \\<br /> \cos x\left( {\cos x + 2} \right) = 0 \hfill \\<br /> \cos x = 0{\text{ /}}\arccos {\text{ }} \wedge \cos x = - 2{\text{ /}}\arccos \hfill \\<br /> x_1 = 90;{\text{ }}x_2 = 270{\text{ }}x = {\text{ no se define para negativos}} \hfill \\<br /> \boxed{x_1 = 90;{\text{ }}x_2 = 270} \hfill \\ <br />\end{gathered} <br />\]$ Solución 8 $TEX: \[<br />\begin{gathered}<br /> 2\cos x = 1 - \sin x \hfill \\<br /> 2\sqrt {1 - \sin ^2 x} = 1 - \sin x \hfill \\<br /> 4\left( {1 - \sin ^2 x} \right) = 1 - 2\sin x + \sin ^2 x \hfill \\<br /> 4 - 4\sin ^2 x = 1 - 2\sin x + \sin ^2 x \hfill \\<br /> 5\sin ^2 x - 2\sin x - 3 = 0 \hfill \\<br /> \left( {5\sin x + 3} \right)\left( {\sin x - 1} \right) = 0 \hfill \\<br /> \sin x = \frac{{ - 3}}<br />{5}{\text{ /}}\arcsin {\text{ }}\sin x = 1{\text{ /}}\arcsin \hfill \\<br /> x = \frac{{ - 3}}<br />{5}\arcsin {\text{ }}x = 90 \hfill \\<br /> x_1 = \frac{{ - 3}}<br />{5}\arcsin + 180{\text{ }} + 360k \hfill \\<br /> x_2 = 360 - \frac{{ - 3}}<br />{5}\arcsin + 360k \hfill \\<br /> x_3 = 90 + 360k \hfill \\ <br />\end{gathered} <br />\]$ Mensaje modificado por caf_tito el Dec 5 2006, 07:15 PM --------------------

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 07:45 PM Publicado: #15
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	CITA(caf_tito @ Dec 5 2006, 09:01 PM) Solución 7 $TEX: \[<br />\begin{gathered}<br /> \cos x + \cos 2x + \cos 3x = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \cos \left( {2x + x} \right) = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \cos 2x\cos x + \sin 2x\sin x = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \left( {\cos ^2 x - \sin ^2 x} \right)\cos x + 2\sin x\cos x\sin x = 0 \hfill \\<br /> \cos x + \cos ^2 x - \sin ^2 x + \cos ^3 x - \sin ^2 x\cos x + 2\sin ^2 x\cos x = 0 \hfill \\<br /> \cos x^3 + \cos ^2 x + \sin ^2 x\cos x + \cos x = 0 \hfill \\<br /> \cos x^3 + \cos ^2 x + \left( {1 - \cos ^2 x} \right)\cos x + \cos x = 0 \hfill \\<br /> \cos x^3 + \cos ^2 x + \cos x - \cos ^3 x + \cos x = 0 \hfill \\<br /> \cos ^2 x + 2\cos x = 0 \hfill \\<br /> \cos x\left( {\cos x + 2} \right) = 0 \hfill \\<br /> \cos x = 0{\text{ /}}\arccos {\text{ }} \wedge \cos x = - 2{\text{ /}}\arccos \hfill \\<br /> x_1 = 90;{\text{ }}x_2 = 270{\text{ }}x = {\text{ no se define para negativos}} \hfill \\<br /> \boxed{x_1 = 90;{\text{ }}x_2 = 270} \hfill \\ <br />\end{gathered} <br />\]$ Recomiendo usar 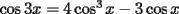$TEX: $\cos 3x = 4\cos ^3 x - 3\cos x$$ CITA(caf_tito @ Dec 5 2006, 09:01 PM) b]Solución 8[/b] $TEX: \[<br />\begin{gathered}<br /> 2\cos x = 1 - \sin x \hfill \\<br /> 2\sqrt {1 - \sin ^2 x} = 1 - \sin x \hfill \\<br /> 4\left( {1 - \sin ^2 x} \right) = 1 - 2\sin x + \sin ^2 x \hfill \\<br /> 4 - 4\sin ^2 x = 1 - 2\sin x + \sin ^2 x \hfill \\<br /> 5\sin ^2 x - 2\sin x - 3 = 0 \hfill \\<br /> \left( {5\sin x + 3} \right)\left( {\sin x - 1} \right) = 0 \hfill \\<br /> \sin x = \frac{{ - 3}}<br />{5}{\text{ /}}\arcsin {\text{ }}\sin x = 1{\text{ /}}\arcsin \hfill \\<br /> x = \frac{{ - 3}}<br />{5}\arcsin {\text{ }}x = 90 \hfill \\<br /> x_1 = \frac{{ - 3}}<br />{5}\arcsin + 180{\text{ }} + 360k \hfill \\<br /> x_2 = 360 - \frac{{ - 3}}<br />{5}\arcsin + 360k \hfill \\<br /> x_3 = 90 + 360k \hfill \\ <br />\end{gathered} <br />\]$ Se ruegan escribir un poco más ordenada las raíces obtenidas, porque se ven cosas que están mal escritas y algunas no se entienden (No va en mala onda, y creo que ya está todo especificado en el 1er. post. Todo esto es con armonía )

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 9 2006, 08:45 AM Publicado: #16
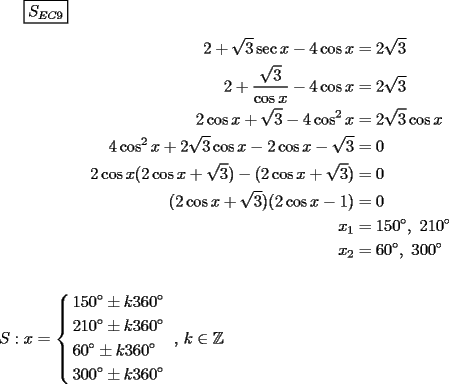
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	$TEX: $\boxed{S_{EC9} }$<br /><br />\begin{equation}<br />\begin{aligned}<br /> 2 + \sqrt 3 \sec x - 4\cos x &= 2\sqrt 3 \\ <br /> 2 + \frac{{\sqrt 3 }}<br />{{\cos x}} - 4\cos x &= 2\sqrt 3 \\ <br /> 2\cos x + \sqrt 3 - 4\cos ^2 x &= 2\sqrt 3 \cos x \\ <br /> 4\cos ^2 x + 2\sqrt 3 \cos x - 2\cos x - \sqrt 3 &= 0 \\ <br /> 2\cos x(2\cos x + \sqrt 3 ) - (2\cos x + \sqrt 3 ) &= 0 \\ <br /> (2\cos x + \sqrt 3 )(2\cos x - 1) &= 0 \\ <br /> x_1 &= 150^\circ ,{\text{ }}210^\circ \\ <br /> x_2 &= 60^\circ ,{\text{ }}300^\circ \\ <br />\end{aligned}<br />\end{equation}\\<br />\\<br />$S:x = \left\{ \begin{gathered}<br /> 150^\circ \pm k360^\circ \hfill \\<br /> 210^\circ \pm k360^\circ \hfill \\<br /> 60^\circ \pm k360^\circ \hfill \\<br /> 300^\circ \pm k360^\circ \hfill \\ <br />\end{gathered} \right.{\text{ }}{\text{, }}k \in \mathbb{Z}$$

Jorgeston Ver Perfil del Miembro Ver los mensajes de este miembro	May 29 2007, 04:43 PM Publicado: #17
Dios Matemático Supremo Grupo: Baneado Mensajes: 2.588 Registrado: 7-November 06 Miembro Nº: 2.747	Me parece interesante y entretenida una ECT maraton. Ojala se interesen mas usuarios, y nos pongamos a resolver ECT como locos. saludos A destacados.

Jorgeston Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 3 2007, 10:42 PM Publicado: #18
Dios Matemático Supremo Grupo: Baneado Mensajes: 2.588 Registrado: 7-November 06 Miembro Nº: 2.747	Ya, re-empezemos de nuevo la ECT maraton ejej: Resuelva: 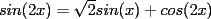$TEX: $sin(2x)=\sqrt{2}sin(x)+cos(2x)$$ saludos

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 26 2008, 02:28 PM Publicado: #19
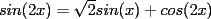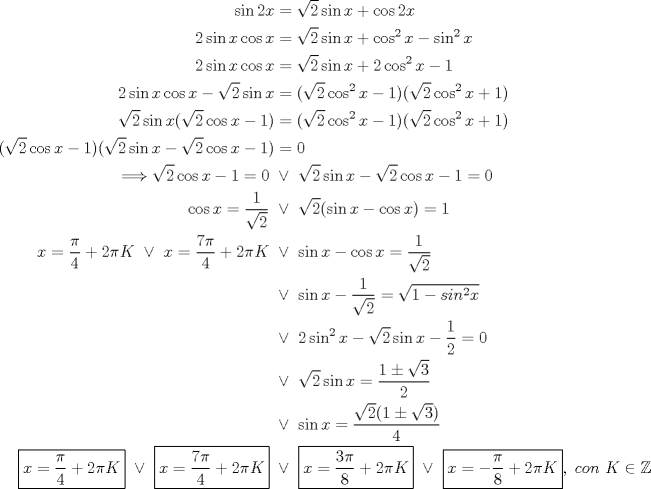
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(jorgeston @ Jun 4 2007, 01:42 AM) Ya, re-empezemos de nuevo la ECT maraton ejej: Resuelva: $TEX: $sin(2x)=\sqrt{2}sin(x)+cos(2x)$$ saludos $TEX: \begin{equation}\begin{aligned}<br />\sin{2x}&=\sqrt{2}\sin{x}+\cos{2x}\\<br />2\sin{x}\cos{x}&=\sqrt{2}\sin{x}+\cos^2{x}-\sin^2{x}\\<br />2\sin{x}\cos{x}&=\sqrt{2}\sin{x}+2\cos^2{x}-1\\<br />2\sin{x}\cos{x}-\sqrt{2}\sin{x}&=(\sqrt{2}\cos^2{x}-1)(\sqrt{2}\cos^2{x}+1)\\<br />\sqrt{2}\sin{x}(\sqrt{2}\cos{x}-1)&=(\sqrt{2}\cos^2{x}-1)(\sqrt{2}\cos^2{x}+1)\\<br />(\sqrt{2}\cos{x}-1)(\sqrt{2}\sin{x}-\sqrt{2}\cos{x}-1)&=0\\<br />\Longrightarrow \sqrt{2}\cos{x}-1=0 \ &\lor \ \sqrt{2}\sin{x}-\sqrt{2}\cos{x}-1=0\\<br />\cos{x}=\dfrac{1}{\sqrt{2}} \ &\lor \ \sqrt{2}(\sin{x}-\cos{x})=1\\<br />x=\dfrac{\pi}{4}+2\pi K \ \lor \ x=\dfrac{7\pi}{4}+2\pi K \ &\lor \ \sin{x}-\cos{x}=\dfrac{1}{\sqrt{2}}\\<br />&\lor \ \sin{x}-\dfrac{1}{\sqrt{2}} = \sqrt{1-sin^2{x}}\\<br />&\lor \ 2\sin^2{x}-\sqrt{2}\sin{x}-\dfrac{1}{2}=0\\<br />&\lor \ \sqrt{2}\sin{x}=\dfrac{1\pm\sqrt{3}}{2}\\<br />&\lor \ \sin{x}=\dfrac{\sqrt{2}(1\pm\sqrt{3})}{4}\\<br />\boxed{x=\dfrac{\pi}{4}+2\pi K} \ \lor \ \boxed{x=\dfrac{7\pi}{4}+2\pi K} \ &\lor \ \boxed{x=\dfrac{3\pi}{8}+2\pi K} \ \lor \ \boxed{x=-\dfrac{\pi}{8}+2\pi K},\ con\ K\in\mathbb{Z}\\<br />\end{aligned}\end{equation}$ -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

camileis Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 15 2008, 03:42 PM Publicado: #20
Dios Matemático Grupo: Usuario FMAT Mensajes: 296 Registrado: 26-May 08 Miembro Nº: 24.633 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	-------------------- ex-ingenieria en biotecnologia -USACH 2008 ahora fonoaudiologia -UCH 2009

« Posterior · Trigonometría · Anterior »

9 Páginas:

< 1 2 3 4 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 2nd April 2025 - 09:42 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.