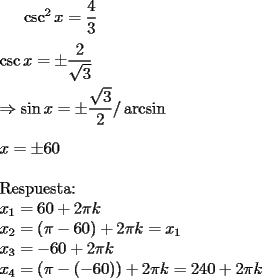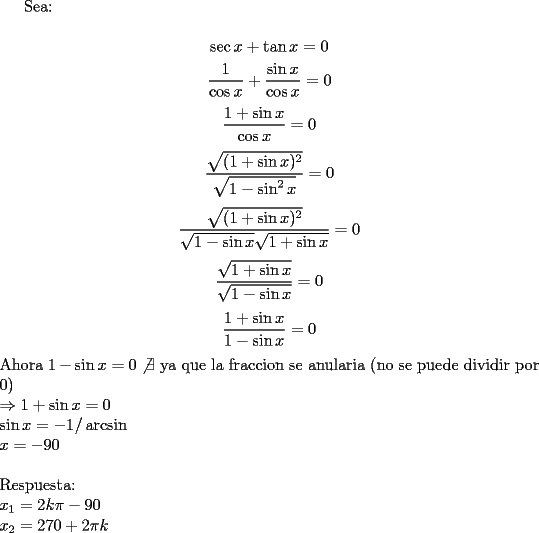ECT-Maratón - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Álgebra > Trigonometría

9 Páginas:

1 2 3 > »

Reply to this topic

Start new topic

ECT-Maratón, Maratón de ecuaciones trigonométricas

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 03:53 PM Publicado: #1
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	Cómo dejar de lado a las interestresantes ecuaciones trigonométricas. Bueno, si nadie Autoritario se opone. Esto consiste en que primero se colocará una serie de ecuaciones en donde se irán resolviendo en un orden cualquiera (también aceptando soluciones alternas las cuales puedan facilitar un desarrollo más conciso a la ecuación). Posterior a ello, el usuario que resuelva la ecuación de proponer otra y así sucesivamente (este proceso se inicia cuando hayan sido resueltas las 1eras. 9 ecuaciones propuestas). * Se piden las soluciones expresadas en ángulos positivos. Cuando sea necesario, expresar la raíz en grados y minutos. La idea es que se lleguen a unas 44 ecuaciones y ojalá este topic tenga buena recepción Empezamos Hallar el conjunto solución a las siguientes ecuaciones: $TEX: \[<br />\begin{gathered}<br /> \boxed{Ec. - 1}{\text{ }}4\operatorname{sen} ^2 x \cdot \tan x - 4\operatorname{sen} ^2 x - 3\tan x + 3 = 0 \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 2}{\text{ }}\csc x + \cot x = \sqrt 3 \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 3}{\text{ }}4\cos 2x + 3\cos x = 1 \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 4}{\text{ }}\operatorname{sen} x = \operatorname{sen} 2x \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 5}{\text{ }}\csc ^2 x = \frac{4}<br />{3} \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 6}{\text{ }}\sec x + \tan x = 0 \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 7}{\text{ }}\cos x + \cos 2x + \cos 3x = 0 \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 8}{\text{ }}2\cos x = 1 - \operatorname{sen} x \hfill \\<br /> \hfill \\<br /> \boxed{Ec. - 9}{\text{ }}2 + \sqrt 3 \sec x - 4\cos x = 2\sqrt 3 \hfill \\ <br />\end{gathered} <br />\]$ Mensaje modificado por Krizalid el Dec 5 2006, 06:45 PM

caf_tito Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 04:43 PM Publicado: #2
Dios Matemático Supremo Grupo: Colaborador Silver Mensajes: 1.605 Registrado: 25-June 05 Miembro Nº: 123 Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> 4\sin ^2 x\tan x - 4\sin ^2 x - 3\tan x + 3 = 0 \hfill \\<br /> 4\sin ^2 x\left( {\tan x - 1} \right) - 3\left( {\tan x - 1} \right) = 0 \hfill \\<br /> \left( {4\sin ^2 x - 3} \right)\left( {\tan x - 1} \right) = 0 \hfill \\<br /> 4\sin ^2 x - 3 = 0{\text{ }}\tan x - 1 = 0 \hfill \\<br /> \sin ^2 x = \frac{3}<br />{4}{\text{ }}\tan x = 1{\text{ /arctan}} \hfill \\<br /> \sin x = \pm \frac{{\sqrt 3 }}<br />{2}{\text{ /}}\arcsin {\text{ }}x_1 = 45 \hfill \\<br /> x = \pm 60 \hfill \\<br /> \hfill \\<br /> \boxed{x_1 = 45{\text{ }}x_2 = 60{\text{ }}x_3 = - 60} \hfill \\ <br />\end{gathered} <br />\]$ Haciendo casi por priemra vez en mi vida una ecuación Trigonométrica Edit: $TEX: \[<br />\begin{gathered}<br /> {\text{Aplicando la tablita de Todas Sin ta cos}} \hfill \\<br /> x_2 = 60 \to x = 120 \hfill \\<br /> x_3 = - 60 \to x = 240 \wedge x = 300 \hfill \\<br /> x_1 = 45 \to x = 225 \hfill \\<br /> {\text{Finalmente tomando los valores positivos tendremos}} \hfill \\<br /> \boxed{x_1 = 45{\text{ ; }}x_2 = 60{\text{ ;}}x_3 = 225{\text{ ; }}x_4 = 120{\text{; }}x_5 = 240{\text{ ; }}x_6 = 300} \hfill \\ <br />\end{gathered} <br />\]$ Mensaje modificado por caf_tito el Dec 5 2006, 05:01 PM --------------------

caf_tito Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 04:51 PM Publicado: #3
Dios Matemático Supremo Grupo: Colaborador Silver Mensajes: 1.605 Registrado: 25-June 05 Miembro Nº: 123 Colegio/Liceo: Universidad: Sexo:	Esperando que esté bien $TEX: \[<br />\boxed{{\text{S}}_{{\text{ecuacion 2}}} }<br />\]$ $TEX: \[<br />\begin{gathered}<br /> \csc x + \cot x = \sqrt 3 \hfill \\<br /> \frac{1}<br />{{\sin x}} + \frac{{\cos x}}<br />{{\sin x}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{\sin x}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{\sqrt {1 - \cos ^2 x} }} = \sqrt 3 \hfill \\<br /> \frac{{\sqrt {1 + \cos x} \sqrt {1 + \cos x} }}<br />{{\sqrt {1 + \cos x} \sqrt {1 - \cos x} }} = \sqrt 3 \hfill \\<br /> \sqrt {\frac{{1 + \cos x}}<br />{{1 - \cos x}}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{1 - \cos x}} = 3 \hfill \\<br /> 1 + \cos x = 3 - 3\cos x \hfill \\<br /> 4\cos x = 2 \hfill \\<br /> \cos x = \frac{1}<br />{2}{\text{ /}}\arccos \hfill \\<br /> x = 60 \hfill \\<br /> \boxed{x_1 = 60{\text{ }}x_2 = 300} \hfill \\ <br />\end{gathered} <br />\]$ P.S: Me había faltado multiplicar por 3 el coseno al final. Mensaje modificado por caf_tito el Dec 5 2006, 05:13 PM --------------------

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 04:52 PM Publicado: #4
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	CITA(caf_tito @ Dec 5 2006, 06:43 PM) $TEX: $\boxed{x_1 = 45{\text{ }}x_2 = 60{\text{ }}x_3 = - 60}$$ Haciendo casi por priemra vez en mi vida una ecuación Trigonométrica Falta llevar a ángulo positivo la 3era. raíz y aún quedan tres soluciones más para esa ecuación Saludos P.D.: no está mal para la 1era. vez

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 04:55 PM Publicado: #5
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	CITA(caf_tito @ Dec 5 2006, 06:51 PM) Esperando que esté bien $TEX: \[<br />\boxed{{\text{S}}_{{\text{ecuacion 2}}} }<br />\]$ $TEX: \[<br />\begin{gathered}<br /> \csc x + \cot x = \sqrt 3 \hfill \\<br /> \frac{1}<br />{{\sin x}} + \frac{{\cos x}}<br />{{\sin x}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{\sin x}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{\sqrt {\left( {1 - \cos ^2 x} \right)} }} = \sqrt 3 \hfill \\<br /> \frac{{\sqrt {\left( {1 + \cos x} \right)} \sqrt {\left( {1 + \cos x} \right)} }}<br />{{\sqrt {\left( {1 + \cos x} \right)} \sqrt {\left( {1 - \cos x} \right)} }} = \sqrt 3 \hfill \\<br /> \frac{{\sqrt {\left( {1 + \cos x} \right)} }}<br />{{\sqrt {\left( {1 - \cos x} \right)} }} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{1 - \cos x}} = 3 \hfill \\<br /> 1 + \cos x = 3 - \cos x \hfill \\<br /> 2\cos x = 2 \hfill \\<br /> \cos x = 1{\text{ /}}\arccos \hfill \\<br /> x = 0 \hfill \\ <br />\end{gathered} <br />\]$ Respuesta incorrecta Hint: Como consejo, eleva al cuadrado la ecuación desde el 3er. paso. Saludos

Zirou Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 05:34 PM Publicado: #6
Máquina que convierte café en teoremas Grupo: Colaborador Silver Mensajes: 1.665 Registrado: 18-August 05 Desde: Concepción Miembro Nº: 247 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	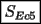$TEX: $\boxed{S_{Ec5}Editado}$$ $TEX: $\csc^2x=\dfrac{4}{3}$\\<br />\\<br />$\csc x=\pm\dfrac{2}{\sqrt{3}}$\\<br />\\<br />$\Rightarrow \sin x=\pm\dfrac{\sqrt{3}}{2} /\arcsin$\\<br />\\<br />$x=\pm60$\\<br />\\<br />Respuesta:\\<br />$x_1=60+2\pi k$\\<br />$x_2=(\pi -60)+2\pi k=x_1$\\<br />$x_3=-60+2\pi k$\\<br />$x_4=(\pi -(-60))+2\pi k=240+2\pi k$<br />$ Mensaje modificado por zirou el Dec 5 2006, 06:17 PM -------------------- $TEX: $mathcal{Z}$ $imath$ $Re$ $varnothing$ $mho$$ Manual para subir imágenes y archivos a fmat (con servidor propio) Manual de latex Estilo Propio Lista de libros en fmat "Un Matemático es una máquina que trasforma café en teoremas"(Erdös) --- Consultas, sugerencias, reclamos via mp o a los correos mencionados.

Zirou Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 05:54 PM Publicado: #7
Máquina que convierte café en teoremas Grupo: Colaborador Silver Mensajes: 1.665 Registrado: 18-August 05 Desde: Concepción Miembro Nº: 247 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	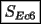$TEX: $\boxed{S_{Ec6}}$$ $TEX: Sea:\\<br />$$\sec x+\tan x=0$$<br />$$\dfrac{1}{\cos x}+\dfrac{\sin x}{\cos x}=0$$<br />$$\dfrac{1+\sin x}{\cos x}=0$$<br />$$\dfrac{\sqrt{(1+\sin x)^2}}{\sqrt{1-\sin^2 x}}=0$$<br />$$\dfrac{\sqrt{(1+\sin x)^2}}{\sqrt{1-\sin x}\sqrt{1+\sin x}}=0$$<br />$$\dfrac{\sqrt{1+\sin x}}{\sqrt{1-\sin x}}=0$$<br />$$\dfrac{1+\sin x}{1-\sin x}=0$$<br />Ahora $1- \sin x=0 \not \exists$ ya que la fraccion se anularia (no se puede dividir por 0)\\<br />$\Rightarrow 1+\sin x=0$\\<br />$\sin x=-1 /\arcsin$\\<br />$x=-90$\\<br />\\<br />Respuesta:\\<br />$x_1=2k\pi-90$\\<br />$x_2=270+2\pi k$\\<br />$ Mensaje modificado por zirou el Dec 5 2006, 06:21 PM -------------------- $TEX: $mathcal{Z}$ $imath$ $Re$ $varnothing$ $mho$$ Manual para subir imágenes y archivos a fmat (con servidor propio) Manual de latex Estilo Propio Lista de libros en fmat "Un Matemático es una máquina que trasforma café en teoremas"(Erdös) --- Consultas, sugerencias, reclamos via mp o a los correos mencionados.

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 05:58 PM Publicado: #8
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	CITA(caf_tito @ Dec 5 2006, 06:43 PM) $TEX: \[<br />\begin{gathered}<br /> 4\sin ^2 x\tan x - 4\sin ^2 x - 3\tan x + 3 = 0 \hfill \\<br /> 4\sin ^2 x\left( {\tan x - 1} \right) - 3\left( {\tan x - 1} \right) = 0 \hfill \\<br /> \left( {4\sin ^2 x - 3} \right)\left( {\tan x - 1} \right) = 0 \hfill \\<br /> 4\sin ^2 x - 3 = 0{\text{ }}\tan x - 1 = 0 \hfill \\<br /> \sin ^2 x = \frac{3}<br />{4}{\text{ }}\tan x = 1{\text{ /arctan}} \hfill \\<br /> \sin x = \pm \frac{{\sqrt 3 }}<br />{2}{\text{ /}}\arcsin {\text{ }}x_1 = 45 \hfill \\<br /> x = \pm 60 \hfill \\<br /> \hfill \\<br /> \boxed{x_1 = 45{\text{ }}x_2 = 60{\text{ }}x_3 = - 60} \hfill \\ <br />\end{gathered} <br />\]$ Haciendo casi por priemra vez en mi vida una ecuación Trigonométrica Edit: $TEX: \[<br />\begin{gathered}<br /> {\text{Aplicando la tablita de Todas Sin ta cos}} \hfill \\<br /> x_2 = 60 \to x = 120 \hfill \\<br /> x_3 = - 60 \to x = 240 \wedge x = 300 \hfill \\<br /> x_1 = 45 \to x = 225 \hfill \\<br /> {\text{Finalmente tomando los valores positivos tendremos}} \hfill \\<br /> \boxed{x_1 = 45{\text{ ; }}x_2 = 60{\text{ ;}}x_3 = 225{\text{ ; }}x_4 = 120{\text{; }}x_5 = 240{\text{ ; }}x_6 = 300} \hfill \\ <br />\end{gathered} <br />\]$ Solución correcta (recordar anteponer un 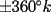$TEX: $\pm 360^\circ k$$ , con $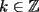TEX: $k \in \mathbb{Z}$$ en cada solución). CITA(caf_tito @ Dec 5 2006, 06:51 PM) Esperando que esté bien $TEX: \[<br />\boxed{{\text{S}}_{{\text{ecuacion 2}}} }<br />\]$ $TEX: \[<br />\begin{gathered}<br /> \csc x + \cot x = \sqrt 3 \hfill \\<br /> \frac{1}<br />{{\sin x}} + \frac{{\cos x}}<br />{{\sin x}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{\sin x}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{\sqrt {1 - \cos ^2 x} }} = \sqrt 3 \hfill \\<br /> \frac{{\sqrt {1 + \cos x} \sqrt {1 + \cos x} }}<br />{{\sqrt {1 + \cos x} \sqrt {1 - \cos x} }} = \sqrt 3 \hfill \\<br /> \sqrt {\frac{{1 + \cos x}}<br />{{1 - \cos x}}} = \sqrt 3 \hfill \\<br /> \frac{{1 + \cos x}}<br />{{1 - \cos x}} = 3 \hfill \\<br /> 1 + \cos x = 3 - 3\cos x \hfill \\<br /> 4\cos x = 2 \hfill \\<br /> \cos x = \frac{1}<br />{2}{\text{ /}}\arccos \hfill \\<br /> x = 60 \hfill \\<br /> \boxed{x_1 = 60{\text{ }}x_2 = 300} \hfill \\ <br />\end{gathered} <br />\]$ Acá hay un leve detalle con las soluciones, solamente una es correcta (recordar que se pide el conjunto solución). Saludos Mensaje modificado por Krizalid el Dec 5 2006, 05:58 PM

「Krizalid」 Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 06:08 PM Publicado: #9
Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad: Sexo:	CITA(zirou @ Dec 5 2006, 07:34 PM) 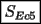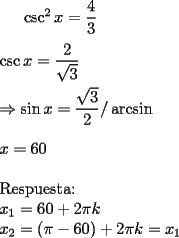$TEX: $\boxed{S_{Ec5}}$$ $TEX: $\csc^2x=\dfrac{4}{3}$\\<br />\\<br />$\csc x=\dfrac{2}{\sqrt{3}}$\\<br />\\<br />$\Rightarrow \sin x=\dfrac{\sqrt{3}}{2} /\arcsin$\\<br />\\<br />$x=60$\\<br />\\<br />Respuesta:\\<br />$x_1=60+2\pi k$\\<br />$x_2=(\pi -60)+2\pi k=x_1$$ Acá faltan dos soluciones más CITA(zirou @ Dec 5 2006, 07:54 PM) 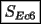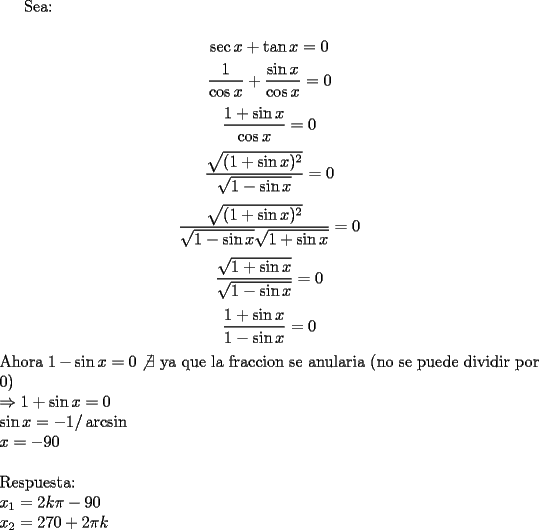$TEX: $\boxed{S_{Ec6}}$$ $TEX: Sea:\\<br />$$\sec x+\tan x=0$$<br />$$\dfrac{1}{\cos x}+\dfrac{\sin x}{\cos x}=0$$<br />$$\dfrac{1+\sin x}{\cos x}=0$$<br />$$\dfrac{\sqrt{(1+\sin x)^2}}{\sqrt{1-\sin x}}=0$$<br />$$\dfrac{\sqrt{(1+\sin x)^2}}{\sqrt{1-\sin x}\sqrt{1+\sin x}}=0$$<br />$$\dfrac{\sqrt{1+\sin x}}{\sqrt{1-\sin x}}=0$$<br />$$\dfrac{1+\sin x}{1-\sin x}=0$$<br />Ahora $1- \sin x=0 \not \exists$ ya que la fraccion se anularia (no se puede dividir por 0)\\<br />$\Rightarrow 1+\sin x=0$\\<br />$\sin x=-1 /\arcsin$\\<br />$x=-90$\\<br />\\<br />Respuesta:\\<br />$x_1=2k\pi-90$\\<br />$x_2=270+2\pi k$<br />$ Recordar que la secante y la tangente de 270° no están definidas No se entiende la respuesta de la 1era. raíz

Zirou Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2006, 06:09 PM Publicado: #10
Máquina que convierte café en teoremas Grupo: Colaborador Silver Mensajes: 1.665 Registrado: 18-August 05 Desde: Concepción Miembro Nº: 247 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Solo recordar que las soluciones deben ir en una forma general y no solo los 2 o 3 valores que nos de la calculadora: Para Seno 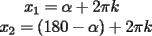$TEX: $x_1=\alpha+2\pi k$\\<br />$x_2=(180-\alpha)+2\pi k$$ Para Coseno 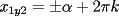$TEX: $x_{1y2}=\pm\alpha+2\pi k$$ Para Tangente 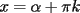$TEX: $x=\alpha+\pi k$$ -------------------- $TEX: $mathcal{Z}$ $imath$ $Re$ $varnothing$ $mho$$ Manual para subir imágenes y archivos a fmat (con servidor propio) Manual de latex Estilo Propio Lista de libros en fmat "Un Matemático es una máquina que trasforma café en teoremas"(Erdös) --- Consultas, sugerencias, reclamos via mp o a los correos mencionados.

« Posterior · Trigonometría · Anterior »

9 Páginas:

1 2 3 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 26th April 2025 - 12:03 AM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.