Trigonometria - Foro fmat.cl

Foro fmat.cl > Programa Enseñanza Universitaria > Consultas > Banco de Problemas Resueltos > Álgebra > Trigonometría

Trigonometria, Fácil, a lucirse mechón, novato, cachorro...

Opciones

danielomalmsteen Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 20 2010, 01:51 PM Publicado: #1
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.413 Registrado: 13-March 08 Desde: Al frente del mundo Magico Miembro Nº: 16.846 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Pruebe que : 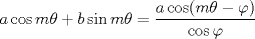$TEX: $a\cos{m\theta} + b\sin{m\theta} = \dfrac{a\cos({m\theta - \varphi)}}{\cos\varphi}$$ donde 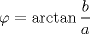$TEX: $\varphi = \arctan\dfrac{b}{a}$$ con $TEX: $a$$ y $TEX: $b$$ positivos editado xd Mensaje modificado por danielomalmsteen el Feb 20 2010, 02:21 PM --------------------

felper Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 20 2010, 02:13 PM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.767 Registrado: 21-January 08 Desde: Santiago - Ancud Miembro Nº: 14.865 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Mmmm, es idea mía, o debería ser 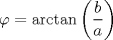$TEX: $\varphi=\arctan\left(\dfrac{b}{a} \right)$$ ? -------------------- Estudia para superarte a ti mismo, no al resto.

Manuel Fco Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 20 2010, 02:21 PM Publicado: #3
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.077 Registrado: 19-December 07 Desde: Santiago, RM. Miembro Nº: 13.971 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> \phi = \arctan \frac{b}<br />{a} \hfill \\<br /> tg\phi = \frac{b}<br />{a} \hfill \\<br /> tg\phi = \frac{{sen\phi }}<br />{{\cos \phi }} = \frac{b}<br />{a} \hfill \\<br /> \Rightarrow a = \frac{{\cos \phi \cdot b}}<br />{{sen\phi }} \wedge b = \frac{{sen\phi \cdot a}}<br />{{\cos \phi }} \hfill \\<br /> \cos (m\theta - \phi ) = \cos m\theta \cdot \cos \phi + senm\theta \cdot sen\phi \hfill \\<br /> a\cos (m\theta - \phi ) = a\cos m\theta \cdot \cos \phi + asenm\theta \cdot sen\phi \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = \frac{{a \cdot \cos m\theta \cdot \cos \phi + a \cdot senm\theta \cdot sen\phi }}<br />{{\cos \phi }} \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = a\cos m\theta + a \cdot senm\theta \cdot tg\phi \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = a\cos m\theta + \frac{{\cos \phi \cdot b}}<br />{{sen\phi }} \cdot senm\theta \cdot \frac{{sen\phi }}<br />{{\cos \phi }} \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = a\cos m\theta + b \cdot senm\theta \hfill \\ <br />\end{gathered} <br />\]$ Con razón no me salía xd, saludos daniel !

danielomalmsteen Ver Perfil del Miembro Ver los mensajes de este miembro	Feb 20 2010, 02:22 PM Publicado: #4
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.413 Registrado: 13-March 08 Desde: Al frente del mundo Magico Miembro Nº: 16.846 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(felper @ Feb 20 2010, 03:13 PM) Mmmm, es idea mía, o debería ser 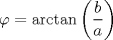$TEX: $\varphi=\arctan\left(\dfrac{b}{a} \right)$$ ? CITA(Manuel Fco @ Feb 20 2010, 03:21 PM) $TEX: \[<br />\begin{gathered}<br /> \phi = \arctan \frac{b}<br />{a} \hfill \\<br /> tg\phi = \frac{b}<br />{a} \hfill \\<br /> tg\phi = \frac{{sen\phi }}<br />{{\cos \phi }} = \frac{b}<br />{a} \hfill \\<br /> \Rightarrow a = \frac{{\cos \phi \cdot b}}<br />{{sen\phi }} \wedge b = \frac{{sen\phi \cdot a}}<br />{{\cos \phi }} \hfill \\<br /> \cos (m\theta - \phi ) = \cos m\theta \cdot \cos \phi + senm\theta \cdot sen\phi \hfill \\<br /> a\cos (m\theta - \phi ) = a\cos m\theta \cdot \cos \phi + asenm\theta \cdot sen\phi \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = \frac{{a \cdot \cos m\theta \cdot \cos \phi + a \cdot senm\theta \cdot sen\phi }}<br />{{\cos \phi }} \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = a\cos m\theta + a \cdot senm\theta \cdot tg\phi \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = a\cos m\theta + \frac{{\cos \phi \cdot b}}<br />{{sen\phi }} \cdot senm\theta \cdot \frac{{sen\phi }}<br />{{\cos \phi }} \hfill \\<br /> \frac{{a\cos (m\theta - \phi )}}<br />{{\cos \phi }} = a\cos m\theta + b \cdot senm\theta \hfill \\ <br />\end{gathered} <br />\]$ Con razón no me salía xd, saludos daniel ! Sorry sorry xD Y se vaa --------------------

« Posterior · Trigonometría · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 28th October 2025 - 10:00 PM