Control 2: Calculo Avanzado y aplicaciones 2009/2 - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Chile > Cálculo Avanzado

2 Páginas:

1 2 >

Reply to this topic

Start new topic

Control 2: Calculo Avanzado y aplicaciones 2009/2, Variable compleja

Rattlehead_ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 23 2009, 08:29 PM Publicado: #1
Dios Matemático Grupo: Usuario FMAT Mensajes: 428 Registrado: 19-July 07 Desde: Ñuñoa Miembro Nº: 7.679 Nacionalidad: Universidad: Sexo:	CONTROL 2: MA2002 Calculo Avanzado y Aplicaciones $TEX: <br />\noindent \fbox{Problema 1}\\<br /><br />\noindent a) Encuentre el radio de convergencia de la serie $\displaystyle \sum_{n=0}^{\infty} \dfrac{e^{in}}{2n+1} \left( \dfrac{2}{3i} \right) ^n(z+4i)^n$ \\<br /><br />\noindent b) Encuentre la serie de Taylor de $f(z)=z \log(z)$ en torno a $z_0=1+i$ y determine su radio de convergencia. \\ <br /><br />\noindent c) Sea $f: \mathbb{C} \rightarrow \mathbb{C}$ holomorfa tal que $f''(z)=2f(z)+1$ con $f(0)=1$, $f'(0)=0$. Encuentre la serie de potencias de $f$ en torno a $0$ y determine su radio de convergencia. \\<br /><br />\noindent d) Sea $f$ holomorfa en $\mathbb{C}$. Pruebe que si $Re(f), Im(f)$, o $\|f\|$ es constante, entonces $f$ es constante.$ $TEX: <br />\noindent \fbox{Problema 2} \\<br /><br />\noindent a) Calcule $\displaystyle \oint_{\|z\|=4} \dfrac{1}{z^2\sinh(z)}dz$, con la circunferenca $\|z\|=4$ recorrida en sentido antihorario. \\<br /><br />\noindent b) Pruebe que $\displaystyle \sum_{n=0}^{\infty} \left( \dfrac{\alpha^n}{n!} \right)^2=\dfrac{1}{2\pi} \int_0^{2\pi}\exp(2\alpha \cos(\theta)d\theta$\\<br />Indicacion: Comienze probando que $\displaystyle \left( \dfrac{\alpha^n}{n!} \right)^2= \dfrac{1}{2\pi i }\oint_{\|z\|=1} \dfrac{\alpha^n \exp(\alpha z)}{n! z^{n+1}}dz$$ $TEX: <br />\noindent \fbox{Problema 3}\\<br /><br />\noindent a) Calcule $\displaystyle \int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \dfrac{1}{a+\sin^2(\theta)} d\theta$ con $\alpha >0$\\<br /><br />\noindent b) Calcule $\displaystyle \int_0^{\infty} \exp(-x^2) \cos(2\beta x) dx$ donde $\beta>0$ \\<br />Indicacion: Integre $f(z)= \exp(-z^2)$ sobre el rectangulo de vertices $R, R+i\beta, -R, -R+i\beta$ y considere el limite $R\rightarrow \infty$ probando que las integrales sobre los lados verticales tienden a cero.$ -------------------- "Las Matematicas son el alfabeto con el cual Dios ha escrito el universo"

Jean Renard Gran... Jean Renard Granier Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 24 2009, 03:44 PM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad: Universidad: Sexo:	Buen control, parece que igual los rajaron un poco. El primer problema, destaco la parte c, un poco de paciencia. El segundo problema, parte a. Bonita integral, si bien no escapa mucho de técnicas estándar. El segundo problema, parte b. Creo que ese fue un problema cortacabezas por excelencia en el presente control El tercer problema, 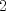$TEX: $$2$$$ integrales previamente preguntadas en el foro, no son difíciles, especialmente la segunda. Atentamente Jean Renard Granier Mensaje modificado por Jean Renard Granier el Oct 24 2009, 03:45 PM -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 26 2009, 06:22 PM Publicado: #3
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Jean Renard Granier @ Oct 24 2009, 04:44 PM) El primer problema, destaco la parte c, un poco de paciencia. El segundo problema, parte a. Bonita integral, si bien no escapa mucho de técnicas estándar. Cacho super poco más allá de cálculo 3, por eso te agradecería que postearas el desarrollo de los problemas que te cité, no es malo ver problemas resueltos -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

Findecano Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 26 2009, 06:32 PM Publicado: #4
Maestro Matemático Grupo: Usuario FMAT Mensajes: 89 Registrado: 28-January 08 Miembro Nº: 15.062 Nacionalidad: Universidad: Sexo:	Nos rajaron en el 1 y ahora los problemas eran larguisimos!!!!!!! con miles de polos! ¬¬ -------------------- Dondequiera que se ama el arte de la medicina se ama también a la humanidad (Platón)

PsicoStitch Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 27 2009, 09:27 PM Publicado: #5
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 699 Registrado: 6-May 07 Miembro Nº: 5.650 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Findecano @ Oct 26 2009, 07:32 PM) Nos rajaron en el 1 y ahora los problemas eran larguisimos!!!!!!! con miles de polos! ¬¬ el polo d orden 3 de la pregunta 2 estaba pajerisimo --------------------

Jean Renard Gran... Jean Renard Granier Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 27 2009, 09:44 PM Publicado: #6
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad: Universidad: Sexo:	CITA(PsicoStitch @ Oct 27 2009, 10:27 PM) el polo d orden 3 de la pregunta 2 estaba pajerisimo $TEX: <br />\[<br />\begin{gathered}<br /> {\text{Por calcular }}\mathop{\int\mkern-20.8mu \circlearrowleft}\nolimits_{\left\| z \right\| = 4} <br /> {f\left( z \right)dz,f\left( z \right) = \tfrac{1}<br />{{z^2 \sinh \left( z \right)}}} . \hfill \\<br /> {\text{La forma a integrar esta centrada en el origen y tiene radio }}4.{\text{ }} \hfill \\<br /> {\text{Dada esa informacion}}{\text{, }}f\left( z \right){\text{ admite como posibles polos }}z_1 = - \pi i,{\text{ }}z_2 = \pi i,z_3 = 0,{\text{con }} \hfill \\<br /> ord\left( {z_1 } \right) = ord\left( {z_2 } \right) = 1,ord\left( {z_3 } \right) = 3. \hfill \\<br /> {\text{Partiendo por lo mas rapido}}{\text{, se analizan en detalle }}z_1 {\text{ y }}z_2 . \hfill \\<br /> {\text{La formula a aplicar con Residuos es en cada caso}} \hfill \\<br /> 2\pi i\Re es\left( {f,z_i } \right),i = 1,2. \hfill \\<br /> {\text{Donde }}\Re es\left( {f,z_1 } \right) = \mathop {\lim }\limits_{z \to z_1 } \tfrac{{\left( {z - z_1 } \right)}}<br />{{z^2 \sinh \left( z \right)}},\Re es\left( {f,z_2 } \right) = \mathop {\lim }\limits_{z \to z_2 } \tfrac{{\left( {z - z_2 } \right)}}<br />{{z^2 \sinh \left( z \right)}}{\text{, se pueden resolver usando Hopital }}{\text{.}} \hfill \\<br /> {\text{Por ultimo}}{\text{, se analiza en detalle }}z_3 .{\text{ }} \hfill \\<br /> {\text{La formula a aplicar con Residuos en este caso es}} \hfill \\<br /> 2\pi i\Re es\left( {f,z_3 } \right). \hfill \\<br /> {\text{Ahora}}{\text{, observar que }}\mathop {\lim }\limits_{z \to z_3 } \tfrac{{\left( {z - z_3 } \right)^3 }}<br />{{z^2 \sinh \left( z \right)}} = \mathop {\lim }\limits_{z \to 0} \tfrac{{z^3 }}<br />{{z^2 \sinh \left( z \right)}} = \mathop {\lim }\limits_{z \to 0} \tfrac{z}<br />{{\sinh \left( z \right)}} = 1{\text{ existe}}{\text{.}} \hfill \\<br /> {\text{Con esto se puede decir que}} \hfill \\<br /> \Re es\left( {f,0} \right) = \mathop {\lim }\limits_{z \to 0} \tfrac{1}<br />{{2!}}\tfrac{{\partial ^2 }}<br />{{\partial z^2 }}\left( {z^3 f\left( z \right)} \right) = \mathop {\lim }\limits_{z \to 0} \tfrac{1}<br />{{2!}}\tfrac{{\partial ^2 }}<br />{{\partial z^2 }}\left( {\tfrac{z}<br />{{\sinh \left( z \right)}}} \right){\text{, se los dejo para calcularlo}}{\text{.}} \hfill \\<br /> {\text{Por lo tanto}}{\text{, }}\mathop{\int\mkern-20.8mu \circlearrowleft}\nolimits_{\left\| z \right\| = 4} <br /> {f\left( z \right)dz = } 2\pi i\Re es\left( {f, - \pi i} \right) + 2\pi i\Re es\left( {f,\pi i} \right) + 2\pi i\Re es\left( {f,0} \right). \hfill \\ <br />\end{gathered} <br />\]<br />$ Sobre la otra pregunta Nacho, la verdad es que con los conocimientos que tienes podrías hacerla sin mucho problemas, es recursiva man. Atentamente Jean Renard Granier -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.

Pedro² Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 27 2009, 10:23 PM Publicado: #7
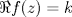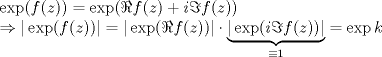Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	La 1.d) Si $TEX: $\Re{f(z)}=k$$ , constante, se tiene entonces que $TEX: \noindent $\exp(f(z))=\exp(\Re{f(z)}+i\Im{f(z)})\\<br />\Rightarrow \|\exp(f(z))\|=\|\exp(\Re{f(z)})\|\cdot\underbrace{\|\exp(i\Im{f(z)})\|}_{\equiv 1}=\exp k$$ Luego, $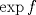TEX: $\exp f$$ es entera (por ser composición de funciones enteras) y acotada en $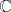TEX: $\mathbb{C}$$ (por ser constante), se concluye del teorema de Liouville que $TEX: $\exp f$$ es constante. 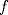Por lo anterior, $TEX: $f$$ es constante. 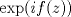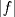Para la parte imaginaria la demostración es análoga considerando $TEX: $\exp (if(z))$$ , y para $TEX: $\|f\|$$ es directo por el mismo argumento xD. Saludos =) PD: La 3.b) está resuelta aquí usando variable compleja -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática* - Mechón 2009* "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy

DressedToKill Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 27 2009, 10:27 PM Publicado: #8
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.818 Registrado: 21-December 06 Miembro Nº: 3.434	La 1.d) en mi opinión es más fácil ocupando las ecuaciones de Cauchy-Riemann (alguien motivado que ponga la solución usándolas haha). -------------------- http://www.youtube.com/watch?v=sp_WV91jx8E...feature=related

Gazoo Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 4 2009, 10:38 PM Publicado: #9
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.112 Registrado: 21-December 05 Desde: El Bosque - Stgo Miembro Nº: 473 Colegio/Liceo: Universidad: Sexo:	La 2.b), la indicación no sale directo por F.I. de Cauchy?... y para probar lo que se pide, Laurent?... una manito porfa. -------------------- "El sentido común es el conjunto de todos los prejuicios adquiridos antes de los 18 años" A. Einstein. Estudiante Ingeniería Civil Eléctrica - DIE USACH

PsicoStitch Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 5 2009, 12:18 AM Publicado: #10
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 699 Registrado: 6-May 07 Miembro Nº: 5.650 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	La pauta esta en u cursos. La indicacion sale directo por cauchy. --------------------

« Posterior · Cálculo Avanzado · Anterior »

2 Páginas:

1 2 >

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 09:23 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.