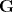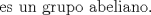|
|
|
|
|
|
|
  |
 Jul 22 2009, 07:22 PM Jul 22 2009, 07:22 PM
Publicado:
#1
|
|
|
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463 |
-------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau
|
|
|
|
 Jan 23 2015, 10:26 PM Jan 23 2015, 10:26 PM
Publicado:
#2
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 465 Registrado: 15-July 11 Miembro Nº: 91.905 Nacionalidad:  Universidad:  Sexo:  |
Sea 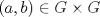 . La aplicación . La aplicación 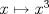 es un automorfismo, en particular es sobreyectiva. Esto significa que existe es un automorfismo, en particular es sobreyectiva. Esto significa que existe 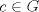 tal que tal que  . Como . Como 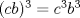 , entonces , entonces  . Luego . Luego 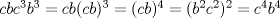 . Por ley de cancelación se tiene que . Por ley de cancelación se tiene que  y se concluye que y se concluye que 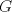 es abeliano. es abeliano. 
-------------------- He-llo? Could you say that again? More slowly? In a language I understand? Depending on what you said, I might kick your ass! |
|
|
|
 Apr 20 2016, 06:12 PM Apr 20 2016, 06:12 PM
Publicado:
#3
|
|
|
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463 |
Muy bien, Cenizas con Mostaza. Aunque, si no recuerdo mal, bastaba con demostrar que
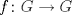 dada por dada por 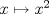 es un homomorfismo de es un homomorfismo de 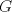 . .
-------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 12th July 2025 - 02:41 AM |






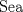
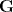
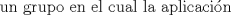
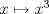
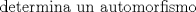 .
.