|
|
|
|
|
|
|
  |
 Jul 1 2009, 03:11 PM Jul 1 2009, 03:11 PM
Publicado:
#1
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 457 Registrado: 10-April 07 Miembro Nº: 5.093 Nacionalidad:  Sexo:  |
Hola necesito ayuda con este problema D:
saludos
Archivo(s) Adjunto(s)
|
|
|
|
 Jul 1 2009, 05:07 PM Jul 1 2009, 05:07 PM
Publicado:
#2
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad:  Sexo:  |
Como
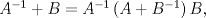 entonces entonces 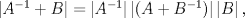 y y 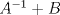 es invertible y su inversa es invertible y su inversa 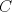 verifica verifica 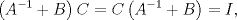 luego luego 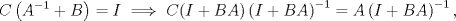 y así y así  y el otro es lado es un poco más truculento, tenemos: y el otro es lado es un poco más truculento, tenemos: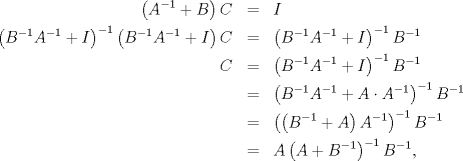 y estamos listos. |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 15th July 2025 - 03:54 PM |









