|
|
|
|
|
|
|
  |
 Sep 1 2009, 09:41 PM Sep 1 2009, 09:41 PM
Publicado:
#61
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
De acuerdo, Pasten. Mi propuesta es: Existen números perfectos pares en la sucesión de Fibonacci. ¿Cierto o falso? ![TEX: <br />\[<br />\begin{gathered}<br /> {\text{Han tocado uno de mis temas favoritos}}{\text{, posteo mi respuesta}}{\text{. }} \hfill \\<br /> {\text{No existen numeros perfectos pares en la Sucesion de Fibonacci}}{\text{. }} \hfill \\<br /> {\text{Como bonus}}{\text{, puedo asegurar que tampoco existen dichos numeros en la Sucesion de Lucas}}{\text{.}} \hfill \\<br /> {\text{En primer lugar}}{\text{, definimos }}P{\text{ como los Numeros Primos}}. \hfill \\<br /> {\text{Definimos un numero de la Sucesion de Fibonacci como }}F_{n \in \mathbb{N}} {\text{. }} \hfill \\<br /> {\text{De forma analoga}}{\text{, definimos un numero de la Sucesion de Lucas como }}L_{n \in \mathbb{N}} . \hfill \\<br /> {\text{Primero}}{\text{, se sabe que si 2}}^p - 1 \in P{\text{ tq }}p \in P,{\text{ entonces una condicion necesaria y suficiente }} \hfill \\<br /> {\text{para que un numero par }}n_{par} {\text{ sea perfecto es que necesariamente debe ser de la forma }} \hfill \\<br /> 2^{p - 1} \left( {{\text{2}}^p - 1} \right)\left( 1 \right). \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/40670f3a70c23ae0ecd73fa6edb9ab2b.png) ![TEX: <br />\[<br />\begin{gathered}<br /> {\text{Segundo}}{\text{, evaluamos por casos}}{\text{.}} \hfill \\<br /> {\text{Primer caso }} \hfill \\<br /> {\text{Sea }}n_s = 2^{p - 1} \left( {{\text{2}}^p - 1} \right){\text{ un numero segun }}\left( 1 \right). \hfill \\<br /> {\text{Suponga que }}\exists n_0 \in \mathbb{N}{\text{ tq }}F_{n_0 } = n_s . \hfill \\<br /> {\text{Dado que todo numero par perfecto es de la forma }}\left( 1 \right),{\text{ }}\left. {16} \right|n_s {\text{ tq }}n_s > 28. \hfill \\<br /> {\text{Ahora}}{\text{, se sabe que los unicos numeros de dicha sucesion divisibles por }}16{\text{ tambien lo}} \hfill \\<br /> {\text{son por }}9,{\text{ contradiccion}}. \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/c46b9a0844219fd73fb630e4a0544809.png) ![TEX: <br />\[<br />\begin{gathered}<br /> {\text{Segundo caso}}{\text{.}} \hfill \\<br /> {\text{Sea }}n_s = 2^{p - 1} \left( {{\text{2}}^p - 1} \right){\text{ un numero segun }}\left( 1 \right). \hfill \\<br /> {\text{Suponga que }}\exists n_0 \in \mathbb{N}{\text{ tq }}L_{n_0 } = n_s . \hfill \\<br /> {\text{En primer lugar}}{\text{, evaluando en }}\left( 1 \right),{\text{ obtenemos para }}p = 2{\text{ }}n_s = 6{\text{ y para }}p = 3 \hfill \\<br /> n_s = 28\left( 2 \right). \hfill \\<br /> {\text{Sabemos que los numeros obtenidos en }}\left( 2 \right){\text{ no existen en la Sucesion de Lucas}}{\text{. }} \hfill \\<br /> {\text{Entonces}}{\text{, sin perder generalidad}}{\text{, se puede suponer que }}p > 3\left( 3 \right).{\text{ }} \hfill \\<br /> {\text{Por }}\left( 3 \right),{\text{ }}\left. 8 \right|L_{r \in \mathbb{N}} {\text{, contradiccion}}{\text{.}} \hfill \\<br /> {\text{Esperando una respuesta}}{\text{, estimado Jose}} \hfill \\<br /> {\text{Atentamente}} \hfill \\<br /> {\text{Jean}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/b66a98d9b2954e88ad5b06312c540a43.png)
-------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 Sep 2 2009, 07:57 AM Sep 2 2009, 07:57 AM
Publicado:
#62
|
|
|
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463 |
Hay algunos detalles que sería bueno aclarar.
-------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau
|
|
|
|
 Sep 2 2009, 06:00 PM Sep 2 2009, 06:00 PM
Publicado:
#63
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
Partiendo por una facilita.
Toda métrica generalizada es una métrica. Demuestre o refute. -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 Sep 2 2009, 06:24 PM Sep 2 2009, 06:24 PM
Publicado:
#64
|
|
|
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463 |
Falso. La función
 es una métrica generalizada sobre los números reales que no es métrica (pues no cumple con la desigualdad triangular). es una métrica generalizada sobre los números reales que no es métrica (pues no cumple con la desigualdad triangular).
-------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau
|
|
|
|
 Sep 2 2009, 06:26 PM Sep 2 2009, 06:26 PM
Publicado:
#65
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
Falso. La función  es una métrica generalizada sobre los números reales, pero no determina una métrica sobre dicho conjunto. es una métrica generalizada sobre los números reales, pero no determina una métrica sobre dicho conjunto.Bien José, ahora proponga. -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 Sep 2 2009, 06:33 PM Sep 2 2009, 06:33 PM
Publicado:
#66
|
|
|
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463 |
Sea R un anillo cuyo único elemento nilpotente es el 0. Se afirma entonces que todo elemento idempotente de R pertenece al centro de R. ¿Cierto o falso?
-------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau
|
|
|
|
 Sep 6 2009, 03:41 PM Sep 6 2009, 03:41 PM
Publicado:
#67
|
|
|
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463 |
Vamos, Pasten... ¡Tú puedes, bacán!
-------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau
|
|
|
|
 Sep 6 2009, 04:58 PM Sep 6 2009, 04:58 PM
Publicado:
#68
|
|
|
Dios Matemático Supremo Grupo: Baneado Mensajes: 2.588 Registrado: 7-November 06 Miembro Nº: 2.747 |
MP mejor
Mensaje modificado por Jorgeston el Sep 6 2009, 04:58 PM |
|
|
|
 Sep 6 2009, 06:50 PM Sep 6 2009, 06:50 PM
Publicado:
#69
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
Sea R un anillo cuyo único elemento nilpotente es el 0. Se afirma entonces que todo elemento idempotente de R pertenece al centro de R. ¿Cierto o falso? Vamos, Pasten... ¡Tú puedes, bacán! Calma José, cuando él tenga tiempo de responder lo va a hacer. Let it be man, no te hagas mala sangre. -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 Sep 7 2009, 12:02 PM Sep 7 2009, 12:02 PM
Publicado:
#70
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 765 Registrado: 6-December 05 Miembro Nº: 458 Nacionalidad:  Sexo:  |
Sea R un anillo cuyo único elemento nilpotente es el 0. Se afirma entonces que todo elemento idempotente de R pertenece al centro de R. ¿Cierto o falso? Sobre esa pregunta, pense que no era adecuado que conteste ese tipo de trivialidades. Crei mejor dejarlo a alguien mas, porque ademas de ser facil no era una pregunta con mucha gracia. Pero dado que estamos en estas: Vamos, Pasten... ¡Tú puedes, bacán! tendre que contestar: Cierto. Sea  idempotente, como idempotente, como 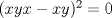 entonces entonces 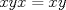 . Similarmente . Similarmente 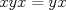 por lo que por lo que 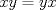 . .Ahora, como la respuesta esta correcta propongo el siguiente: Definicion previa para que todos entiendan: Si K es un campo K[x] son los polinomios de indeterminada x y coeficientes en K, y K(x) son las fracciones de polinomios de K[x] (el campo de las funciones racionales sobre K). V o F?: Sea K campo de caracteristica cero. Suponga que F es una funcion racional sobre K tal que F, F+1, F+2 son cuadrados de K(x). Entonces F es constante. Saludos -------------------- Pasten, un buen muchacho en quien confiar.
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 13th July 2025 - 03:37 AM |










