Propuesto V: Desigualdad

Foro fmat.cl > Sector Preparación Olimpiadas > Sector PreOlímpico > Desigualdades > Problemas Resueltos

Propuesto V: Desigualdad, no requiere nada avanzado

Opciones

~Fatal_Collapse~ Ver Perfil del Miembro Ver los mensajes de este miembro	May 23 2009, 07:24 PM Publicado: #1
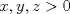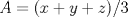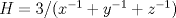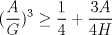Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.564 Registrado: 12-November 07 Desde: La Union, XIV Region de los Rios Miembro Nº: 12.607 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Sean $TEX: $x,y,z>0$$ . Sean $TEX: $A=(x+y+z)/3$$ , $TEX: $G=\sqrt[3]{xyz}$$ y $TEX: $H=3/(x^{-1}+y^{-1}+z^{-1})$$ . Demuestre que: $TEX: $(\displaystyle \frac{A}{G})^3\ge \displaystyle \frac{1}{4}+\displaystyle \frac{3A}{4H}$$ Fuente: IMO longlist 1992, propuesto por Polonia -------------------- Ricardo Vargas Obando Ex-alumno Deutsche Schule La Unión (Generación 2010, de los 150 años). Novato de Licenciatura en Matemática/Estadística, en la Pontificia Universidad Católica de Chile. Grupo de facebook de Novatos Matemática y Estadística PUC 2011 Currículum Olímpico: Medalla de Plata, XIX Olimpiada Nacional de Matemática Nivel Menor (2007) Medalla de Oro, XXI Olimpiada Nacional de Matemática Nivel Mayor (2009) Medalla de Bronce, XXV Olimpiada Iberoamericana de Matemática (2010) Medalla de Oro, XXII Olimpiada Nacional de Matemática Nivel Mayor (2010) "What we learned as children, that one plus one equals two, we know to be false. One plus one equals one. We even have a word when you plus another, equals one. That word is love." "Todos piensan en cambiar el mundo, pero nadie piensa en cambiarse a sí mismo."

Killua Ver Perfil del Miembro Ver los mensajes de este miembro	May 23 2009, 08:22 PM Publicado: #2
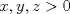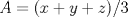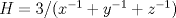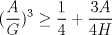Staff Fmat Grupo: Moderador Mensajes: 1.185 Registrado: 29-October 05 Desde: Santiago, Chile Miembro Nº: 352 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Kain #13 @ May 23 2009, 08:24 PM) Sean $TEX: $x,y,z>0$$ . Sean $TEX: $A=(x+y+z)/3$$ , $TEX: $G=\sqrt[3]{xyz}$$ y $TEX: $H=3/(x^{-1}+y^{-1}+z^{-1})$$ . Demuestre que: $TEX: $(\displaystyle \frac{A}{G})^3\ge \displaystyle \frac{1}{4}+\displaystyle \frac{3A}{4H}$$ Fuente: IMO longlist 1992, propuesto por Polonia $TEX: \noindent Por la desigualdad $A\ge{H}$, se tiene que $\dfrac{x+y+z}{3}\ge\dfrac{3xyz}{xy+yz+zx}\Rightarrow{xy+yz+zx}\ge\dfrac{9xyz}{x+y+z}$, y de la desigualdad $x^2+y^2+z^2\ge{xy+yz+zx}$ $(i)$, se sigue que $x^2+y^2+z^2\ge\dfrac{9xyz}{x+y+z}$.\\<br /><br />\noindent Multiplicando por $3$, y sumando $(i)$:<br /><br />$$3(x^2+y^2+z^2)\ge\dfrac{27xyz}{x+y+z}$$<br />$$4(x^2+y^2+z^2)\ge\dfrac{27xyz}{x+y+z}+xy+yz+zx$$<br />$$4(x^2+y^2+z^2)+8(xy+yz+zx)\ge\dfrac{27xyz}{x+y+z}+9(xy+yz+zx)$$<br />$$4(x+y+z)^2\ge{9\left(\dfrac{3xyz}{x+y+z}+xy+yz+zx\right)}$$<br />$$4(x+y+z)^3\ge{9(3xyz+(x+y+z)(xy+yz+zx))}$$<br />$$12(x+y+z)^3\ge{27(3xyz+(x+y+z)(xy+yz+zx)}$$<br />$$\boxed{\dfrac{(x+y+z)^3}{27xyz}\ge{\dfrac{3xyz+(x+y+z)(xy+yz+zx)}{12xyz}}}$$<br /><br />\noindent Esta \'ultima desigualdad es equivalente a $\left(\dfrac{A}{G}\right)^3\ge\dfrac{H+3A}{4H}$, probando as\'i lo pedido $\blacksquare$<br />$ Saludos. -------------------- "He looks rather ill, but he looks all over the genius he was" (G. H. Hardy) "A mathematician is a device for turning coffee into theorems" (Paul Erdös)

~Fatal_Collapse~ Ver Perfil del Miembro Ver los mensajes de este miembro	May 23 2009, 08:27 PM Publicado: #3
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.564 Registrado: 12-November 07 Desde: La Union, XIV Region de los Rios Miembro Nº: 12.607 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Wow killua, que rapidez!!!! Y la solucion es igual a la mia =) SAludos y que bueno que estes activo =) -------------------- Ricardo Vargas Obando Ex-alumno Deutsche Schule La Unión (Generación 2010, de los 150 años). Novato de Licenciatura en Matemática/Estadística, en la Pontificia Universidad Católica de Chile. Grupo de facebook de Novatos Matemática y Estadística PUC 2011 Currículum Olímpico: Medalla de Plata, XIX Olimpiada Nacional de Matemática Nivel Menor (2007) Medalla de Oro, XXI Olimpiada Nacional de Matemática Nivel Mayor (2009) Medalla de Bronce, XXV Olimpiada Iberoamericana de Matemática (2010) Medalla de Oro, XXII Olimpiada Nacional de Matemática Nivel Mayor (2010) "What we learned as children, that one plus one equals two, we know to be false. One plus one equals one. We even have a word when you plus another, equals one. That word is love." "Todos piensan en cambiar el mundo, pero nadie piensa en cambiarse a sí mismo."

Killua Ver Perfil del Miembro Ver los mensajes de este miembro	May 24 2009, 07:31 PM Publicado: #4
Staff Fmat Grupo: Moderador Mensajes: 1.185 Registrado: 29-October 05 Desde: Santiago, Chile Miembro Nº: 352 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Kain #13 @ May 23 2009, 09:27 PM) Wow killua, que rapidez!!!! Y la solucion es igual a la mia =) SAludos y que bueno que estes activo =) Jeje, se hace lo que se puede, cuando alcanza el tiempo. Saludos -------------------- "He looks rather ill, but he looks all over the genius he was" (G. H. Hardy) "A mathematician is a device for turning coffee into theorems" (Paul Erdös)

« Posterior · Problemas Resueltos · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 09:56 PM