guia de limites - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Federico Santa María > Mat 021

11 Páginas:

« < 6 7 8 9 10 > »

Reply to this topic

Start new topic

guia de limites

Angedm Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:15 PM Publicado: #71
Principiante Matemático Grupo: Usuario FMAT Mensajes: 6 Registrado: 11-April 09 Miembro Nº: 47.821 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	holaaa, por casualidad alguien sabe como hace la i) del item 13????

Pedro² Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:15 PM Publicado: #72
Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Ussop @ May 3 2009, 09:40 PM) $TEX: \[<br />\begin{gathered}<br /> \left. {17} \right)\mathop {\lim }\limits_{x \to 1} \frac{{x^n - 1}}<br />{{x^m - 1}} = \frac{{\left( {x - 1} \right)\left( {x^{n - 1} + x^{n - 2} + x^{n - 3} + .... + x + 1} \right)}}<br />{{\left( {x - 1} \right)\left( {x^{m - 1} + x^{m - 2} + x^{m - 3} + .... + x + 1} \right)}} = \hfill \\<br /> \frac{{\left( {x^{n - 1} + x^{n - 2} + x^{n - 3} + .... + x + 1} \right)}}<br />{{\left( {x^{m - 1} + x^{m - 2} + x^{m - 3} + .... + x + 1} \right)}} = \frac{n}<br />{m} \hfill \\<br /> \left. {19} \right)\mathop {\lim }\limits_{x \to 1} \frac{{\sqrt[n]{x} - 1}}<br />{{\sqrt[m]{x} - 1}} \hfill \\<br /> u = \sqrt[{mn}]{x} \hfill \\<br /> \sqrt[n]{x} = x^{\frac{1}<br />{n}} = \sqrt[{mn}]{{\left( {x^{\frac{1}<br />{n}} } \right)^{mn} }} = \sqrt[{mn}]{{x^m }} = u^m \hfill \\<br /> \sqrt[m]{x} = x^{\frac{1}<br />{m}} = \sqrt[{mn}]{{\left( {x^{\frac{1}<br />{m}} } \right)^{mn} }} = \sqrt[{mn}]{{x^n }} = u^n \hfill \\<br /> \mathop {\lim }\limits_{u \to 1} \frac{{u^m - 1}}<br />{{u^n - 1}} = = \frac{{\left( {u - 1} \right)\left( {u^{m - 1} + u^{m - 2} + u^{m - 3} + .... + u + 1} \right)}}<br />{{\left( {u - 1} \right)\left( {u^{n - 1} + u^{n - 2} + u^{n - 3} + .... + u + 1} \right)}} = \hfill \\<br /> \frac{{\left( {u^{m - 1} + u^{m - 2} + u^{m - 3} + .... + u + 1} \right)}}<br />{{\left( {u^{n - 1} + u^{n - 2} + u^{n - 3} + .... 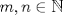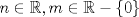+ u + 1} \right)}} = \frac{m}<br />{n} \hfill \\ <br />\end{gathered} <br />\]$ Ojo en el 17, si bien ese desarrollo es válido para $TEX: $m,n\in\mathbb{N}$$ , el valor del límite sigue siendo el mismo para $TEX: $n\in\mathbb{R},m\in\mathbb{R}-\{0\}$$ . 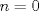Excluiremos el caso de $TEX: $n=0$$ en el desarrollo, pues es directo. $TEX: \[<br />\begin{gathered}<br /> \mathop {\lim }\limits_{x \to 1} \frac{{x^n - 1}}<br />{{x^m - 1}}\;\;n,m \ne 0 \hfill \\<br /> {\textsf{Haciendo }}z = x - 1,{\textsf{ el limite equivale a:}} \hfill \\<br /> \mathop {\lim }\limits_{z \to 0} \frac{{\left( {z + 1} \right)^n - 1}}<br />{{(z + 1)^m - 1}} = \mathop {\lim }\limits_{z \to 0} \frac{{\exp \left\{ {n\ln (z + 1)} \right\} - 1}}<br />{{\exp \left\{ {m\ln (z + 1)} \right\} - 1}} \cdot \frac{{n\ln (z + 1)}}<br />{{n\ln (z + 1)}} \cdot \frac{{m\ln (z + 1)}}<br />{{m\ln (z + 1)}} \hfill \\<br /> = \mathop {\lim }\limits_{z \to 0} \frac{{\exp \left\{ {n\ln (z + 1)} \right\} - 1}}<br />{{n\ln (z + 1)}} \cdot \frac{{m\ln (z + 1)}}<br />{{\exp \left\{ {m\ln (z + 1)} \right\} - 1}} \cdot \frac{n}<br />{m} \hfill \\<br /> {\textsf{Usando el hecho de que }}\mathop {\lim }\limits_{x \to 0} \frac{{\exp x - 1}}<br />{x} = 1,{\textsf{ se sigue que:}} \hfill \\<br /> \mathop {\lim }\limits_{z \to 0} \frac{{\exp \left\{ {n\ln (z + 1)} \right\} - 1}}<br />{{n\ln (z + 1)}} \cdot \frac{{m\ln (z + 1)}}<br />{{\exp \left\{ {m\ln (z + 1)} \right\} - 1}} \cdot \frac{n}<br />{m} = 1 \cdot 1 \cdot \frac{n}<br />{m} \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to 1} \frac{{x^n - 1}}<br />{{x^m - 1}} = \frac{n}<br />{m} \hfill \\ <br />\end{gathered} <br />\]<br />$ También cuidado en el 21, deben considerar los diferentes casos ( 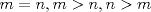$TEX: $m=n,m>n,n>m$$ ), pues entregan distintos resultados. Salu Mensaje modificado por Pedro² el May 3 2009, 09:17 PM -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática* - Mechón 2009* "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy

Ussop Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:16 PM Publicado: #73
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.349 Registrado: 8-October 07 Desde: Quilicura Miembro Nº: 11.057 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Ussop @ May 3 2009, 02:05 PM) $TEX: \[<br />\begin{gathered}<br /> \mathop {\lim }\limits_{x \to \infty } \left( {\sqrt {x + a} - \sqrt x } \right) \hfill \\<br /> \left( {\sqrt {x + a} - \sqrt x } \right) \cdot \frac{{\left( {\sqrt {x + a} + \sqrt x } \right)}}<br />{{\left( {\sqrt {x + a} + \sqrt x } \right)}} = \frac{{x + a - x}}<br />{{\sqrt {x + a} + \sqrt x }} = \frac{a}<br />{{\sqrt x \left( {\sqrt {1 + \frac{a}<br />{x}} + 1} \right)}} \hfill \\<br /> \mathop {\lim }\limits_{x \to \infty } \frac{a}<br />{{\sqrt x \left( {\sqrt {1 + \frac{a}<br />{x}} + 1} \right)}} = \frac{a}<br />{{\sqrt \infty \left( {\sqrt {1 + \frac{a}<br />{\infty }} + 1} \right)}} = \frac{a}<br />{{\sqrt \infty \left( {\sqrt {1 + 0} + 1} \right)}} = \frac{a}<br />{\infty } = 0 \hfill \\ <br />\end{gathered} <br />\]$ -------------------- Plan Comun Ingenieria USM 2009 Santiago Ingenieria Civil Electrica Usm 2010 Santiago

Angedm Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:19 PM Publicado: #74
Principiante Matemático Grupo: Usuario FMAT Mensajes: 6 Registrado: 11-April 09 Miembro Nº: 47.821 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	ahaha..gracias:D

Juzerman Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:24 PM Publicado: #75
Matemático Grupo: Usuario FMAT Mensajes: 56 Registrado: 1-May 09 Miembro Nº: 49.967 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Ussop @ May 3 2009, 10:12 PM) igual no entendi bn xD ahora cache como hacerlo, aca esta el resultado ojala te quede mas claro con este desarrollo $TEX: <br /><math>\begin{align}<br /> & \underset{x\to 0}{\mathop{\lim }}\,\frac{\ln \left( 1+kx \right)}{x} \\ <br /> & u=\ln (1+kx)\to e^{u}=1+kx\to x=\frac{e^{u}-1}{k} \\ <br /> & \underset{u\to 0}{\mathop{\lim }}\,\frac{u}{{}^{e^{u}-1}\!\!\diagup\!\!{}_{k}\;}\to \frac{uk}{e^{u}-1}\to \left( \frac{e^{u}-1}{u} \right)^{-1}\bullet k\to \left( \ln e \right)^{-1}\bullet k=k \\ <br />\end{align}</math><br />$

felipetrup Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:27 PM Publicado: #76
Maestro Matemático Grupo: Usuario FMAT Mensajes: 96 Registrado: 27-March 09 Desde: Valparaiso Miembro Nº: 46.206 Nacionalidad: Universidad: Sexo:	me pueden ayudar con los ejercicios de la seccion 14 pero los que vienen del ejercicio j, mi profe no nos mostro algun metodo de resolver esos ejercicos, talvaez mañana en mate nos enseñen como... por favor -------------------- ------------------------------------------------------------------------------------- - Ingenieria Civil - Universidad Técnica Federico Santa Maria Casa Central - Valparaiso - Chile El 98% de los adolescentes han fumado, si eres del dichoso 2% que no lo ha hecho, copia y pega esto en tu firma

Ussop Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:28 PM Publicado: #77
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.349 Registrado: 8-October 07 Desde: Quilicura Miembro Nº: 11.057 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Juzerman @ May 3 2009, 10:24 PM) ahora cache como hacerlo, aca esta el resultado ojala te quede mas claro con este desarrollo 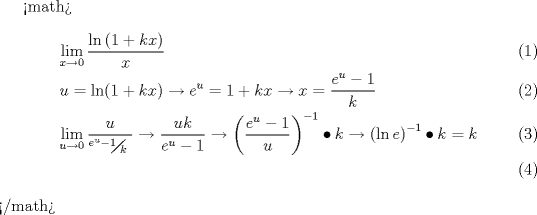$TEX: <br /><math>\begin{align}<br /> & \underset{x\to 0}{\mathop{\lim }}\,\frac{\ln \left( 1+kx \right)}{x} \\ <br /> & u=\ln (1+kx)\to e^{u}=1+kx\to x=\frac{e^{u}-1}{k} \\ <br /> & \underset{u\to 0}{\mathop{\lim }}\,\frac{u}{{}^{e^{u}-1}\!\!\diagup\!\!{}_{k}\;}\to \frac{uk}{e^{u}-1}\to \left( \frac{e^{u}-1}{u} \right)^{-1}\bullet k\to \left( \ln e \right)^{-1}\bullet k=k \\ <br />\end{align}</math><br />$ me quedo clarisimo -------------------- Plan Comun Ingenieria USM 2009 Santiago Ingenieria Civil Electrica Usm 2010 Santiago

mat!as Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:32 PM Publicado: #78
Dios Matemático Grupo: Usuario FMAT Mensajes: 464 Registrado: 23-February 08 Miembro Nº: 15.787 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	cabros, estoy algo urgido, todavia nisiquiera empiezo a hacer la guia de continuidad XDD.. alguien mas esta en las mismas que yo para no sentirme tan miserable??

Vileichon Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:32 PM Publicado: #79
Principiante Matemático Grupo: Usuario FMAT Mensajes: 7 Registrado: 26-October 08 Miembro Nº: 37.071	vale gracias

Ussop Ver Perfil del Miembro Ver los mensajes de este miembro	May 3 2009, 09:33 PM Publicado: #80
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.349 Registrado: 8-October 07 Desde: Quilicura Miembro Nº: 11.057 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(mat!as @ May 3 2009, 10:32 PM) cabros, estoy algo urgido, todavia nisiquiera empiezo a hacer la guia de continuidad XDD.. alguien mas esta en las mismas que yo para no sentirme tan miserable?? a mi me falta la de geo analitica y la de continuidad -------------------- Plan Comun Ingenieria USM 2009 Santiago Ingenieria Civil Electrica Usm 2010 Santiago

« Posterior · Mat 021 · Anterior »

11 Páginas:

« < 6 7 8 9 10 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 4th April 2025 - 02:57 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.