 Producto escalar |
|
|
|
|
|
|
Foro fmat.cl > Programa Enseñanza Universitaria > Consultas > Banco de Problemas Resueltos > Álgebra > Geometría Analitica  |
  May 1 2009, 08:22 PM
Publicado:
#1
|
|
 Dios Matemático Supremo  Grupo: Usuario FMAT Mensajes: 1.767 Registrado: 21-January 08 Desde: Santiago - Ancud Miembro Nº: 14.865 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:   |
Demuestre que para cualquier par de vectores, ambas definiciones del producto escalar son iguales:
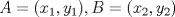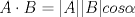Sean dos vectores,  , separados por un ángulo   y  Espero no esté 
-------------------- Estudia para superarte a ti mismo, no al resto. |
 |
 
|
|
May 2 2009, 11:52 PM
Publicado:
#2
|
|
 Maestro Matemático Grupo: Usuario FMAT Mensajes: 74 Registrado: 4-May 08 Desde: Ancud Miembro Nº: 22.124 Nacionalidad: Colegio/Liceo: Universidad:  Sexo: |
A base de Trigonometria, vectores unitarios y normas ^^!
![TEX: \[<br />\begin{gathered}<br /> A = (x_1 ,y_1 ) = \left( {\left[ {\left\| A \right\| \cdot \cos (\theta )} \right],\left[ {\left\| A \right\| \cdot sen(\theta )} \right]} \right) \hfill \\<br /> A = \left\| A \right\| \cdot \cos (\theta ) \cdot i + \left\| A \right\| \cdot sen(\theta ) \cdot j \hfill \\<br /> \hfill \\<br /> B = (x_2 ,y_2 ) = \left( {\left[ {\left\| B \right\| \cdot \cos (\theta + a)} \right],\left[ {\left\| B \right\| \cdot sen(\theta + a)} \right]} \right) \hfill \\<br /> B = \left\| B \right\| \cdot \cos (\theta + a) \cdot i + \left\| B \right\| \cdot sen(\theta + a) \cdot j \hfill \\<br /> \hfill \\<br /> {\text{Si rotamos ambos vectores }} - \theta {\text{ grados, haciendo que }}A{\text{ coincida con el eje }}x{\text{ nos queda:}} \hfill \\<br /> A = \left\| A \right\| \cdot \cos (0) \cdot i + \left\| A \right\| \cdot sen(0) \cdot j \hfill \\<br /> A = \left\| A \right\| \cdot i + 0 \hfill \\<br /> A = \left( {\left\| A \right\|,0} \right) \hfill \\<br /> \hfill \\<br /> B = \left\| B \right\| \cdot \cos (\theta + a - \theta ) \cdot i + \left\| B \right\| \cdot sen(\theta + a - \theta ) \cdot j \hfill \\<br /> B = \left\| B \right\| \cdot \cos (a) \cdot i + \left\| B \right\| \cdot sen(a) \cdot j \hfill \\<br /> B = \left( {\left\| B \right\| \cdot \cos (a),\left\| B \right\| \cdot sen(a)} \right) \hfill \\<br /> \hfill \\<br /> {\text{Tomando la definicion: }}\boxed{A \cdot B = x_1 x_2 + y_1 x_2 } \hfill \\<br /> A \cdot B = \left\| A \right\| \times \left\| B \right\| \cdot \cos (a) + 0 \times \left\| B \right\| \cdot sen(a) \hfill \\<br /> \boxed{A \cdot B = \left\| A \right\| \times \left\| B \right\| \cdot \cos (a)} \hfill \\ <br />\end{gathered} <br />\]](./tex/752d9425ac46c374c96a96f2ea2836fb.png) 
--------------------    |
|
|
|
|
Jul 31 2009, 01:18 AM
Publicado:
#3
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.767 Registrado: 21-January 08 Desde: Santiago - Ancud Miembro Nº: 14.865 Nacionalidad: Colegio/Liceo: Universidad: Sexo: |
-------------------- Estudia para superarte a ti mismo, no al resto. |
|
|
|
|
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 23rd November 2024 - 07:54 PM |
