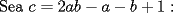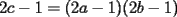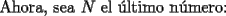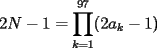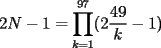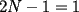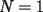|
|
|
|
|
|
|
  |
 Sep 22 2006, 06:00 PM Sep 22 2006, 06:00 PM
Publicado:
#1
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 556 Registrado: 16-August 06 Desde: Rio de Janeiro Miembro Nº: 1.950 Nacionalidad:  Colegio/Liceo:  Universidad: .png) Sexo:  |
En un pizarrón estan escritos los números
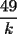 para para 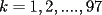 . En cada paso, dos números . En cada paso, dos números  y y 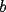 son borrados y el número son borrados y el número 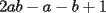 es agregado en su lugar. Luego de 96 pasos, solo queda un número en el pizarrón. Determinar todos los posibles valores de dicho número. es agregado en su lugar. Luego de 96 pasos, solo queda un número en el pizarrón. Determinar todos los posibles valores de dicho número.Suerte Mensaje modificado por Francisco Muñoz el Feb 23 2007, 12:28 AM |
|
|
|
 Jan 4 2007, 04:06 PM Jan 4 2007, 04:06 PM
Publicado:
#2
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 374 Registrado: 16-September 06 Desde: New Haven, CT, USA. Miembro Nº: 2.275 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Solución:
![TEX: \[<br />\begin{gathered}<br /> {\text{Tomemos la pareja (}}a_1 ,a_2 ).{\text{ Obteniendo el numero 2}}a_1 a_2 - a_1 - a_2 + 1 = \frac{{(2a_1 - 1)(2a_2 - 1)}}<br />{2} + \frac{1}<br />{2} \hfill \\<br /> {\text{Veamos lo que ocurre en el caso de tomar 3 numeros}}{\text{, obtenemos la pareja }} \hfill \\<br /> \left( {a_3 ,2a_1 a_2 - a_1 - a_2 + 1} \right).{\text{ Luego:}} \hfill \\<br /> 2a_3 \left( {2a_1 a_2 - a_1 - a_2 + 1} \right) - a_3 - \left( {2a_1 a_2 - a_1 - a_2 + 1} \right) + 1 \hfill \\<br /> = 4a_1 a_2 a_3 - 2a_1 a_3 - 2a_2 a_3 + 2a_3 - a_3 - 2a_1 a_2 + a_1 + a_2 - 1 + 1 \hfill \\<br /> = 4a_1 a_2 a_3 - 2a_1 a_3 - 2a_2 a_3 - 2a_1 a_2 + a_3 + a_1 + a_2 \hfill \\<br /> = \frac{{(2a_1 - 1)(2a_2 - 1)\left( {2a_3 - 1} \right)}}<br />{2} + \frac{1}<br />{2} \hfill \\<br /> {\text{Cuando tomamos 4 variables obtenemos:}} \hfill \\<br /> \frac{{(2a_1 - 1)(2a_2 - 1)\left( {2a_3 - 1} \right)\left( {2a_4 - 1} \right)}}<br />{2} + \frac{1}<br />{2} \hfill \\<br /> \hfill \\<br /> {\text{Ahora podemos apreciar que hay una recurrencia que se ve representada por esta expresion:}} \hfill \\<br /> \boxed{\left( {\frac{1}<br />{2}\prod\limits_{i = 1}^n {2a_i - 1} } \right) + \frac{1}<br />{2}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/1167944799.gif) ![TEX: \[<br />\begin{gathered}<br /> {\text{Probaremos por induccion:}} \hfill \\<br /> {\text{Cuando }}n = 1: \hfill \\<br /> \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^1 {2a_i - 1} } \right) + \frac{1}<br />{2} = \frac{1}<br />{2}\left( {2a_1 - 1} \right) + \frac{1}<br />{2} = a_1 \hfill \\<br /> {\text{Asumiendo que se cumple para }}n = k{\text{ probaremos para }}k + 1. \hfill \\<br /> {\text{Tenemos la pareja:}} \hfill \\<br /> \left[ {\left( {\frac{1}<br />{2}\prod\limits_{i = 1}^k {2a_i - 1} } \right) + \frac{1}<br />{2},a_{k + 1} } \right] \hfill \\<br /> {\text{Obteniendo el numero:}} \hfill \\<br /> = 2a_{k + 1} \left[ {\left( {\frac{1}<br />{2}\prod\limits_{i = 1}^k {2a_i - 1} } \right) + \frac{1}<br />{2}} \right] - a_{k + 1} - \left[ {\left( {\frac{1}<br />{2}\prod\limits_{i = 1}^k {2a_i - 1} } \right) + \frac{1}<br />{2}} \right] + 1 \hfill \\<br /> = 2a_{k + 1} \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^k {2a_i - 1} } \right) + \frac{1}<br />{2}\left( {2a_{k + 1} } \right) - a_{k + 1} - \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^k {2a_i - 1} } \right) - \frac{1}<br />{2} + 1 \hfill \\<br /> = \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^k {2a_i - 1} } \right)\left( {2a_{k + 1} - 1} \right) + \left( {a_{k + 1} - a_{k + 1} } \right) + 1 - \frac{1}<br />{2} \hfill \\<br /> = \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^{k + 1} {2a_i - 1} } \right) + \frac{1}<br />{2} \hfill \\<br /> {\text{Demostrando lo pedido}}{\text{.}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/1167944802.gif) ![TEX: <br />\[<br />\begin{gathered}<br /> {\text{Ahora podemos aplicar esta formula general al problema:}} \hfill \\<br /> {\text{Notemos que }}a_i = \frac{{49}}<br />{i} \hfill \\<br /> = \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^{97} {2a_i - 1} } \right) + \frac{1}<br />{2} = \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^{97} {2\left( {\frac{{49}}<br />{i}} \right) - 1} } \right) + \frac{1}<br />{2} = \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^{97} {\frac{{98}}<br />{i} - 1} } \right) + \frac{1}<br />{2} = \left( {\frac{1}<br />{2}\prod\limits_{i = 1}^{97} {\frac{{98 - i}}<br />{i}} } \right) + \frac{1}<br />{2} \hfill \\<br /> = \frac{1}<br />{2}\left( {\frac{{97}}<br />{1}\cdot\frac{{96}}<br />{2}\cdot\frac{{95}}<br />{3}\cdot\frac{{94}}<br />{4}\cdot...\cdot\frac{4}<br />{{94}}\cdot\frac{3}<br />{{95}}\cdot\frac{2}<br />{{96}}\cdot\frac{1}<br />{{97}}} \right) + \frac{1}<br />{2} = \frac{1}<br />{2}\left( {\frac{{97!}}<br />{{97!}}} \right) + \frac{1}<br />{2} = \frac{1}<br />{2} + \frac{1}<br />{2} = 1 \hfill \\<br /> {\text{Finalmente el numero que se obtiene es 1}}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{Salud}}\varphi {\text{os}} \hfill \\ <br />\end{gathered} <br />\]<br /><br />](./tex/1167944805.gif)
|
|
|
|
 Jan 4 2007, 04:27 PM Jan 4 2007, 04:27 PM
Publicado:
#3
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 556 Registrado: 16-August 06 Desde: Rio de Janeiro Miembro Nº: 1.950 Nacionalidad:  Colegio/Liceo:  Universidad: .png) Sexo:  |
Jejeje veo que has resuleto mis problemas
Solución alternativa: Saludos |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 14th July 2025 - 03:12 PM |