Dimensión - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Consultas > Banco de Problemas Resueltos > Álgebra > Álgebra Lineal

Reply to this topic

Start new topic

Dimensión, Uno a bote pronto

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	Apr 2 2009, 01:13 PM Publicado: #1
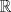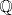Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	¿Cuál es la dimensión de $TEX: $\mathbb{R}$$ como espacio vectorial sobre $TEX: $\mathbb{Q}$$ ? Saludos. -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

DressedToKill Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 7 2009, 05:53 PM Publicado: #2
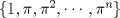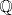Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.818 Registrado: 21-December 06 Miembro Nº: 3.434	Es infinito. La forma usual de demostrarlo (supongo) es considerar el conjunto $TEX: $\{1, \pi, \pi^2, \cdots, \pi^n\}$$ . El conjunto anterior es LI para cualquier n, pues en caso contrario tendríamos que $TEX: $\pi$$ es solución de algún polinomio en $TEX: $\mathbb{Q}$$ , pero muchos libros nos dicen que $TEX: $\pi$$ es trascendente, lo que sería una contradicción. Por lo anterior, no puede haber una base finita y de ahí que la dimensión es infinito. -------------------- http://www.youtube.com/watch?v=sp_WV91jx8E...feature=related

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 7 2009, 06:07 PM Publicado: #3
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	Buena, Dressed. Igual se puede mejorar un tanto, ¿no? Podríamos cambiar $TEX: $\pi$$ por otro número cuya trascendencia sea más fácil de obtener, ¿no? e viene a la mente de inmediato, pero su trascendencia tampoco queda al tiro. ¿Quién se anima a subir una prueba totalmente autocontenida de este desafío? Otro punto a considerar, ¿podemos hacer la demostración independiente del conocimiento de que el número fulano es trascendente? -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

Kaissa Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 7 2009, 08:09 PM Publicado: #4
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 9.897 Registrado: 6-April 08 Miembro Nº: 19.238 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: $ $\\<br />Lema 1: Existen n\'umeros trascendentes.\\<br />Dem: facilita, los n\'umeros algebraicos son numerables, pero los reales no.\\<br />$ $\\<br />Sea $t\in\mathbb{R}$ un trascendente cualquiera; entonces claramente la igualdad $\displaystyle\sum_{k=0}^{n}q_{k}t^{k}=0$ no se puede cumplir cuando alguno de los $q_{k}\in\mathbb{Q}$ es no nulo ($t$ ser\'ia algebraico).\\<br />Por tanto $[\mathbb{R}:\mathbb{Q}]=\infty$.<br />$ $\\<br />Espero no se me haya indo nada.$ Mensaje modificado por Kaissa el Nov 7 2009, 08:11 PM -------------------- Este es un extracto de Qué es fmat by Kenshin. La pregunta para ti estimado(a) lector(a) es... ¿Qué haces tu para que ésto se cumpla?

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 7 2009, 08:47 PM Publicado: #5
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	Y bien, he ahí una solución más. Muy buena, Kaissa. -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

daglnn0x0 Ver Perfil del Miembro Ver los mensajes de este miembro	Nov 19 2009, 10:08 AM Publicado: #6
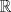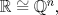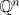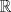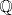Maestro Matemático Grupo: Usuario FMAT Mensajes: 89 Registrado: 30-July 09 Miembro Nº: 56.216 Nacionalidad: Universidad: Sexo:	Más simple aún; si $TEX: $\mathbb{R}$$ tuviera dimensión finita n, tendríamos que $TEX: $\mathbb{R} \cong \mathbb{Q}^n$,$ contradicción pues $TEX: $\mathbb{Q}^n$$ es numerable. De hecho, $TEX: $\mathbb{R}$$ como $TEX: $\mathbb{Q}$$ espacio vectorial no posee una base numerable. 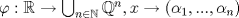De serlo, basta considerar la aplicación inyectiva $TEX: $ \varphi :\mathbb{R}\rightarrow \bigcup_{n\in\mathbb{N}}\mathbb{Q}^n, x \rightarrow (\alpha_{1}, ... 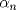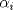,\alpha_{n})$$ , donde n es el mayor entero tal que $TEX: $\alpha_{n}$$ es no nulo y los $TEX: $\alpha_{i}$$ son los coeficientes de los elementos de la base. Como el conjunto de llegada de la función es numerable, se tiene una contradicción.

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 4 2009, 01:25 AM Publicado: #7
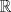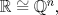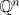Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	CITA(daglnn0x0 @ Nov 19 2009, 10:08 AM) Más simple aún; si $TEX: $\mathbb{R}$$ tuviera dimensión finita n, tendríamos que $TEX: $\mathbb{R} \cong \mathbb{Q}^n$,$ contradicción pues $TEX: $\mathbb{Q}^n$$ es numerable. Excelente respuesta, estimado daglnn0x0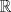... 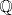CITA(daglnn0x0 @ Nov 19 2009, 10:08 AM) De hecho, $TEX: $\mathbb{R}$$ como $TEX: $\mathbb{Q}$$ espacio vectorial no posee una base numerable. 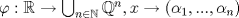De serlo, basta considerar la aplicación inyectiva $TEX: $ \varphi :\mathbb{R}\rightarrow \bigcup_{n\in\mathbb{N}}\mathbb{Q}^n, x \rightarrow (\alpha_{1}, ... 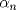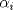,\alpha_{n})$$ , donde n es el mayor entero tal que $TEX: $\alpha_{n}$$ es no nulo y los $TEX: $\alpha_{i}$$ son los coeficientes de los elementos de la base. Como el conjunto de llegada de la función es numerable, se tiene una contradicción. Excelente, excelente, excelente... -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	Mar 24 2010, 10:21 PM Publicado: #8
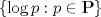Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	Denotemos con P al conjunto de números primos (positivos). 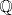Una alternativa de solución más consiste en emplear el TFA para demostrar que el conjunto $TEX: $\{\log p: p \in \mathbf{P} \}$$ es un subconjunto linealmente independiente de los reales (considerando que el campo subyacente al espacio es $TEX: $\mathbb{Q}$$ ). Así, de la infinitud de P se tendría que el grado de la extensión $TEX: $[\mathbb{R}: \mathbb{Q}]$$ no puede ser finito. QED. -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

« Posterior · Álgebra Lineal · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 3rd April 2025 - 09:00 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.