Clasico 21 - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Consultas > Banco de Problemas Resueltos > Álgebra > Sumatorias

Reply to this topic

Start new topic

Clasico 21

XaPi Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 13 2009, 01:52 AM Publicado: #1
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.872 Registrado: 9-March 06 Desde: Welcome Miembro Nº: 614 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	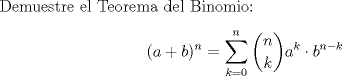$TEX: Demuestre el Teorema del Binomio: $$(a+b)^n = \sum_{k=0}^{n} {n \choose k} a^k \cdot b^{n-k}$$$ Hay varias soluciones Saludos -------------------- USA MAPLE ANTES QUE L'HOPITAL!!!! --- fan ----------------- CURRICULUM VITAE ----------------- 296 pts en la PSU de Matemáticas Admisión 2010. Estudiante de Primer Año de Licenciatura en Historia, Ciencias Sociales y Filosofía Jurídica U. de Talca VII Region Chile

Crash! Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 13 2009, 10:38 AM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 929 Registrado: 22-June 08 Desde: Santiago Miembro Nº: 27.979 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> {\text{Recordando que:}} \hfill \\<br /> \hfill \\<br /> \left( {\begin{array}{{20}c}<br /> n \\<br /> k \\<br /><br /> \end{array} } \right) + \left( {\begin{array}{{20}c}<br /> n \\<br /> {k + 1} \\<br /><br /> \end{array} } \right) = \left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> {k + 1} \\<br /><br /> \end{array} } \right) \hfill \\<br /> \hfill \\<br /> \left( {\begin{array}{{20}c}<br /> n \\<br /> 0 \\<br /><br /> \end{array} } \right) = \left( {\begin{array}{{20}c}<br /> n \\<br /> n \\<br /><br /> \end{array} } \right) = 1\;;\;siendo\;\left( {\begin{array}{{20}c}<br /> n \\<br /> k \\<br /><br /> \end{array} } \right) = \frac{{n!}}<br />{{k!\left( {n - k} \right)!}} \hfill \\<br /> \hfill \\<br /> Por\;Induccion: \hfill \\<br /> \hfill \\<br /> Para\;n = 1\;tenemos \hfill \\<br /> \hfill \\<br /> \left( {a + b} \right)^1 = \sum\limits_{k = 0}^1 {\left( {\begin{array}{{20}c}<br /> 1 \\<br /> k \\<br /><br /> \end{array} } \right)a^k b^{1 - k} } = a + b \hfill \\<br /> \hfill \\<br /> Hipotesis\;de\;induccion: \hfill \\<br /> \hfill \\<br /> \left( {a + b} \right)^n = \sum\limits_{k = 0}^n {\left( {\begin{array}{{20}c}<br /> n \\<br /> k \\<br /><br /> \end{array} } \right)a^k b^{n - k} } \hfill \\<br /> \hfill \\<br /> Por\;demostrar\;que: \hfill \\<br /> \hfill \\<br /> \left( {a + b} \right)^{n + 1} = \sum\limits_{k = 0}^{n + 1} {\left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> k \\<br /><br /> \end{array} } \right)a^k b^{n + 1 - k} } \hfill \\ <br />\end{gathered} <br />\]<br />$ $TEX: \[<br />\begin{gathered}<br /> \left( {a + b} \right)^{n + 1} = \sum\limits_{k = 0}^{n + 1} {\left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> k \\<br /><br /> \end{array} } \right)a^k b^{n + 1 - k} } \hfill \\<br /> \hfill \\<br /> \left( {a + b} \right)^{n + 1} = \left( {a + b} \right)\left( {a + b} \right)^n \hfill \\<br /> \hfill \\<br /> = \left( {a + b} \right)\sum\limits_{k = 0}^n {\left( {\begin{array}{{20}c}<br /> n \\<br /> k \\<br /><br /> \end{array} } \right)a^k b^{n - k} } \hfill \\<br /> \hfill \\<br /> = \left( {a + b} \right)\left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> 0 \\<br /><br /> \end{array} } \right)a^0 b^n + \left( {\begin{array}{{20}c}<br /> n \\<br /> 1 \\<br /><br /> \end{array} } \right)a^1 b^{n - 1} + \cdots + \left( {\begin{array}{{20}c}<br /> n \\<br /> {n - 1} \\<br /><br /> \end{array} } \right)a^{n - 1} b^1 + \left( {\begin{array}{{20}c}<br /> n \\<br /> n \\<br /><br /> \end{array} } \right)a^n b^0 } \right\} \hfill \\<br /> \hfill \\<br /> = \left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> 0 \\<br /><br /> \end{array} } \right)a^1 b^n + \left( {\begin{array}{{20}c}<br /> n \\<br /> 1 \\<br /><br /> \end{array} } \right)a^2 b^{n - 1} + \cdots + \left( {\begin{array}{{20}c}<br /> n \\<br /> {n - 1} \\<br /><br /> \end{array} } \right)a^n b^1 + \left( {\begin{array}{{20}c}<br /> n \\<br /> n \\<br /><br /> \end{array} } \right)a^{n + 1} b^0 } \right\} \hfill \\<br /> + \left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> 0 \\<br /><br /> \end{array} } \right)a^0 b^{n + 1} + \left( {\begin{array}{{20}c}<br /> n \\<br /> 1 \\<br /><br /> \end{array} } \right)a^1 b^n + \cdots + \left( {\begin{array}{{20}c}<br /> n \\<br /> {n - 1} \\<br /><br /> \end{array} } \right)a^{n - 1} b^2 + \left( {\begin{array}{{20}c}<br /> n \\<br /> n \\<br /><br /> \end{array} } \right)a^n b^1 } \right\} \hfill \\<br /> \hfill \\<br /> = \left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> 0 \\<br /><br /> \end{array} } \right)} \right\}a^0 b^{n + 1} + \left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> 0 \\<br /><br /> \end{array} } \right) + \left( {\begin{array}{{20}c}<br /> n \\<br /> 1 \\<br /><br /> \end{array} } \right)} \right\}a^1 b^n + \cdots + \left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> {n - 1} \\<br /><br /> \end{array} } \right) + \left( {\begin{array}{{20}c}<br /> n \\<br /> n \\<br /><br /> \end{array} } \right)} \right\}a^n b^1 \hfill \\<br /> + \left\{ {\left( {\begin{array}{{20}c}<br /> n \\<br /> n \\<br /><br /> \end{array} } \right)} \right\}a^{n + 1} b^0 \hfill \\<br /> \hfill \\<br /> = \left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> 0 \\<br /><br /> \end{array} } \right)a^0 b^{n + 1} + \left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> 1 \\<br /><br /> \end{array} } \right)a^1 b^n + \cdots + \left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> n \\<br /><br /> \end{array} } \right)a^n b^1 + \left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> {n + 1} \\<br /><br /> \end{array} } \right)a^{n + 1} b^0 \hfill \\<br /> \hfill \\<br /> = \sum\limits_{k = 0}^{n + 1} {\left( {\begin{array}{{20}c}<br /> {n + 1} \\<br /> k \\<br /><br /> \end{array} } \right)a^k b^{n + 1 - k} } \hfill \\ <br />\end{gathered} <br />\]<br />$ Mensaje modificado por Emex el Jan 13 2009, 10:41 AM -------------------- Ex-Electrico Usach 2008 Mechón Injenieria 2009 Tengo Sed.

XaPi Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 13 2009, 01:47 PM Publicado: #3
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.872 Registrado: 9-March 06 Desde: Welcome Miembro Nº: 614 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Esta bien. Se esperan mas soluciones. Saludos -------------------- USA MAPLE ANTES QUE L'HOPITAL!!!! --- fan ----------------- CURRICULUM VITAE ----------------- 296 pts en la PSU de Matemáticas Admisión 2010. Estudiante de Primer Año de Licenciatura en Historia, Ciencias Sociales y Filosofía Jurídica U. de Talca VII Region Chile

Pedro² Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 26 2009, 03:16 PM Publicado: #4
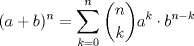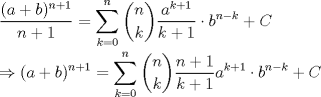Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Otra forma (también por inducción): Notar que $TEX: $\displaystyle (a+b)^n=\sum_{k=0}^n\binom{n}{k}a^k\cdot b^{n-k}$$ implica (integrando respecto a $TEX: $a$$ ): $TEX: \noindent $\displaystyle \dfrac{(a+b)^{n+1}}{n+1}=\sum_{k=0}^n\binom{n}{k}\dfrac{a^{k+1}}{k+1}\cdot b^{n-k}+C\\<br />\\<br /> \Rightarrow (a+b)^{n+1}=\sum_{k=0}^n\binom{n}{k}\dfrac{n+1}{k+1} a^{k+1} \cdot b^{n-k}+C $$ Pero, $TEX: $\displaystyle \binom{n}{k}\dfrac{n+1}{k+1}=\dfrac{(n+1)!}{(k+1)!((n+1)-(k+1))!}=\binom{n+1}{k+1}$$ Así, $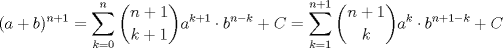TEX: $\displaystyle (a+b)^{n+1}=\sum_{k=0}^n\binom{n+1}{k+1}a^{k+1}\cdot b^{n-k}+C=\sum_{k=1}^{n+1}\binom{n+1}{k}a^k\cdot b^{n+1-k}+C$$ Haciendo 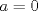$TEX: $a=0$$ , se obtiene que $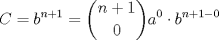TEX: $\displaystyle C=b^{n+1}=\binom{n+1}{0}a^0\cdot b^{n+1-0}$$ . De lo anterior, se concluye que 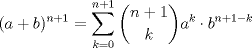$TEX: $\displaystyle (a+b)^{n+1}=\sum_{k=0}^{n+1}\binom{n+1}{k}a^k\cdot b^{n+1-k}$$ , la afirmación sigue por inducción respecto al exponente. -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática* - Mechón 2009* "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy

7words Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 26 2009, 03:51 PM Publicado: #5
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.756 Registrado: 16-September 07 Desde: al frente del Express Miembro Nº: 10.238 Nacionalidad: Universidad: Sexo:	otra forma conocida : sea : $TEX: \[f\left( x \right) = {\left( {a + x} \right)^n}\]$ derivando n veces tenemos que: $TEX: \[{f^n}\left( x \right) = k!\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){\left( {a + x} \right)^{n - k}}\]<br />$ si se hace x=0, tenemos: $TEX: <br />\[{f^n}\left( 0 \right) = k!\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){a^{n - k}}\]$ y si lo utilisamos en el polinomio de taylor queda: $TEX: \[{\left( {a + x} \right)^n} = f\left( x \right) = \sum\limits_{k = 0}^n {\frac{{{f^n}\left( 0 \right)}}<br />{{k!}}{x^k}} = \sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){a^{n - k}}{x^k}} .\]<br />$ -------------------- Ahora van quedando en el foro solo los niñitos tontitos graves, que lata... u.u

Abu-Khalil Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 24 2010, 10:50 PM Publicado: #6
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(7words @ Jul 26 2009, 05:51 PM) otra forma conocida : y si lo utilisamos en el polinomio de taylor queda: $TEX: \[{\left( {a + x} \right)^n} = f\left( x \right) = \sum\limits_{k = 0}^n {\frac{{{f^n}\left( 0 \right)}}<br />{{k!}}{x^k}} = \sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){a^{n - k}}{x^k}} .\]<br />$ Y el error donde queda ? -------------------- ~milopez $TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]$ $TEX: <br />\begin{equation}\begin{aligned}<br />dS_t&=\mu S_t dt+\sqrt{V_t}S_t dW^S_t\\<br />dV_t&=\kappa(\theta-V_t)dt+\sigma\sqrt{V_t} dW^V_t\\<br />\end{aligned}\end{equation}<br />$ $TEX: <br />$$\mathbb A^{\text U}_M(N)$$<br />$

Rurouni Kenshin Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 24 2010, 10:58 PM Publicado: #7
Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad: Sexo:	CITA(7words @ Jul 26 2009, 05:51 PM) otra forma conocida : sea : $TEX: \[f\left( x \right) = {\left( {a + x} \right)^n}\]$ derivando k veces ( 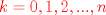$TEX: $\color{red}k=0,1,2,...,n$$ ) tenemos que: $TEX: \[{f^{\color{red}k}}\left( x \right) = k!\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){\left( {a + x} \right)^{n - k}}\]<br />$ si se hace x=0, tenemos: $TEX: <br />\[{f^{\color{red}k}}\left( 0 \right) = k!\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){a^{n - k}}\]$ y si lo utilizamos en el polinomio de taylor queda: $TEX: \[{\left( {a + x} \right)^n} = f\left( x \right) = \sum\limits_{k = 0}^n {\frac{{{f^{\color{red}k}}\left( 0 \right)}}<br />{{k!}}{x^k}} = \sum\limits_{k = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> k \hfill \\ <br />\end{gathered} \right){a^{n - k}}{x^k}} .\]<br />$ Ahi le edite algunos detallitos de tipeo, además de que hay que agregar [observacion de Abu-Khalil] que el Error es Cero, pues 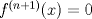$TEX: $f^{(n+1)}(x)=0$$ (en particular lo es en cualquier $TEX: $\xi\in [0,x]$$ ). Saludos -------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!) Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)

Leonardo Maurici... Leonardo Mauricio Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 24 2010, 10:59 PM Publicado: #8
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.244 Registrado: 11-October 09 Desde: Santiago Miembro Nº: 60.148 Nacionalidad: Universidad: Sexo:	Esta la aprendi en la escuela de verano, es la misma ke hizo el profe http://materias.unq.edu.ar/pye/Notas%20de%..._Bin_Newton.pdf -------------------- CITA(Marcel Claude @ Oct 13 2013, 22:10 PM) Venezuela es más democrático que Chile XD CITA(luismema @ Dec 6 2012, 05:48 PM) CITA(Exorcista @ Dec 6 2012, 05:39 PM) x1000 ... a tipos como este me refiero cuando hablo de ''ingenieros beauchef frustrados'', que lata...... compadre vaya a la escuela de psicologia de la U, ya que le queda cerquita y pide hora, por que de verdad del tiempo que he estado en el foro, usted no aporta mucha. Pd: ademas hablas y discriminas a la gente de otra U como si las conocieras, tu no pareces ser de la usach. Si aveiguo algo que te sirva respecto a lo de salud, te lo informo. CITA(master_c @ Sep 23 2012, 12:11 PM) (a Kaissa) 6528 post sin decir nada, solo criticar en un foro que es para ayudar, proponer y resolver (compartir conocimiento) y me hablas de malgastar la vida :death: CITA(clowny @ Sep 11 2012, 03:45 PM) jajajajajja, no me voy a rebajar a pelear con alguien dice que usa mac en su pc (si mac) jajajaja. win2 va a caher junto a mac tarde o temprano, esa es mi opinión :) CITA(Kura @ Jun 7 2012, 01:13 PM) El mecanismo de evaluación es así, obtener buenas notas es posible aprovechando los bugs del sistema. CITA(Gastón Burrull @ Apr 27 2012, 11:15 PM) CITA(clowny @ Apr 27 2012, 11:10 PM) Sería re fome el mundo lleno de físicos y matemáticos... Seguro que tienes internet y computadora gracias a la numerología... CITA(VA Jiménez @ Dec 28 2011, 04:28 PM) ya que hablas de mediocridad, el criticar eso es, por decir lo menos, mediocre. CITA(VA Jiménez @ Dec 28 2011, 04:28 PM) y que te rías de eso me parece bastante penoso, Mr. "Estoy haciendo un doctorado y lo menciono en 5 de cada 6 post" (dirigido a Sansanito) CITA(anonimo @ Dec 28 2011, 04:35 PM) pd: xD he leido muuchos post de Sansanito y recién ahora sé que estudia un doctorado xD (y eso que hemos hablado por pm) CITA(clowny @ Jan 27 2011, 09:35 PM) ...hablé de que el liberalismo de smith en alguna parte era mejor que el neoliberalismo, y se mal interpretó con eso... RE: Simplemente en ninguna parte.... CITA(clowny @ Jan 27 2011, 09:43 PM) Imaginate llevan al mod, a mxmaster y a yarly a hablar del modelo economico moderno ohmy.gif! RE: Me imagino a clowny en las mismas... CITA(El Geek @ Jan 27 2012, 01:57 PM) Notable que tu siendo un chanta que no salva a nadie (porque en el foro no has mostrado jamás tener alguna destreza con mate sino que puro blablá) sigas viniendo a trollear dándoteas de capo.

Kaissa Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 24 2010, 11:02 PM Publicado: #9
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 9.897 Registrado: 6-April 08 Miembro Nº: 19.238 Nacionalidad: Colegio/Liceo: Sexo:	escriban la solucion con combinatoria, a mi gusto la mas bacan de todas. -------------------- Este es un extracto de Qué es fmat by Kenshin. La pregunta para ti estimado(a) lector(a) es... ¿Qué haces tu para que ésto se cumpla?

Kaissa Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 25 2010, 01:46 PM Publicado: #10
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 9.897 Registrado: 6-April 08 Miembro Nº: 19.238 Nacionalidad: Colegio/Liceo: Sexo:	bueno... ya que nadie me pesco aqui voy con una solucion poco comun, pero mas bacan que esa induccion (a mi gusto la uinduccion es como "compruebe la formula" pero no te dice como llegar) $TEX: $ $\\<br />Como el(la) lector(a) habr\'a notado, queremos hallar <br />\begin{eqnarray}<br />(a+b)^{n}=(a+b)\times(a+b)\times\cdots\times(a+b)<br />\end{eqnarray} <br />$n$ veces repetido el factor.\\<br />$\bullet$ El mayor exponente que obtendremos de $a$ es $n$ y se obtiene eligiendo la $a$ de cada factor, lo cual puede hacerse de 1 sola forma, as\'i que su coeficiente es 1. (an\'alogamente con $b$).\\<br />$\bullet$ Sea $1\leq k\leq n-1$, si quisi\'eramos seleccionar $k$ $a$-es de estos $n$ par\'entesis, quedar\'ian $n-k$ $b$-es en los siguientes. La pregunta del mill\'on es: De cu\'antas maneras puedo elegir $k$ $a$-es de un conjunto con $n$ $a$-es?.\\<br />La respuesta es obvia, y la tiene la sra combinatoria, en efecto son $\displaystyle\binom{n}{k}$ formas.\\<br />Hemos entonces demostrado que el coeficiente de $a^{n}$ y $b^{n}$ son ambos 1, y que dado $1\leq k\leq n-1$ el coeficiente de $a^{k}b^{n-k}$ es $\displaystyle\binom{n}{k}$.\\<br />Nos queda \'unicamente escribirlo como sumatoria, pero eso es f\'acil observando que $1=\displaystyle\binom{n}{0}=\displaystyle\binom{n}{n}$ y que $\displaystyle\binom{n}{k}=\displaystyle\binom{n}{n-k}$ por lo que todos los t\'erminos se pueden conectar en una forma general mediante la formulita conocida<br />\begin{eqnarray}<br />(a+b)^{n}=\displaystyle\sum_{k=0}^{n}\binom{n}{k}a^{n-k}b^{k}<br />\end{eqnarray}<br />$ Mensaje modificado por Kaissa el Jan 25 2010, 01:48 PM -------------------- Este es un extracto de Qué es fmat by Kenshin. La pregunta para ti estimado(a) lector(a) es... ¿Qué haces tu para que ésto se cumpla?

« Posterior · Sumatorias · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 26th April 2025 - 12:07 AM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.