|
|
|
|
|
|
|
  |
 Dec 22 2008, 06:07 PM Dec 22 2008, 06:07 PM
Publicado:
#1
|
|
|
Maestro Matemático Grupo: Usuario FMAT Mensajes: 124 Registrado: 27-June 08 Desde: Las Condes, Santiago. Miembro Nº: 28.486 Nacionalidad:  Sexo:  |
Buenas tardes...
Mientras hacia un ejercicio de calcular el area de un astroide..me asalto una duda con los limites de integracion...a ver si me ayudan =) dice: ![TEX: Considere el astroide dado por la curva parametrica<br />\[<br />\begin{gathered}<br /> x(t) = a^3 \cos ^3 t \hfill \\<br /> y(t) = a^3 sen^3 t \hfill \\ <br />\end{gathered} <br />\]<br />\[<br />a \geqslant 0<br />\]<br /><br /><br />Bueno para calcular el area hice..<br /><br />\[<br />4\int\limits_0^a {\left| {y(t)} \right|dx(t)} <br />\]<br /><br /><br />Integre desde el origen hasta un "a" que representa uno de los vertices del astroide...y lo multiplico por 4 en virtud de las simetrias de la figura...<br /><br />luego la integral queda como:<br /><br />\[<br />4\int\limits_0^a {a^3 sen^3 (t)( - 3a^3 Cos^2 (t)} Sen(t))dt<br />\]<br /><br />Pero para determinar los limites quede plop...<br /><br />pues tengo que:<br /><br />si \[<br />x = 0 \Rightarrow a^3 Cos^3 (t) = 0 \Leftrightarrow Cos(t) = 0 \Leftrightarrow t = \frac{\pi }<br />{2}<br />\]<br /><br />y para el otro:<br /><br />\[<br />x = a \Rightarrow a^3 Cos^3 (t) = a \Leftrightarrow Cos^3 (t) = a^{ - 2} ??<br />\]<br /><br />Y eso no se como expresarlo..<br /><br />Espero no haberme equivocado en como tomar los limites de integracion..bueno eso seria mi duda a ver si me dan una mano porfa<br /><br /><br />Nota: a mayor que cero y constante<br /><br />](./tex/5f8ef99d93184c7ef2eab3e833fa9ede.png) Gracias <3 Mensaje modificado por Estor el Dec 22 2008, 06:17 PM -------------------- Perseverar da el triunfo.
carli<3  |
|
|
|
 Dec 22 2008, 07:39 PM Dec 22 2008, 07:39 PM
Publicado:
#2
|
|
 Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Está correcto lo de las simetrías, pero si te fijas el parámetro "t" está variando desde
 a a  . Como estamos integrando respecto al eje X, el límite inferior de integración será cuando . Como estamos integrando respecto al eje X, el límite inferior de integración será cuando 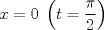 y el superior para y el superior para 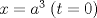 . Luego la integral debe ser: . Luego la integral debe ser:![TEX: \[<br />\begin{gathered}<br /> I = 4\int_{t_0 }^{t_1 } {y(t)\;x'(t)\;dt = 4\int_{\pi /2}^0 {a^3 \sin ^3 t \cdot \left( { - 3a^3 \sin t\cos ^2 t} \right)} } \;dt \hfill \\<br /> I = 12a^6 \int_0^{\pi /2} {\sin ^4 t\cos ^2 t\;dt} \hfill \\<br /> {\textsf{Esta integral se puede evaluar por metodos convencionales o usando la funcion Beta:}} \hfill \\<br /> I = 6a^6\beta \left( {\frac{5}<br />{2},\frac{3}<br />{2}} \right) = 6a^6 \cdot \frac{1}<br />{{3!}} \cdot \frac{{\sqrt \pi }}<br />{2} \cdot \frac{{3\sqrt \pi }}<br />{4} = \frac{3\pi }<br />{8} \hfill \\<br /> \Rightarrow I = \frac{{3\pi }}<br />{8} \cdot a^6 \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/ff57f08e99b3504d249cdc94751ab9bd.png) Por lo menos yo lo aprendí así xD Saludos! Mensaje modificado por Pedro² el Dec 23 2008, 12:02 AM -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática - Mechón 2009 "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy |
|
|
|
 Dec 22 2008, 07:49 PM Dec 22 2008, 07:49 PM
Publicado:
#3
|
|
|
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 193 Registrado: 31-October 08 Desde: Bogotá, Colombia Miembro Nº: 37.497 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
|
|
|
|
 Dec 22 2008, 07:51 PM Dec 22 2008, 07:51 PM
Publicado:
#4
|
|
|
Maestro Matemático Grupo: Usuario FMAT Mensajes: 124 Registrado: 27-June 08 Desde: Las Condes, Santiago. Miembro Nº: 28.486 Nacionalidad:  Sexo:  |
esa misma era mi duda...de hecho inicialmente iba a integrar de
![TEX: \[<br />[0,\frac{\pi }<br />{2}]<br />\]<br />](./tex/581bd4472e49400f35e6613e08c43dd7.png) Pero a secas...vale gracias =)  Mensaje modificado por Estor el Dec 22 2008, 07:53 PM -------------------- Perseverar da el triunfo.
carli<3  |
|
|
|
 Dec 22 2008, 07:55 PM Dec 22 2008, 07:55 PM
Publicado:
#5
|
|
|
Maestro Matemático Grupo: Usuario FMAT Mensajes: 124 Registrado: 27-June 08 Desde: Las Condes, Santiago. Miembro Nº: 28.486 Nacionalidad:  Sexo:  |
Está correcto lo de las simetrías, pero si te fijas el parámetro "t" está variando desde  a a  . Como estamos integrando respecto al eje X, el límite inferior de integración será cuando . Como estamos integrando respecto al eje X, el límite inferior de integración será cuando 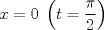 y el superior para y el superior para 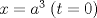 . .Ahi como q me perdi un poco :/ -------------------- Perseverar da el triunfo.
carli<3  |
|
|
|
 Dec 22 2008, 07:56 PM Dec 22 2008, 07:56 PM
Publicado:
#6
|
|
 Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Ahi como q me perdi un poco :/ Imaginate el gráfico. Uno va barriendo el área desde 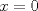 a a  , ahora ve que valores de "t" le corresponden a esos "x". , ahora ve que valores de "t" le corresponden a esos "x".
-------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática - Mechón 2009 "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy |
|
|
|
 Dec 22 2008, 07:59 PM Dec 22 2008, 07:59 PM
Publicado:
#7
|
|
|
Maestro Matemático Grupo: Usuario FMAT Mensajes: 124 Registrado: 27-June 08 Desde: Las Condes, Santiago. Miembro Nº: 28.486 Nacionalidad:  Sexo:  |
no si entiendo la idea pero como q no me quiere entrar que es hasta
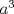 :x :x
-------------------- Perseverar da el triunfo.
carli<3  |
|
|
|
 Dec 22 2008, 08:05 PM Dec 22 2008, 08:05 PM
Publicado:
#8
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.920 Registrado: 19-August 06 Desde: DIM, DCC Beauchef Miembro Nº: 1.989 Nacionalidad:  Universidad:  Sexo:  |
Quizás el siguiente enlace podría resolver tu duda.
Mensaje modificado por neo shykerex el Dec 22 2008, 08:06 PM -------------------- Miembro de Anime No Seishin Doukokai, podrías ser el próximo.
|
|
|
|
 Dec 22 2008, 08:09 PM Dec 22 2008, 08:09 PM
Publicado:
#9
|
|
 Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Por esto:
 tr8astroide.gif ( 5.59k )
Número de descargas: 14
tr8astroide.gif ( 5.59k )
Número de descargas: 14Tiene que ver por como definiste tu curva, si hubiera sido, por ejemplo, 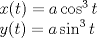 El punto P sería (a,0) -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática - Mechón 2009 "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy |
|
|
|
 Dec 22 2008, 08:30 PM Dec 22 2008, 08:30 PM
Publicado:
#10
|
|
|
Maestro Matemático Grupo: Usuario FMAT Mensajes: 124 Registrado: 27-June 08 Desde: Las Condes, Santiago. Miembro Nº: 28.486 Nacionalidad:  Sexo:  |
clarisimo!
vale gracias! -------------------- Perseverar da el triunfo.
carli<3  |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 14th October 2025 - 09:21 PM |









