Identificarse Registrarse

Psu
Enseñanza Básica
Enseñanza Media
Universidad
Olimpiadas
Comunidad



 
 Reply to this topicStart new topic
> Ecuacion irracional
xdanielx
mensaje Dec 9 2008, 08:02 PM
Publicado: #1


Dios Matemático Supremo


Grupo: Baneado
Mensajes: 3.360
Registrado: 11-March 08
Miembro Nº: 16.617
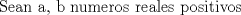TEX: Sean a, b numeros reales positivos

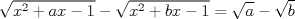
TEX: $$<br />\sqrt {x^2 + ax - 1} - \sqrt {x^2 + bx - 1} = \sqrt a - \sqrt b <br />$$











TEX: saludos

Mensaje modificado por vivanco el Dec 9 2008, 08:17 PM
Go to the top of the page
 
+Quote Post
El_Feo
mensaje Apr 23 2020, 08:45 PM
Publicado: #2


Principiante Matemático
Ícono de Grupo

Grupo: Usuario FMAT
Mensajes: 5
Registrado: 22-April 20
Miembro Nº: 165.119
Nacionalidad:
Sexo:



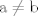Supongamos TEX: $\mathrm{a\neq b}$, de lo contrario todo número real es solución.

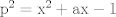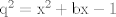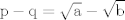Sean TEX: $\mathrm{p^2=x^2+ax-1}$ y TEX: $\mathrm{q^2=x^2+bx-1}$. De este modo, TEX: $\mathrm{p - q = \sqrt{a}-\sqrt{b}}$.

Por otro lado 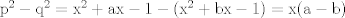TEX: $\mathrm{p^2-q^2=x^2+ax-1-(x^2+bx-1)=x(a-b)}$. Luego,

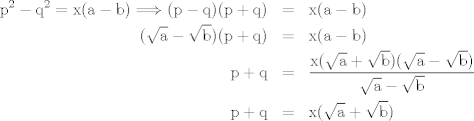TEX: <br />\begin{eqnarray*}<br />\mathrm{p^2-q^2}=\mathrm{x(a-b)} \Longrightarrow \mathrm{(p-q)(p+q)} &=& \mathrm{ x(a-b)}\\<br />\mathrm{(\sqrt{a}-\sqrt{b})(p+q)} &=& \mathrm{x(a-b)}\\<br />\mathrm{p+q} &=& \mathrm{\displaystyle\frac{x(\sqrt{a}+\sqrt{b})(\sqrt{a}-\sqrt{b})}{\sqrt{a}-\sqrt{b}}}\\<br />\mathrm{p+q} &=& \mathrm{x(\sqrt{a}+\sqrt{b})}<br />\end{eqnarray*}<br />

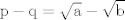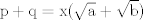Por lo tanto, como TEX: $\mathrm{p - q = \sqrt{a}-\sqrt{b}}$ y TEX: $\mathrm{p+q = x(\sqrt{a}+\sqrt{b})}$, al sumar ambas igualdades
y dividir por 2 se tiene que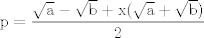

TEX: <br />$$\mathrm{p=\displaystyle\frac{\mathrm{\sqrt{a}-\sqrt{b}}+x(\sqrt{a}+\sqrt{b})}{2}}$$


Ahora, reemplazamos p en la igualdad TEX: $\mathrm{p^2=x^2+ax-1}$, de donde resulta una ecuación en x.
En efecto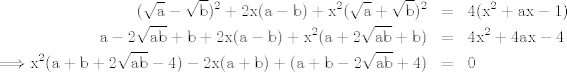

TEX: <br />\begin{eqnarray*}<br />\mathrm{(\sqrt{a}-\sqrt{b})^2+2x(a-b)+x^2(\sqrt{a}+\sqrt{b})^2} &=& \mathrm{4(x^2+ax-1)}\\<br />\mathrm{a-2\sqrt{ab}+b + 2x(a-b)+x^2(a+2\sqrt{ab}+b)} &=& \mathrm{4x^2+4ax-4}\\<br />\Longrightarrow \mathrm{x^2(a+b+2\sqrt{ab}-4)-2x(a+b)+ (a+b-2\sqrt{ab}+4)} &=& 0<br />\end{eqnarray*}<br />

El discriminante del ecuación es

TEX: <br />\begin{eqnarray*}<br />&& \mathrm{4(a+b)^2 - 4[(a+b)+(2\sqrt{ab}-4)] [(a+b)-(2\sqrt{ab}-4)]} \\<br />&=& \mathrm{4(a+b)^2-4[(a+b)^2-(2\sqrt{ab}-4)^2]}\\<br />&=& \mathrm{4(2\sqrt{ab}-4)^2}<br />\end{eqnarray*}<br />

Luego, las raíces son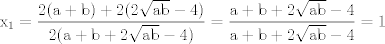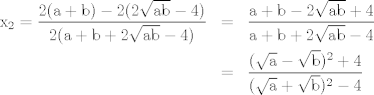

TEX: <br />$$\mathrm{x_1=\displaystyle\frac{2(a+b)+2(2\sqrt{ab}-4)}{2(a+b+2\sqrt{ab}-4)}=\displaystyle\frac{a+b+2\sqrt{ab}-4}{a+b+2\sqrt{ab}-4}=1}$$

TEX: <br />\begin{eqnarray*}<br />\mathrm{x_2}=\mathrm{\displaystyle\frac{2(a+b)-2(2\sqrt{ab}-4)}{2(a+b+2\sqrt{ab}-4)}}&=&\mathrm{\displaystyle\frac{a+b-2\sqrt{ab}+4}{a+b+2\sqrt{ab}-4}}\\<br />&=&\mathrm{\displaystyle\frac{(\sqrt{a}-\sqrt{b})^2+4}{(\sqrt{a}+\sqrt{b})^2-4}}<br />\end{eqnarray*}<br />

Donde es necesaria la condición 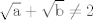TEX: $\mathrm{\sqrt{a}+\sqrt{b} \neq 2}$.
Go to the top of the page
 
+Quote Post
 

Reply to this topicStart new topic
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):

 

Versión Lo-Fi Fecha y Hora actual: 6th March 2025 - 09:47 PM
Powered By IP.Board 2.2.1 © 2007  IPS, Inc.