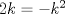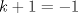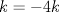|
|
|
|
|
|
|
  |
 Oct 25 2008, 01:06 AM Oct 25 2008, 01:06 AM
Publicado:
#1
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[<br />\begin{gathered}<br /> {\text{Control N1 :}} \hfill \\<br /> \hfill \\<br /> {\text{I}}{\text{. Sean A}}{\text{,B}}{\text{,X}} \in M_2 \left( \mathbb{R} \right){\text{, donde B es una matriz no singular y se cumple}} \hfill \\<br /> {\text{que : }}B^t \cdot X^t = \left( {A - X \cdot B} \right)^t {\text{ }}{\text{. Si }}A = \left( {\begin{array}{*{20}c}<br /> 1 & 0 \\<br /> 3 & 1 \\<br /><br /> \end{array} } \right),B = \left( {\begin{array}{*{20}c}<br /> 1 & 0 \\<br /> 3 & 2 \\<br /><br /> \end{array} } \right){\text{ }}{\text{, encuentre la}} \hfill \\<br /> {\text{matriz X }}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{II}}{\text{. Sea }}A \in M_n \left( \mathbb{R} \right){\text{ }}{\text{. Calcule el o los valores de }}k{\text{ para que la matriz :}} \hfill \\<br /> A = \left( {\begin{array}{*{20}c}<br /> 0 & {2k} & 1 \\<br /> {k^2 } & 0 & {4k} \\<br /> {k + 1} & k & 0 \\<br /><br /> \end{array} } \right){\text{ sea antisimetrica }}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{III}}{\text{. Determine la matriz A }}{\text{, dada la ecuacion :}} \hfill \\<br /> \left( {\begin{array}{*{20}c}<br /> 1 & 0 & 0 \\<br /> 0 & 2 & 0 \\<br /> 0 & 0 & { - 3} \\<br /><br /> \end{array} } \right) \cdot A \cdot \left( {\begin{array}{*{20}c}<br /> 1 & 0 & 0 \\<br /> 0 & 0 & 1 \\<br /> 0 & 1 & 0 \\<br /><br /> \end{array} } \right) = \left( {\begin{array}{*{20}c}<br /> 2 & 3 & { - 1} \\<br /> { - 6} & 0 & {10} \\<br /> {18} & 3 & 0 \\<br /><br /> \end{array} } \right) \hfill \\<br /> \hfill \\<br /> {\text{IV}}{\text{. Demuestre que la matriz }}A = \left( {\begin{array}{*{20}c}<br /> {\cos \phi } & {\sin \phi } & 0 \\<br /> { - \sin \phi } & {\cos \phi } & 0 \\<br /> 0 & 0 & 1 \\<br /><br /> \end{array} } \right){\text{ es invertible para}} \hfill \\<br /> {\text{cualquier valor de }}\phi {\text{ y encuentre A}}^{ - 1} \hfill \\ <br />\end{gathered} <br />\]](./tex/d65f254afeba0b1a133275af129e4e18.png)
-------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Oct 25 2008, 01:11 AM Oct 25 2008, 01:11 AM
Publicado:
#2
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[<br />\begin{gathered}<br /> {\text{Control N2 :}} \hfill \\<br /> \hfill \\<br /> {\text{I}}{\text{. Resuelva la siguiente ecuacion :}} \hfill \\<br /> \left| {\begin{array}{*{20}c}<br /> 1 & 1 & 1 & 1 \\<br /> x & a & 0 & 0 \\<br /> x & 0 & b & 0 \\<br /> x & 0 & 0 & c \\<br /><br /> \end{array} } \right| = 0 \hfill \\<br /> \hfill \\<br /> {\text{II}}{\text{. Dada la matriz }}A = \left( {\begin{array}{*{20}c}<br /> 1 & 2 & {a + b - 1} \\<br /> 5 & { - 13} & {5a - 2b} \\<br /> 3 & { - 7} & a \\<br /> 1 & 1 & b \\<br /><br /> \end{array} } \right){\text{ }}{\text{, con : }}a,b \in \mathbb{R}{\text{ }}{\text{. Determine }} \hfill \\<br /> {\text{los valores de }}a,b{\text{ de modo que la matriz A tenga rango 2 }}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{III}}{\text{. Calcule la inversa de la matriz B}} = \left( {\begin{array}{*{20}c}<br /> {\text{1}} & {\text{1}} & {\text{2}} \\<br /> {\text{0}} & {\text{4}} & {\text{1}} \\<br /> {\text{1}} & {\text{3}} & {\text{0}} \\<br /><br /> \end{array} } \right){\text{ Por el metodo de la}} \hfill \\<br /> {\text{matriz adjunta }}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{IV}}{\text{. Calcule }}\left| A \right|{\text{ }}{\text{, si : }}A = \left( {\begin{array}{*{20}c}<br /> 1 & 2 & 3 & 4 \\<br /> 1 & 3 & 6 & {10} \\<br /> 1 & 4 & {10} & {20} \\<br /> 1 & 5 & {15} & {35} \\<br /><br /> \end{array} } \right) \hfill \\ <br />\end{gathered} <br />\]](./tex/6a925a3906bbf46b38977147eff2e7f5.png)
-------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Oct 25 2008, 01:16 AM Oct 25 2008, 01:16 AM
Publicado:
#3
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[<br />\begin{gathered}<br /> {\text{Control N3 :}} \hfill \\<br /> \hfill \\<br /> {\text{I}}{\text{. Dado el sistema : }} \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> 3x - \lambda y + 2z = \lambda - 1 \hfill \\<br /> 2x - 5y + 3z = 1 \hfill \\<br /> x + 3y - \left( {\lambda - 1} \right)z = 0 \hfill \\ <br />\end{gathered} \,}}\! \right| \hfill \\<br /> {\text{Encuentre el valor de }}\lambda \in \mathbb{R}{\text{ de modo que el sistema :}} \hfill \\<br /> a){\text{Tenga infinitas soluciones}} \hfill \\<br /> b){\text{Sea incompatible}} \hfill \\<br /> c){\text{Tenga solucion unica}} \hfill \\<br /> \hfill \\<br /> {\text{II}}{\text{. Resolver el sistema por el metodo LU :}} \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> 2x_1 + 4x_2 + 6x_3 = 18 \hfill \\<br /> 4x_1 + 5x_2 + 6x_3 = 24 \hfill \\<br /> 3x_1 + x_2 - 2x_3 = 4 \hfill \\ <br />\end{gathered} \,}}\! \right| \hfill \\<br /> \hfill \\<br /> {\text{III}}{\text{. El siguiente sistema tiene solucion unica :}} \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> ay + bx = c \hfill \\<br /> cx + az = b \hfill \\<br /> bz + cy = a \hfill \\ <br />\end{gathered} \,}}\! \right| \hfill \\<br /> {\text{Demuestre que }}abc \ne 0{\text{ y encuentre la solucion }}{\text{.}} \hfill \\<br /> \hfill \\<br /> {\text{IV}}{\text{. Resolver el sistema mediante Cramer :}} \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> 5x - 3t + 2z + w = 0 \hfill \\<br /> x + t + 4z - w = 0 \hfill \\<br /> 3x - t + z - 2w = 0 \hfill \\<br /> 2x - 2y - 3z - w = 0 \hfill \\ <br />\end{gathered} \,}}\! \right| \hfill \\ <br />\end{gathered} <br />\]](./tex/f0fcc7aa8d016725ee3064ad2fc39f97.png)
-------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Oct 25 2008, 10:09 AM Oct 25 2008, 10:09 AM
Publicado:
#4
|
|
 Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 3.812 Registrado: 4-November 07 Desde: Santiago Miembro Nº: 12.213 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
C1) 4)
![TEX: \noindent Calculando determinante por el método de cofactores de $A$, utilizemos la 3era fila y nos queda que el determinante está dado por <br />\[\cos^2\phi+\sin^2\phi =1 \]<br />Por lo tanto la matriz es invertible. Por otro lado, sabemos que la inversa $X$ debe ser de $3\times 3$ y debe cumplir que<br />\[AX = I\]<br />Lo cual nos lleva a la matriz ampliada<br />\begin{equation*}\begin{aligned}<br />\left[\begin{tabular}{c c c | c c c}<br />$\cos\phi$&$\sin\phi$&$0$&$1$&$0$&$0$\\<br />$-\sin\phi$&$\cos\phi$&$0$&$0$&$1$&$0$\\<br />$0$&$0$&$1$&$0$&$0$&$1$<br />\end{tabular}<br />\right]<br />\sim<br />\left[\begin{tabular}{c c c | c c c}<br />$\cos\phi$&$\sin\phi$&$0$&$1$&$0$&$0$\\<br />$0$&$\sec\phi$&$0$&$\tan \phi$&$1$&$0$\\<br />$0$&$0$&$1$&$0$&$0$&$1$<br />\end{tabular}<br />\right]\\<br />\sim<br />\left[\begin{tabular}{c c c | c c c}<br />$\cos\phi$&$0$&$0$&$1-\sin^2\phi$&$-\cos\phi\sin\phi$&$0$\\<br />$0$&$\sec\phi$&$0$&$\tan \phi$&$1$&$0$\\<br />$0$&$0$&$1$&$0$&$0$&$1$<br />\end{tabular}<br />\right]<br />\sim<br />\left[\begin{tabular}{c c c | c c c}<br />$1$&$0$&$0$&$\cos\phi$&$-\sin\phi$&$0$\\<br />$0$&$1$&$0$&$\sin \phi$&$\cos\phi$&$0$\\<br />$0$&$0$&$1$&$0$&$0$&$1$<br />\end{tabular}<br />\right]<br />\end{aligned}\end{equation*}<br />Luego <br />\[X=\left[\begin{tabular}{c c c}<br />$\cos\phi$&$-\sin\phi$&$0$\\<br />$\sin \phi$&$\cos\phi$&$0$\\<br />$0$&$0$&$1$<br />\end{tabular}<br />\right].\]<br />](./tex/c31a5aa603718708d5c33f514fed37ab.png)
-------------------- |
|
|
|
 Oct 25 2008, 02:05 PM Oct 25 2008, 02:05 PM
Publicado:
#5
|
|
 Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 1.067 Registrado: 11-September 07 Desde: Coquimbo Miembro Nº: 10.097 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
C1- 4) Posteado y resuelto también AQUÍ.
--------------------  "¿Qué es la vida? Una ilusión, una sombra, una ficción, y el mayor bien es pequeño: que toda la vida es sueño, y los sueños, sueños son." |
|
|
|
 Oct 26 2008, 07:29 PM Oct 26 2008, 07:29 PM
Publicado:
#6
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[<br />\begin{gathered}<br /> {\text{Control 1 }}{\text{, pregunta 1 :}} \hfill \\<br /> \boxed{{\text{Solucion :}}} \hfill \\<br /> {\text{Trabajando la igualdad :}} \hfill \\<br /> \left( {B^t \cdot X^t } \right) = \left( {X \cdot B} \right)^t = \left( {A - X \cdot B} \right)^t \hfill \\<br /> \Rightarrow X \cdot B = A - X \cdot B \hfill \\<br /> \Rightarrow 2X \cdot B = A \hfill \\<br /> {\text{Como }}B{\text{ es no singular }}{\text{, entonces existe }}B^{ - 1} {\text{ asi :}} \hfill \\<br /> \Rightarrow 2X = A \cdot B^{ - 1} \hfill \\<br /> \Rightarrow X = \frac{1}<br />{2} \cdot \left( {A \cdot B^{ - 1} } \right) \hfill \\<br /> \hfill \\<br /> {\text{Luego la inversa de B es : }}\frac{{\text{1}}}<br />{{\text{2}}}\left( {\begin{array}{*{20}c}<br /> {\text{2}} & {\text{0}} \\<br /> { - 3} & 1 \\<br /><br /> \end{array} } \right) \hfill \\<br /> {\text{Por lo tanto :}} \hfill \\<br /> X = \frac{1}<br />{4} \cdot \left\{ {\left( {\begin{array}{*{20}c}<br /> 1 & 0 \\<br /> 3 & 1 \\<br /><br /> \end{array} } \right)\left( {\begin{array}{*{20}c}<br /> {\text{2}} & {\text{0}} \\<br /> { - 3} & 1 \\<br /><br /> \end{array} } \right)} \right\} = \frac{1}<br />{4} \cdot \left( {\begin{array}{*{20}c}<br /> 2 & 0 \\<br /> 3 & 1 \\<br /><br /> \end{array} } \right) \hfill \\<br /> \Rightarrow X = \left( {\begin{array}{*{20}c}<br /> {\frac{1}<br />{2}} & 0 \\<br /> {\frac{3}<br />{4}} & {\frac{1}<br />{4}} \\<br /><br /> \end{array} } \right) \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/afda3c9a059fd719dfc150f332d0050a.png)
-------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Dec 6 2011, 01:36 PM Dec 6 2011, 01:36 PM
Publicado:
#7
|
|
|
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 2.818 Registrado: 3-October 09 Miembro Nº: 59.773 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Control 1, pregunta 2.
Como es sabido, una matriz antisimétrica se caracteriza por ![TEX: \[({a_{ij}}) = - ({a_{ji}})\]](/tex-image/81909b20cdffbbc87ce5fe537e5461bd.png) , dado esto tenemos entonces 3 casos: , dado esto tenemos entonces 3 casos:Cuando se cumple que 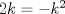 , se obtiene , se obtiene 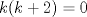 , entonces , entonces  , ó , ó  . .Cuando se cumple que  , se obtiene , se obtiene  . Por último, cuando se obtiene que . Por último, cuando se obtiene que 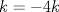 se obtiene que se obtiene que 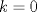 . .Por lo tanto, los valores posibles de 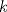 , para que la matriz sea antisimétrica son , para que la matriz sea antisimétrica son ![TEX: \[\{ - 2,0\} \]](/tex-image/5355eef2bd3dfcbd7aaa34c8a2edde91.png) Saludos. -------------------- Me voy, me jui.
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 27th October 2025 - 09:15 PM |









![TEX: \[\mathfrak{L}=\int_{-\infty}^\infty e^{-289x^2}dx=\frac{\Gamma (\frac{1}{2})}{17}=\frac{\sqrt{\pi}}{17}\]](./tex/e0e88dba678ca3ead120b088f41191b2.png)