Derivatón Uach - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Austral de Chile > Cálculo I

10 Páginas:

« < 5 6 7 8 9 > »

Reply to this topic

Start new topic

Derivatón Uach, versión de prueba

Erwin and Fire Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 06:54 PM Publicado: #61
Principiante Matemático Grupo: Usuario FMAT Mensajes: 11 Registrado: 4-April 08 Miembro Nº: 18.992 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> Sea\,f(x) = \sqrt {x\,} \,\,;\,\,\,x_0 = 1\,;\,\,\Delta x = \alpha \hfill \\<br /> f(x_0 + \Delta x) = \sqrt {\alpha + 1} \hfill \\<br /> f'(x) = \frac{1}<br />{{2\sqrt x }} \to f'(x_0 ) = \frac{1}<br />{2} \hfill \\<br /> \Delta y = f(x_0 + \Delta x) - f(x_0 )\, \Rightarrow \Delta y = \sqrt {\alpha + 1} - 1 \hfill \\<br /> dy = f'(x_0 )\Delta x = \frac{\alpha }<br />{2} \hfill \\<br /> \Delta y \approx dy \Rightarrow \sqrt {\alpha + 1} - 1 \approx \frac{\alpha }<br />{2} \hfill \\<br /> \therefore \sqrt {\alpha + 1} \approx \frac{\alpha }<br />{2} + 1 \hfill \\ <br />\end{gathered} <br />\]$ me falto poner esto en la segunda linea XD $TEX: \[<br />f(x_0 + \Delta x) \approx f(x_0 ) + f'(x_0 )\Delta x<br />\]$ si me equivoco, avisen Mensaje modificado por Erwin and Fire el Oct 21 2008, 09:34 PM

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 09:36 PM Publicado: #62
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Erwin and Fire @ Oct 21 2008, 11:54 PM) $TEX: \[<br />\begin{gathered}<br /> Sea\,f(x) = \sqrt {x\,} \,\,;\,\,\,x_0 = 1\,;\,\,\Delta x = \alpha \hfill \\<br /> f(x_0 + \Delta x) = \sqrt {\alpha + 1} \hfill \\<br /> f'(x) = \frac{1}<br />{{2\sqrt x }} \to f'(x_0 ) = \frac{1}<br />{2} \hfill \\<br /> \Delta y = f(x_0 + \Delta x) - f(x_0 )\, \Rightarrow \Delta y = \sqrt {\alpha + 1} - 1 \hfill \\<br /> dy = f'(x_0 )\Delta x = \frac{\alpha }<br />{2} \hfill \\<br /> \Delta y \approx dy \Rightarrow \sqrt {\alpha + 1} - 1 \approx \frac{\alpha }<br />{2} \hfill \\<br /> \therefore \sqrt {\alpha + 1} \approx \frac{\alpha }<br />{2} + 1 \hfill \\ <br />\end{gathered} <br />\]$ me falto poner esto en la segunda linea XD $TEX: \[<br />f(x_0 + \Delta x) \approx f(x_0 ) + f'(x_0 )\Delta x<br />\]$ si me equivoco, avisen Correcto!!! , ahora postea tu problema . Saludos -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

CuadraoFunk Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 09:49 PM Publicado: #63
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 165 Registrado: 15-October 06 Desde: Santiago Miembro Nº: 2.530 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Uchiha Itachi @ Oct 14 2008, 08:39 PM) $TEX: \[<br />\begin{gathered}<br /> {\text{Otra forma de hacer la derivada por definicion de }}f\left( x \right) = \sin x \hfill \\<br /> \boxed{{\text{Solucion :}}} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{\sin \left( {x + h} \right) - \sin x}}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{2\sin \left( {\frac{{x + h - x}}<br />{2}} \right)\cos \left( {\frac{{x + h + x}}<br />{2}} \right)}}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{2\sin \left( {\frac{h}<br />{2}} \right)\cos \left( {\frac{{2x + h}}<br />{2}} \right)}}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{\sin \left( {\frac{h}<br />{2}} \right)}}<br />{{\frac{h}<br />{2}}} \cdot \cos \left( {x + \frac{h}<br />{2}} \right) \hfill \\<br /> f'\left( x \right) = \left\{ {\mathop {\lim }\limits_{h \to 0} \frac{{\sin \left( {\frac{h}<br />{2}} \right)}}<br />{{\frac{h}<br />{2}}}} \right\} \cdot \left\{ {\mathop {\lim }\limits_{h \to 0} \cos \left( {x + \frac{h}<br />{2}} \right)} \right\} \hfill \\<br /> f'\left( x \right) = 1 \cdot \cos \left( x \right) = \cos \left( x \right) \hfill \\<br /> \hfill \\<br /> \therefore f'\left( x \right) = \cos x \hfill \\<br /> \hfill \\<br /> {\text{Saludos}} \hfill \\ <br />\end{gathered} <br />\]$ Siempre se aprecian nuevas formas de ver las cosas -------------------- Felipe Ramirez Ingenieria en Aviacion

Erwin and Fire Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 10:05 PM Publicado: #64
Principiante Matemático Grupo: Usuario FMAT Mensajes: 11 Registrado: 4-April 08 Miembro Nº: 18.992 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	P-18 $TEX: \[<br />\begin{gathered}<br /> Un{\text{ }}automovilista{\text{ }}es{\text{ }}\det enido{\text{ }}por{\text{ }}carabineros{\text{ }}y{\text{ }}multado{\text{ }} \hfill \\<br /> por{\text{ }}exceso{\text{ }}de{\text{ }}velocidad{\text{ }}ya{\text{ }}que{\text{ }}recorrio{\text{ }}245{\text{ }}kms{\text{ }}en \hfill \\<br /> dos{\text{ }}horas.{\text{ }}Alega{\text{ }}inocencia{\text{ }}ante{\text{ }}la{\text{ }}autoridad. \hfill \\<br /> Que{\text{ }}opina{\text{ }}ud?{\text{ }} \hfill \\ <br />\end{gathered} <br />\]$ lo edito, ya que me falto poner que recorrio los 245 km en dos horas Mensaje modificado por Erwin and Fire el Oct 22 2008, 05:53 PM

JoNy_SaTiE Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 22 2008, 06:27 PM Publicado: #65
Dios Matemático Supremo Grupo: Colaborador Gold Mensajes: 1.118 Registrado: 11-September 05 Desde: Valdivia/Ancud Miembro Nº: 302 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Erwin and Fire @ Oct 21 2008, 10:05 PM) P-18 $TEX: \[<br />\begin{gathered}<br /> Un{\text{ }}automovilista{\text{ }}es{\text{ }}\det enido{\text{ }}por{\text{ }}carabineros{\text{ }}y{\text{ }}multado{\text{ }} \hfill \\<br /> por{\text{ }}exceso{\text{ }}de{\text{ }}velocidad{\text{ }}ya{\text{ }}que{\text{ }}recorrio{\text{ }}245{\text{ }}kms{\text{ }}en \hfill \\<br /> dos{\text{ }}horas.{\text{ }}Alega{\text{ }}inocencia{\text{ }}ante{\text{ }}la{\text{ }}autoridad. \hfill \\<br /> Que{\text{ }}opina{\text{ }}ud?{\text{ }} \hfill \\ <br />\end{gathered} <br />\]$ lo edito, ya que me falto poner que recorrio los 245 km en dos horas Tengo dos preguntas. La primera, cuál sería el exceso de velocidad según Carabineros y segundo, ¿este problema se resuelve conderivadas? Saludos -------------------- Comienza a crear documentos con LaTeX. Ya usas LaTeX y quieres aprender un poco más ... pincha aquí Si eres de la UaCH ... únete a la causa !!! J. Jonathan H. Oberreuter A. Universidad Austral de Chile - RWTH Aachen alumni Est. Magister en Acústica y Vibraciones Ingeniero Civil Acústico (E) Bachiller y Licenciado en Cs. de la Ingeniería

Erwin and Fire Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 22 2008, 06:47 PM Publicado: #66
Principiante Matemático Grupo: Usuario FMAT Mensajes: 11 Registrado: 4-April 08 Miembro Nº: 18.992 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(JoNy_SaTiE @ Oct 22 2008, 07:27 PM) Tengo dos preguntas. La primera, cuál sería el exceso de velocidad según Carabineros y segundo, ¿este problema se resuelve conderivadas? Saludos estamos en chile. asi que supongo que la velocidad maxima en carretera es de 120km/h y si se resuelve con derivada, mejor dicho con un teorema que usa el concepto de derivada .

Rattlehead_ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 22 2008, 07:24 PM Publicado: #67
Dios Matemático Grupo: Usuario FMAT Mensajes: 428 Registrado: 19-July 07 Desde: Ñuñoa Miembro Nº: 7.679 Nacionalidad: Universidad: Sexo:	CITA(Erwin and Fire @ Oct 22 2008, 01:05 AM) P-18 $TEX: \[<br />\begin{gathered}<br /> Un{\text{ }}automovilista{\text{ }}es{\text{ }}\det enido{\text{ }}por{\text{ }}carabineros{\text{ }}y{\text{ }}multado{\text{ }} \hfill \\<br /> por{\text{ }}exceso{\text{ }}de{\text{ }}velocidad{\text{ }}ya{\text{ }}que{\text{ }}recorrio{\text{ }}245{\text{ }}kms{\text{ }}en \hfill \\<br /> dos{\text{ }}horas.{\text{ }}Alega{\text{ }}inocencia{\text{ }}ante{\text{ }}la{\text{ }}autoridad. \hfill \\<br /> Que{\text{ }}opina{\text{ }}ud?{\text{ }} \hfill \\ <br />\end{gathered} <br />\]$ lo edito, ya que me falto poner que recorrio los 245 km en dos horas Veamos. $TEX: <br />Podemos identificar el problema como una funcion de la distancia con el tiempo, es decir $f(t)=d$, donde $d$ representa la distancia recorrida, por enunciado $f(2)=245$ y $f(0)=0$, tomando como origen el punto en el que se encontraba hace 2 horas. Claramente la funcion es continua y derivable donde $f'$ representa la velocidad instantanea, entonces por TVM, existe un instante $c$ tal que $f(2)-f(0)=f'©(2-0) \Rightarrow f'©=122.5$. En conclusion durante su recorrido al menos un momento supero la velocidad maxima permitida, por ende es culpable$ -------------------- "Las Matematicas son el alfabeto con el cual Dios ha escrito el universo"

Erwin and Fire Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 22 2008, 07:36 PM Publicado: #68
Principiante Matemático Grupo: Usuario FMAT Mensajes: 11 Registrado: 4-April 08 Miembro Nº: 18.992 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Metal Militia @ Oct 22 2008, 08:24 PM) Veamos. $TEX: <br />Podemos identificar el problema como una funcion de la distancia con el tiempo, es decir $f(t)=d$, donde $d$ representa la distancia recorrida, por enunciado $f(2)=245$ y $f(0)=0$, tomando como origen el punto en el que se encontraba hace 2 horas. Claramente la funcion es continua y derivable donde $f'$ representa la velocidad instantanea, entonces por TVM, existe un instante $c$ tal que $f(2)-f(0)=f'©(2-0) \Rightarrow f'©=122.5$. En conclusion durante su recorrido al menos un momento supero la velocidad maxima permitida, por ende es culpable$ correcto postea tu problema

Rattlehead_ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 22 2008, 07:39 PM Publicado: #69
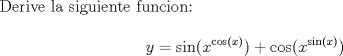Dios Matemático Grupo: Usuario FMAT Mensajes: 428 Registrado: 19-July 07 Desde: Ñuñoa Miembro Nº: 7.679 Nacionalidad: Universidad: Sexo:	$TEX: Derive la siguiente funcion:<br /><br />$$y=\sin(x^{\cos(x)})+\cos(x^{\sin(x)})$$$ -------------------- "Las Matematicas son el alfabeto con el cual Dios ha escrito el universo"

Erwin and Fire Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 22 2008, 09:38 PM Publicado: #70
Principiante Matemático Grupo: Usuario FMAT Mensajes: 11 Registrado: 4-April 08 Miembro Nº: 18.992 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Metal Militia @ Oct 22 2008, 08:39 PM) $TEX: Derive la siguiente funcion:<br /><br />$$y=\sin(x^{\cos(x)})+\cos(x^{\sin(x)})$$$ $TEX: \[<br />\begin{gathered}<br /> y = \sin (x^{\cos (x)} ) + \cos (x^{\sin (x)} ) \hfill \\<br /> y = \sin (w) + \cos (z);w = x^{\cos (x)} ;z = x^{\sin (x)} \hfill \\<br /> y' = \operatorname{si} n'(w)w' + \operatorname{co} s'(z)z' \hfill \\<br /> w = x^{\cos (x)} /\ln \Rightarrow \ln (w) = \cos (x)\ln (x)/()' \hfill \\<br /> \Rightarrow \frac{{w'}}<br />{w} = - \sin (x)\ln (x) + \frac{{\cos (x)}}<br />{x} \hfill \\<br /> \Rightarrow w' = [\frac{{\cos (x)}}<br />{x} - \sin (x)\ln (x)]x^{\cos (x)} \hfill \\<br /> z = x^{\sin (x)} /\ln \Rightarrow \ln (z) = \sin (x)\ln (x)/()' \hfill \\<br /> \Rightarrow \frac{{z'}}<br />{z} = \cos (x)\ln (x) + \frac{{\sin (x)}}<br />{x} \hfill \\<br /> \Rightarrow z' = [\cos (x)\ln (x) + \frac{{\sin (x)}}<br />{x}]x^{\sin (x)} \hfill \\<br /> Luego \hfill \\<br /> y' = \cos (x^{\cos (x)} )[\frac{{\cos (x)}}<br />{x} - \sin (x)\ln (x)]x^{\cos (x)} - \sin (x^{\sin (x)} )[\cos (x)\ln (x) + \frac{{\sin (x)}}<br />{x}]x^{\sin (x)} \hfill \\ <br />\end{gathered} <br />\]$ me equivoque en los signos al final u.u xD Mensaje modificado por Erwin and Fire el Oct 22 2008, 09:49 PM

« Posterior · Cálculo I · Anterior »

10 Páginas:

« < 5 6 7 8 9 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 09:09 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.