Derivatón Uach - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Austral de Chile > Cálculo I

10 Páginas:

« < 4 5 6 7 8 > »

Reply to this topic

Start new topic

Derivatón Uach, versión de prueba

Pedro² Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 18 2008, 08:25 PM Publicado: #51
Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Correcto, hice lo mismo que tu Uchiha =) postea tu problema Saludos. -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática* - Mechón 2009* "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 18 2008, 08:37 PM Publicado: #52
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Para practicar la regla de la cadena : $TEX: \[<br />\begin{gathered}<br /> {\text{Encuentre }}f'\left( x \right){\text{ si :}} \hfill \\<br /> f\left( x \right) = - e^{\sqrt x } \cdot \left( {\left( {x - 2\sqrt x + 1} \right)\cos \sqrt x - \left( {1 - x} \right)\sin \sqrt x } \right) \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 18 2008, 11:25 PM Publicado: #53
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	uuufff... ya se estan volando.. xD!.. me ire a dormir.. mañana la mirare.. saludos.. -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 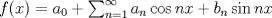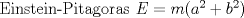$TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 19 2008, 11:57 AM Publicado: #54
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Uchiha Itachi @ Oct 18 2008, 11:37 PM) Para practicar la regla de la cadena : $TEX: \[<br />\begin{gathered}<br /> {\text{Encuentre }}f'\left( x \right){\text{ si :}} \hfill \\<br /> f\left( x \right) = - e^{\sqrt x } \cdot \left( {\left( {x - 2\sqrt x + 1} \right)\cos \sqrt x - \left( {1 - x} \right)\sin \sqrt x } \right) \hfill \\ <br />\end{gathered} <br />\]<br />$ Aqui vamos: 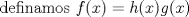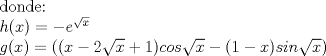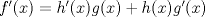$TEX: definamos $f(x)=h(x)g(x)$$ $TEX: donde:<br /><br />$h(x)= -e^{\sqrt{x}}$<br /><br />$g(x)=((x-2\sqrt{x}+1)cos\sqrt{x} -(1-x)sin \sqrt{x})$<br /><br />$ $TEX: $f'(x)=h'(x)g(x)+h(x)g'(x)$$ $TEX: Calculemos las derivadas de h y g<br /><br />$h'(x)=\frac{-e^{\sqrt{x}}}{2\sqrt{x}}$<br /><br />$g'(x)=(1-\frac{1}{\sqrt{x}})cos \sqrt{x}-\frac{(x-2\sqrt{x}+1)sin \sqrt{x}}{2\sqrt{x}}+sin \sqrt{x} - \frac{(1-x)cos \sqrt{x}}{2\sqrt{x}}$<br /><br />$g'(x)= {\frac{(x+ 2\sqrt{x} -3 )}{2\sqrt{x}}}cos \sqrt{x}-{\frac{(x-4\sqrt{x}+1)}{2\sqrt{x}}}sin \sqrt{x}$\\<br /><br />Reemplazamos en $f'(x)$<br /><br />$f'(x)=\frac{-e^{\sqrt{x}}}{2\sqrt{x}}((x-2\sqrt{x} +1)cos\sqrt{x} - (1-x)sin \sqrt{x}) - e^{\sqrt{x}}\left({\frac{(x+ 2\sqrt{x} -3 )}{2\sqrt{x}}}cos \sqrt{x}-{\frac{(x-4\sqrt{x}+1)}{2\sqrt{x}}}sin \sqrt{x}\right)$\\<br /><br /><br />factorizamos y agrupamos los terminos con seno y coseno:<br /><br />$f'(x)=-e^{\sqrt{x}}\left[ \frac{2x-2}{2\sqrt{x}}cos \sqrt{x} - \frac{2-4\sqrt{x}}{2\sqrt{x}}sin \sqrt{x} \right]$<br /><br />$f'(x)=-e^{\sqrt{x}}\left[ \frac{x-1}{\sqrt{x}}cos \sqrt{x} - \frac{1-2\sqrt{x}}{\sqrt{x}}sin \sqrt{x} \right]$\\<br /><br />finamente tenemos:<br /><br />$f'(x)=\frac{-e^{\sqrt{x}}}{\sqrt{x}}\left( (x-1)cos \sqrt{x} + (2\sqrt{x}-1)sin \sqrt{x} \right)$<br /><br />$ Espero que este bien.. Saludos.. pd: me falta un parentesis, pero no quiere aparecer.. Mensaje modificado por smaug el Oct 19 2008, 04:07 PM -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile $TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 19 2008, 07:53 PM Publicado: #55
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Correcto , propon tu problema Saludos -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 19 2008, 08:03 PM Publicado: #56
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Aqui va P-16 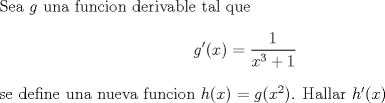$TEX: <br />Sea $g$ una funcion derivable tal que <br />$$<br />g'(x)=\displaystyle\frac{1}{x^3+1}<br />$$<br /><br />se define una nueva funcion $h(x)=g(x^2)$. Hallar $h'(x)$<br /><br />$ Saludos. Mensaje modificado por smaug el Oct 19 2008, 08:11 PM -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 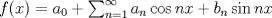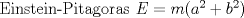$TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 19 2008, 08:10 PM Publicado: #57
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> \boxed{{\text{Solucion:}}} \hfill \\<br /> {\text{Sabiendo que : }} \hfill \\<br /> \left( {f \circ g} \right)^\prime \left( x \right) = f'\left( {g\left( x \right)} \right) \cdot g'\left( x \right) \hfill \\<br /> {\text{Entonces :}} \hfill \\<br /> {\text{Sea : }}f\left( x \right) = x^2 \Rightarrow g\left( {x^2 } \right) = g\left( {f\left( x \right)} \right) = \left( {g \circ f} \right)\left( x \right) \hfill \\<br /> {\text{Asi :}} \hfill \\<br /> h\left( x \right) = g\left( {x^2 } \right) = \left( {g \circ f} \right)\left( x \right) \hfill \\<br /> {\text{Luego :}} \hfill \\<br /> h'\left( x \right) = g'\left( {f\left( x \right)} \right) \cdot f'\left( x \right) \hfill \\<br /> h'\left( x \right) = \frac{1}<br />{{\left( {f\left( x \right)} \right)^3 + 1}} \cdot 2x \hfill \\<br /> \boxed{h'\left( x \right) = \frac{{2x}}<br />{{x^6 + 1}}} \hfill \\<br /> \hfill \\<br /> {\text{Saludos}} \hfill \\ <br />\end{gathered} <br />\]$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 19 2008, 08:12 PM Publicado: #58
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Correcto.. -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile $TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 19 2008, 08:14 PM Publicado: #59
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Ahora mi problema : $TEX: \[<br />{\text{Demuestre que : }}\sqrt {\alpha + 1} \approx \frac{\alpha }<br />{2} + 1<br />\]<br />$ Podria ser de ayuda revisar el ejercicio 2 del siguiente link : http://www.fmat.cl/index.php?showtopic=313...mp;#entry217373 -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 05:22 PM Publicado: #60
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Dale Erwin and Fire, yo se que tu tienes la respuesta,.. si no te apuras yo lo resolvere!.. xD! -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile $TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

« Posterior · Cálculo I · Anterior »

10 Páginas:

« < 4 5 6 7 8 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 10:30 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.