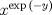|
|
|
|
|
|
|
  |
 Oct 17 2008, 03:31 PM Oct 17 2008, 03:31 PM
Publicado:
#41
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Sea 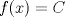 una funcion constante y una funcion constante y 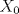 un punto cualkiera de dicha funcion un punto cualkiera de dicha funcionEntonces: 1) 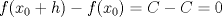 esto implica que: 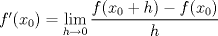 aplicando 1) aquí aplicando 1) aquí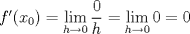 por lo que Si  ---> --->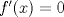 Correcto. Plantea tu problema. -------------------- Ingeniero Civil en Obras Civiles en formación
Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 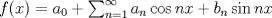  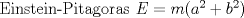 |
|
|
|
 Oct 18 2008, 01:49 PM Oct 18 2008, 01:49 PM
Publicado:
#42
|
|
 Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 28 Registrado: 14-April 08 Desde: Valdivia Miembro Nº: 20.061 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
ok! ahi va..
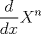 Saludos... |
|
|
|
 Oct 18 2008, 04:40 PM Oct 18 2008, 04:40 PM
Publicado:
#43
|
|
 Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[<br />\begin{gathered}<br /> \frac{d}<br />{{dx}}\left\{ {x^n } \right\} = \mathop {\lim }\limits_{h \to 0} \frac{{(x + h)^n - x^n }}<br />{h} = \mathop {\lim }\limits_{h \to 0} \frac{{e^{n\ln \left( {x + h} \right)} - e^{n\ln x} }}<br />{h} = \mathop {\lim }\limits_{h \to 0} \frac{{e^{n\ln x} \left( {e^{n\ln \left( {x + h} \right) - n\ln x} - 1} \right)}}<br />{h} \hfill \\<br /> = \mathop {\lim }\limits_{h \to 0} \frac{{e^{n\ln x} \left( {\exp \left\{ {n\ln \left( {1 + \frac{h}<br />{x}} \right)} \right\} - 1} \right)}}<br />{h} \hfill \\<br /> {\textsf{Haciendo }}z = n\ln \left( {1 + \frac{h}<br />{x}} \right) \Rightarrow h = x\left( {e^{z/n} - 1} \right) \hfill \\<br /> \frac{d}<br />{{dx}}\left\{ {x^n } \right\} = \mathop {\lim }\limits_{z \to 0} \frac{{e^{n\ln x} \left( {e^z - 1} \right)}}<br />{{x\left( {e^{z/n} - 1} \right)}} = \mathop {\lim }\limits_{z \to 0} \frac{{e^{n\ln x} }}<br />{x} \cdot \left( {\frac{{e^z - 1}}<br />{z}} \right) \cdot \left( {\frac{{z/n}}<br />{{e^{z/n} - 1}}} \right) \cdot n = \frac{{e^{n\ln x} }}<br />{x} \cdot 1 \cdot 1 \cdot n \hfill \\<br /> \Rightarrow \frac{d}<br />{{dx}}\left\{ {x^n } \right\} = nx^{n - 1} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/65aacaec916ac010ae432378ea73aae1.png)
-------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática - Mechón 2009 "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy |
|
|
|
 Oct 18 2008, 05:46 PM Oct 18 2008, 05:46 PM
Publicado:
#44
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
![TEX: \[<br />\begin{gathered}<br /> {\text{Otra forma de hacerlo :}} \hfill \\<br /> \boxed{{\text{Solucion :}}} \hfill \\<br /> \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{f\left( {x + h} \right) - f\left( x \right)}}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{\left( {x + h} \right)^n - x^n }}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{\sum\limits_{i = 0}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> i \hfill \\ <br />\end{gathered} \right)x^{n - i} h^i - x^n } }}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{\left( \begin{gathered}<br /> n \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right)x^n h^0 + \sum\limits_{i = 1}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> i \hfill \\ <br />\end{gathered} \right)x^{n - i} h^i - x^n } }}<br />{h} \hfill \\ <br />\end{gathered} <br />\]](./tex/16dc114239a78291b1620af702f45b4e.png) ![TEX: \[<br />\begin{gathered}<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{\sum\limits_{i = 1}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> i \hfill \\ <br />\end{gathered} \right)x^{n - i} h^i } }}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \frac{{h \cdot \sum\limits_{i = 1}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> i \hfill \\ <br />\end{gathered} \right)x^{n - i} h^{i - 1} } }}<br />{h} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \sum\limits_{i = 1}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> i \hfill \\ <br />\end{gathered} \right)x^{n - i} h^{i - 1} } \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \left\{ {\left( \begin{gathered}<br /> n \hfill \\<br /> 1 \hfill \\ <br />\end{gathered} \right)x^{n - 1} \cdot h^0 + \sum\limits_{i = 2}^n {\left( \begin{gathered}<br /> n \hfill \\<br /> i \hfill \\ <br />\end{gathered} \right)x^{n - i} h^{i - 1} } } \right\} \hfill \\<br /> f'\left( x \right) = \mathop {\lim }\limits_{h \to 0} \left( \begin{gathered}<br /> n \hfill \\<br /> 1 \hfill \\ <br />\end{gathered} \right)x^{n - 1} = n \cdot x^{n - 1} \hfill \\<br /> \hfill \\<br /> {\text{Saludos}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/323f00bbe6e2a74696823dd8145907d9.png) -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Oct 18 2008, 06:04 PM Oct 18 2008, 06:04 PM
Publicado:
#45
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad:  Sexo:  |
Lamentablemente tu solución no es del todo válida, pues, estás calculando el límite para cada
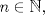 y la derivada se satisface para cualquier y la derivada se satisface para cualquier 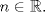 |
|
|
|
 Oct 18 2008, 06:10 PM Oct 18 2008, 06:10 PM
Publicado:
#46
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Lamentablemente tu solución no es del todo válida, pues, estás calculando el límite para cada 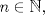 y la derivada se satisface para cualquier y la derivada se satisface para cualquier 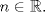 Ahora que lo veo si xD , jjajajaa -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Oct 18 2008, 06:40 PM Oct 18 2008, 06:40 PM
Publicado:
#47
|
|
 Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 28 Registrado: 14-April 08 Desde: Valdivia Miembro Nº: 20.061 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
jaja ha tenido harta participacion este tema =), creo que Pedro como respondio primero, deberia proponer su ejercicio, byes
|
|
|
|
 Oct 18 2008, 07:37 PM Oct 18 2008, 07:37 PM
Publicado:
#48
|
|
 Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Acá mi propuesto.
Hallar 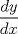 para para ![TEX: \[<br />y^{x^{\exp \left\{ { - y} \right\}} } - e^{\sin x} = 0<br />\]<br />](./tex/bae387622729c486b636b5c2d109acca.png) Salu -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática - Mechón 2009 "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy |
|
|
|
 Oct 18 2008, 08:11 PM Oct 18 2008, 08:11 PM
Publicado:
#49
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
Ahora si , espero no haberme equivocado en nada :
![TEX: \[<br />\begin{gathered}<br /> \boxed{{\text{Solucion :}}} \hfill \\<br /> {\text{La igualdad es : }}y^{x^{e^{ - y} } } - e^{\sin x} = 0 \hfill \\<br /> \Rightarrow y^{x^{e^{ - y} } } = e^{\sin x} /\ln \hfill \\<br /> \Rightarrow x^{e^{ - y} } \ln y = \sin x/\frac{{dy}}<br />{{dx}} \hfill \\<br /> \Rightarrow \left( {x^{e^{ - y} } } \right)^\prime \ln y + \left( {x^{e^{ - y} } } \right)\left( {\ln y} \right)^\prime = \left( {\sin x} \right)^\prime \hfill \\<br /> \hfill \\<br /> {\text{Sea : }} \hfill \\<br /> \varphi = x^{e^{ - y} } /\ln \Rightarrow \ln \varphi = e^{ - y} \cdot \ln x/()' \hfill \\<br /> \Rightarrow \frac{{\varphi '}}<br />{\varphi } = - y'e^{ - y} \ln x + \frac{{e^{ - y} }}<br />{x} \hfill \\<br /> \Rightarrow \varphi ' = \left( {x^{e^{ - y} } } \right) \cdot \left\{ { - y'e^{ - y} \ln x + \frac{{e^{ - y} }}<br />{x}} \right\} \hfill \\ <br />\end{gathered} <br />\]](./tex/2795d3cbd4d141f020b8bbf9fbbc051d.png) ![TEX: \[<br />\begin{gathered}<br /> {\text{Continuando con la derivacion inicial :}} \hfill \\<br /> \Rightarrow \left( {x^{e^{ - y} } } \right)\ln y \cdot \left\{ { - y'e^{ - y} \ln x + \frac{{e^{ - y} }}<br />{x}} \right\} + \frac{{\left( {x^{e^{ - y} } } \right)y'}}<br />{y} = \cos x \hfill \\<br /> \Rightarrow y'\left\{ {\frac{{x^{e^{ - y} } }}<br />{y} - x^{e^{ - y} } e^{ - y} \ln x\ln y} \right\} = \cos x - \frac{{x^{e^{ - y} } e^{ - y} \ln y}}<br />{x} \hfill \\<br /> \Rightarrow y' = \frac{{\frac{{x\cos x - x^{e^{ - y} } e^{ - y} \ln y}}<br />{x}}}<br />{{\frac{{x^{e^{ - y} } - yx^{e^{ - y} } e^{ - y} \ln x\ln y}}<br />{y}}} \hfill \\<br /> \hfill \\<br /> \therefore \frac{{dy}}<br />{{dx}} = \frac{{y\left( {x\cos x - x^{e^{ - y} } e^{ - y} \ln y} \right)}}<br />{{x\left( {x^{e^{ - y} } - yx^{e^{ - y} } e^{ - y} \ln x\ln y} \right)}} \hfill \\ <br />\end{gathered} <br />\]<br />](./tex/f4c4903f3d9516410f5e3bcf7b7645d5.png) Saludos !! -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile
Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH |
|
|
|
 Oct 18 2008, 08:16 PM Oct 18 2008, 08:16 PM
Publicado:
#50
|
|
 Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad:  Colegio/Liceo:  Universidad:  Sexo:  |
me quedo una ensalada.. xD!
se puede despejar y'? -------------------- Ingeniero Civil en Obras Civiles en formación
Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile    |
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 18th October 2025 - 09:32 AM |









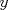 es
es