Derivatón Uach - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Austral de Chile > Cálculo I

10 Páginas:

« < 2 3 4 5 6 > »

Reply to this topic

Start new topic

Derivatón Uach, versión de prueba

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 14 2008, 09:19 PM Publicado: #31
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA P9 (planteado por Uchiha Itachi) Demostrar que 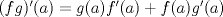$TEX: $(fg)'(a)= g(a)f'(a)+f(a)g'(a)$$ Hola, aqui va.. espero este bien $TEX: Usare esta definición de derivada<br /> <br /> $f'(a)= \displaystyle\lim_{x \to a}{\frac{f(x)-f(a)}{x-a}}$<br /> <br />entonces: <br /><br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{fg(x)-fg(a)}{x-a}}$<br /> <br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{f(x)g(x)-f(a)g(a)}{x-a}}$<br /> <br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{f(x)g(x)-f(a)g(a)+f(a)g(x)-f(a)g(x)}{x-a}}$<br /> <br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{g(x)(f(x)-f(a))}{x-a}} + \displaystyle\lim_{x \to a}{\frac{f(a)(g(x)-g(a))}{x-a}}$<br /> <br />Aplicando el limite <br /> <br /> $fg'(a)= g(a)f'(a)+f(a)g'(a)$<br />$ Saludos -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 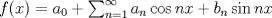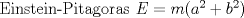$TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 14 2008, 10:48 PM Publicado: #32
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Planteare mi problema, pido disculpas por no esperar la respuesta del moderador o de quien planteo el problema, pero creo que esta bien y si espero alguien mas planteara otro. 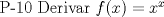$TEX: <br />P-10 Derivar $f(x)=x^x$$ (recordar derivada implicita) Saludos. -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 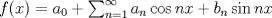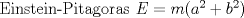$TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

alboadikto Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 15 2008, 12:54 AM Publicado: #33
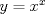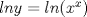Dios Matemático Grupo: Usuario FMAT Mensajes: 213 Registrado: 17-July 07 Desde: El Barrio de Arellano Miembro Nº: 7.631 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Sea $TEX: $y=x^x$$ , aplicando ln $TEX: $lny=ln(x^x)$$ $TEX: \[<br />\begin{gathered}<br /> \left( {\ln y} \right)' = \left( {\ln x^x } \right)' \hfill \\<br /> \frac{1}<br />{y} \cdot y' = \left( {x\ln x} \right)' \hfill \\<br /> \hfill \\<br /> \frac{1}<br />{y} \cdot y' = x'\ln x + x(\ln x)' \hfill \\<br /> \hfill \\<br /> \frac{1}<br />{y} \cdot y' = \ln x + 1 \hfill \\<br /> y' = y(\ln x + 1) \hfill \\<br /> y' = x^x (\ln x + 1) \hfill \\ <br />\end{gathered} <br />\]<br />$ Demás que tengo algún error de tipeo, ya que hace harto que no usaba mathtype y me tiro muchos errores, pero espero que este bien.

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 15 2008, 07:27 AM Publicado: #34
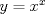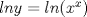Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(P e L a O @ Oct 15 2008, 03:44 AM) Sea $TEX: $y=x^x$$ , aplicando ln $TEX: $lny=ln(x^x)$$ $TEX: \[<br />\begin{gathered}<br /> \left( {\ln y} \right)' = \left( {\ln x^x } \right)' \hfill \\<br /> \frac{1}<br />{y} \cdot y' = \left( {x\ln x} \right)' \hfill \\<br /> \hfill \\<br /> \frac{1}<br />{y} \cdot y' = x'\ln x + x(\ln x)' \hfill \\<br /> \hfill \\<br /> \frac{1}<br />{y} \cdot y' = \ln x + 1 \hfill \\<br /> y' = y(\ln x + 1) \hfill \\<br /> y' = x^x (\ln x + 1) \hfill \\ <br />\end{gathered} <br />\]<br />$ Demás que tengo algún error de tipeo, ya que hace harto que no usaba mathtype y me tiro muchos errores, pero espero que este bien. Correcto.. Plantea tu problema. ( no olvides numerarlo) -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile $TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 15 2008, 09:49 AM Publicado: #35
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(smaug @ Oct 15 2008, 02:09 AM) Hola, aqui va.. espero este bien $TEX: Usare esta definición de derivada<br /> <br /> $f'(a)= \displaystyle\lim_{x \to a}{\frac{f(x)-f(a)}{x-a}}$<br /> <br />entonces: <br /><br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{fg(x)-fg(a)}{x-a}}$<br /> <br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{f(x)g(x)-f(a)g(a)}{x-a}}$<br /> <br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{f(x)g(x)-f(a)g(a)+f(a)g(x)-f(a)g(x)}{x-a}}$<br /> <br /> $fg'(a)= \displaystyle\lim_{x \to a}{\frac{g(x)(f(x)-f(a))}{x-a}} + \displaystyle\lim_{x \to a}{\frac{f(a)(g(x)-g(a))}{x-a}}$<br /> <br />Aplicando el limite <br /> <br /> $fg'(a)= g(a)f'(a)+f(a)g'(a)$<br />$ Saludos Correcto!!! ( Perdon por la demora , pero estaba ocupado ) Saludines -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 15 2008, 05:52 PM Publicado: #36
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> {\text{Perdon si no me toca ( nuevamente ) }}{\text{, pero para que continue :}} \hfill \\<br /> \hfill \\<br /> {\text{Demuestre que si }}f{\text{ es derivable en }}x_0 {\text{ }}{\text{, entonces es continua en }}x_0 \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 16 2008, 12:40 PM Publicado: #37
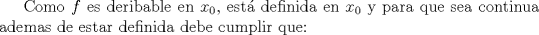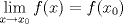Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	No estoy muy seguro de esto, pero se me ocurre que puede ser asi: 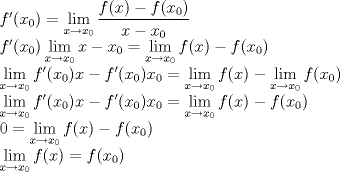$TEX: Como $f$ es derivable en $x_0$, está definida en $x_0$ y para que sea continua ademas de estar definida debe cumplir que:<br />$ $TEX: <br /><br />$\displaystyle\lim_{x \to x_0}{f(x)}=f(x_0)$<br /><br />$ $TEX: Demostración: si $f$ es deribable este limite existe:$ $TEX: <br />$f'(x_0)= \displaystyle\lim_{x \to x_0}{\frac{f(x)-f(x_0)}{x-x_0}}$<br /><br />$f'(x_0)\displaystyle\lim_{x \to x_0}{x-x_0}= \displaystyle\lim_{x \to x_0}{f(x)-f(x_0)}$<br /><br />$\displaystyle\lim_{x \to x_0}{f'(x_0)x-f'(x_0)x_0}= \displaystyle\lim_{x \to x_0}{f(x)}-\displaystyle\lim_{x \to x_0}{f(x_0)}$<br /><br />$\displaystyle\lim_{x \to x_0}{f'(x_0)x-f'(x_0)x_0}= \displaystyle\lim_{x \to x_0}{f(x)}-{f(x_0)}$<br /><br />$0=\displaystyle\lim_{x \to x_0}{f(x)}-{f(x_0)}$<br /><br />$\displaystyle\lim_{x \to x_0}{f(x)}=f(x_0)$<br /><br />$ Saludos Mensaje modificado por smaug el Oct 17 2008, 01:12 PM -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 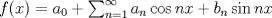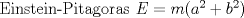$TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 16 2008, 06:58 PM Publicado: #38
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Correcto smaug , yo igual tengo otra forma la expondre por siacaso : $TEX: \[<br />\begin{gathered}<br /> \boxed{{\text{Demostracion :}}} \hfill \\<br /> {\text{Si }}f{\text{ es derivable en }}x_0 {\text{ }}{\text{, entonces :}} \hfill \\<br /> f'\left( {x_0 } \right) = \mathop {\lim }\limits_{h \to 0} \frac{{f\left( {x_0 + h} \right) - f\left( {x_0 } \right)}}<br />{h} = L \hfill \\<br /> {\text{Y debemos demostrar que es continua en ese punto }}{\text{, osea :}} \hfill \\<br /> \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = f\left( {x_0 } \right) \hfill \\<br /> {\text{Entonces haciendo el siguiente cambio de variables:}} \hfill \\<br /> h = x - x_0 \Rightarrow x \to x_0 {\text{ }}{\text{, }}h \to 0 \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = \mathop {\lim }\limits_{h \to 0} f\left( {h + x_0 } \right) \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = \mathop {\lim }\limits_{h \to 0} \left\{ {\frac{{f\left( {h + x_0 } \right) + f\left( {x_0 } \right) - f\left( {x_0 } \right)}}<br />{h}} \right\} \cdot h \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = \mathop {\lim }\limits_{h \to 0} \left\{ {\frac{{f\left( {x_0 + h} \right) - f\left( {x_0 } \right)}}<br />{h}} \right\} \cdot h + \mathop {\lim }\limits_{h \to 0} \left\{ {\frac{{f\left( {x_0 } \right)}}<br />{h} \cdot h} \right\} \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = \left\{ {\mathop {\lim }\limits_{h \to 0} \frac{{f\left( {x_0 + h} \right) - f\left( {x_0 } \right)}}<br />{h}} \right\} \cdot \mathop {\lim }\limits_{h \to 0} h + \mathop {\lim }\limits_{h \to 0} f\left( {x_0 } \right) \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = f'\left( {x_0 } \right) \cdot 0 + f\left( {x_0 } \right) \hfill \\<br /> \Rightarrow \mathop {\lim }\limits_{x \to x_0 } f\left( x \right) = f\left( {x_0 } \right) \hfill \\<br /> \hfill \\<br /> {\text{Q}}{\text{.e}}{\text{.d}} \hfill \\ <br />\end{gathered} <br />\]$ Postea tu problema saludos -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

smaug Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 17 2008, 01:27 PM Publicado: #39
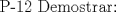Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 145 Registrado: 28-November 07 Desde: valdivia-chiloe Miembro Nº: 13.217 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Uno facil 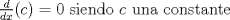$TEX: P-12 Demostrar:$ $TEX: $\frac{d}{dx}©=0$ siendo $c$ una constante$ Saludos.. -------------------- Ingeniero Civil en Obras Civiles en formación Bachiller en Ciencias de la Ingeniería Universidad Austral de Chile 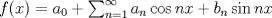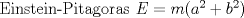$TEX: $f(x)=a_0 + \sum^{\infty}_{n=1} a_{n}\cos {nx} + b_{n}\sin {nx}$$ $TEX: <br />Einstein-Pitagoras<br />$E=m(a^2+b^2)$<br />$

Nicte Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 17 2008, 02:23 PM Publicado: #40
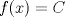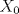Principiante Matemático Destacado Grupo: Usuario FMAT Mensajes: 28 Registrado: 14-April 08 Desde: Valdivia Miembro Nº: 20.061 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Sea $TEX: $f(x)=C$$ una funcion constante y $TEX: $x_0$$ un punto cualkiera de dicha funcion Entonces: 1) 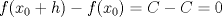$TEX: $f(x_0+h)-f(x_0)=C-C=0$$ esto implica que: 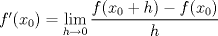$TEX: $f'(x_0)=\displaystyle \lim_{h\rightarrow 0}\displaystyle \frac{f(x_0+h)-f(x_0)}{h}$$ aplicando 1) aquí $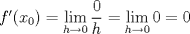TEX: $f'(x_0)=\displaystyle \lim_{h\rightarrow 0}\displaystyle \frac{0}{h}=\displaystyle \lim_{h\rightarrow 0}0=0$$ por lo que Si 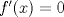$TEX: $f(x)=C$$ ---> $TEX: $f'(x)=0$$ Mensaje modificado por Nicte el Oct 17 2008, 02:30 PM

« Posterior · Cálculo I · Anterior »

10 Páginas:

« < 2 3 4 5 6 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 4th April 2025 - 02:54 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.