Derivatón Uach - Foro fmat.cl

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad Austral de Chile > Cálculo I

10 Páginas:

< 1 2 3 4 > »

Derivatón Uach, versión de prueba

Opciones

kbzoon Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 05:32 PM Publicado: #11
Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 1.627 Registrado: 11-October 07 Desde: Suburbios de Conchalí Miembro Nº: 11.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	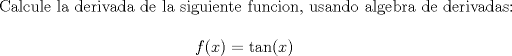CITA(Metal Militia @ Sep 28 2008, 06:08 PM) $TEX: <br />Calcule la derivada de la siguiente funcion, usando algebra de derivadas:<br /><br />$$f(x)=\tan(x)$$$ para que siga el show, vamos con esta sabiendo que $TEX: \[<br />\left( {\frac{f}<br />{g}} \right)^\prime = \frac{{f'g - fg'}}<br />{{g^2 }}<br />\]<br />$ entonces, $TEX: \[<br />f\left( x \right) = \tan \left( x \right) = \frac{{sen\left( x \right)}}<br />{{\cos \left( x \right)}}<br />\]<br />$ luego la derivada es $TEX: \[<br />f'\left( x \right) = \left( {\frac{{sen\left( x \right)}}<br />{{\cos \left( x \right)}}} \right)^\prime = \frac{{sen'\left( x \right)\cos \left( x \right) - \cos \left( x \right)^\prime sen\left( x \right)}}<br />{{\cos ^2 \left( x \right)}} = \frac{{\cos ^2 \left( x \right) + sen^2 \left( x \right)}}<br />{{\cos ^2 \left( x \right)}} = \sec ^2 \left( x \right)<br />\]<br />$ jaja arreglado el error tipeo....suelen pasar estas cosas Mensaje modificado por kbzoon el Sep 28 2008, 06:21 PM -------------------- Injeniería de Minas. The best SpaceDream Radio ever

kbzoon Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 05:38 PM Publicado: #12
Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 1.627 Registrado: 11-October 07 Desde: Suburbios de Conchalí Miembro Nº: 11.213 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	ahora, mi propuesto. P4.- calcular $TEX: \[<br />f'\left( x \right)<br />\]<br />$ de $TEX: \[<br />f\left( x \right) = \tan \left( x \right)\cos \left( x \right) - \arctan \left( {sen\left( x \right)} \right)<br />\]<br />$ ojalá se animen más -------------------- Injeniería de Minas. The best SpaceDream Radio ever

deixo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 06:04 PM Publicado: #13
Dios Matemático Supremo Grupo: Baneado Mensajes: 1.737 Registrado: 6-September 07 Miembro Nº: 9.900	CITA(Metal Militia @ Sep 28 2008, 06:08 PM) Ya , uno facil y didactico para comenzar: $TEX: <br />Calcule la derivada de la siguiente funcion, usando algebra de derivadas:<br /><br />$$f(x)=\tan(x)$$$ ya.... te tiraste al chancho

Pedro² Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 07:54 PM Publicado: #14
Dios Matemático Supremo Grupo: Colaborador Platinum Mensajes: 824 Registrado: 15-October 07 Desde: Valparaíso Miembro Nº: 11.342 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: <br />\[<br />\begin{gathered}<br /> f(x) = \tan x \hfill \\<br /> {\textsf{Por definicion:}} \hfill \\<br /> f'(x) = \mathop {\lim }\limits_{h \to 0} \frac{{\tan (x + h) - \tan x}}<br />{h} \hfill \\<br /> = \mathop {\lim }\limits_{h \to 0} \frac{{\frac{{\tan x + \tan h}}<br />{{1 - \tan x\tan h}} - \tan x}}<br />{h} = \mathop {\lim }\limits_{h \to 0} \frac{{\tan h\left( {1 + \tan ^2 x} \right)}}<br />{{h\left( {1 - \tan x\tan h} \right)}} \hfill \\<br /> {\textsf{Dado que }}\mathop {\lim }\limits_{h \to 0} \frac{{\tan h}}<br />{h} = \mathop {\lim }\limits_{h \to 0} \frac{{\sin h}}<br />{{h \cdot \cos h}} = 1\;{\text{y que }}\sec ^2 x = 1 + \tan ^2 x \hfill \\<br /> f'(x) = \sec ^2 x \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- Pedro P. Montero Silva Estudiante de Licenciatura en Ciencias, Mención Matemática* - Mechón 2009* "One rather curious conclusion emerges, that pure mathematics is on the whole distinctly more useful than applied. A pure mathematician seems to have the advantage on the practical as well as on the aesthetic side. For what is useful above all is technique, and mathematical technique is taught mainly through pure mathematics." G.H. Hardy

dalomismo... Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 08:05 PM Publicado: #15
Maestro Matemático Grupo: Usuario FMAT Mensajes: 128 Registrado: 18-March 08 Desde: Valdivia y varias partes que sinceramente no te debería importar XD Miembro Nº: 17.202 Nacionalidad: Universidad: Sexo:	que dice el jurado???????? -------------------- Estudiante de Ingeniería Civil Acústica Universidad Austral de Chile CITA “Las matemáticas son la música de la razón” James Joseph Silvestre (1814-1897). Matemático británico. “El álgebra es generosa: a menudo da más de lo que se le pide” D’Alembert (1717-1783). Matemático y filósofo francés. “La música es la aritmética de los sonidos, como la óptica es la geometría de la luz.” Claude Debussy (1862-1918) Compositor Francés “La música es un ejercicio aritmético ocultado del alma, que no sabe que está contando.” “La música es el placer que experimenta la mente humana al contar sin darse cuenta de que está contando.” Gottfried Wilhelm Leibniz(1646-1716). Filósofo y Matemático alemán. Un país que no investiga es un país que no progresa MySpace de Azotth MySpace de Denun

SanTix Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 08:14 PM Publicado: #16
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 800 Registrado: 13-December 07 Miembro Nº: 13.852 Nacionalidad: Sexo:	CITA(kbzoon @ Sep 28 2008, 05:28 PM) ahora, mi propuesto. P4.- calcular $TEX: \[<br />f'\left( x \right)<br />\]<br />$ de $TEX: \[<br />f\left( x \right) = \tan \left( x \right)\cos \left( x \right) - \arctan \left( {sen\left( x \right)} \right)<br />\]<br />$ ojalá se animen más $TEX: \[<br />\begin{gathered}<br /> f\left( x \right) = \tan x \cdot \cos x - \arctan \left( {\operatorname{sen} x} \right) = \operatorname{sen} x - \arctan \left( {\operatorname{sen} x} \right) \hfill \\<br /> \hfill \\<br /> {\text{como }}y = \arctan u \Rightarrow y' = \frac{{u'}}<br />{{u^2 + 1}} \hfill \\<br /> \hfill \\<br /> {\text{entonces }}f'\left( x \right) = \cos x - \frac{{\cos x}}<br />{{\operatorname{sen} ^2 x + 1}} = \frac{{\cos x\operatorname{sen} ^2 x}}<br />{{\operatorname{sen} ^2 x + 1}} \hfill \\ <br />\end{gathered} <br />\]$ Mensaje modificado por SanTix el Sep 28 2008, 08:14 PM

SanTix Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 08:40 PM Publicado: #17
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 800 Registrado: 13-December 07 Miembro Nº: 13.852 Nacionalidad: Sexo:	acá va la mía $TEX: \[<br />\boxed{P_5 }{\text{ Sea }}f\left( x \right) = x\left\| x \right\|,{\text{ encuentre }}f'\left( x \right).<br />\]<br />$

Ivan20i Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 08:45 PM Publicado: #18
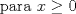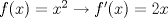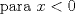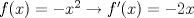
Maestro Matemático Grupo: Usuario FMAT Mensajes: 85 Registrado: 11-September 07 Desde: Consedcion Miembro Nº: 10.092 Nacionalidad: Universidad: Sexo:	$TEX: para $x \geq 0$$ $TEX: $f(x)=x^2 \to f'(x)=2x$$ $TEX: para $x<0$$ $TEX: $f(x)=-x^2 \to f'(x)=-2x$$ -------------------- $TEX: $$ L_{LL}=\sum_{p=0}^{[d/2]} \alpha_p \varepsilon_{a_1 \cdots a_d} R^{a_1 a_2} \ldots R^{a_{2p-1}a_p}e^{a_{2p+1}}\ldots e^{a_d}$$$

SanTix Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 08:57 PM Publicado: #19
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 800 Registrado: 13-December 07 Miembro Nº: 13.852 Nacionalidad: Sexo:	CITA(Ivan20i @ Sep 28 2008, 08:35 PM) $TEX: para $x \geq 0$$ $TEX: $f(x)=x^2 \to f'(x)=2x$$ $TEX: para $x<0$$ $TEX: $f(x)=-x^2 \to f'(x)=-2x$$ correcto

Ivan20i Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 28 2008, 09:00 PM Publicado: #20
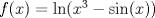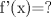
Maestro Matemático Grupo: Usuario FMAT Mensajes: 85 Registrado: 11-September 07 Desde: Consedcion Miembro Nº: 10.092 Nacionalidad: Universidad: Sexo:	$TEX: $f(x)=\ln (x^3 - \sin(x) )$$ $TEX: f'(x)=?$ -------------------- $TEX: $$ L_{LL}=\sum_{p=0}^{[d/2]} \alpha_p \varepsilon_{a_1 \cdots a_d} R^{a_1 a_2} \ldots R^{a_{2p-1}a_p}e^{a_{2p+1}}\ldots e^{a_d}$$$

« Posterior · Cálculo I · Anterior »

10 Páginas:

< 1 2 3 4 > »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 25th April 2025 - 11:55 PM