Certamen 1 E.D. II 2008 - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Concepción > Ecuaciones Diferenciales Parciales I, Complemento de Cálculo

Reply to this topic

Start new topic

Certamen 1 E.D. II 2008, 26.09.2008

intégramelo Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 26 2008, 11:59 AM Publicado: #1
Principiante Matemático Grupo: Usuario FMAT Mensajes: 6 Registrado: 11-September 08 Miembro Nº: 34.399	$TEX: \noindent<br /><br />\begin{center}<br /><br />\textbf{EVALUACION 1}\\ECUACIONES DIFERENCIALES II (525222)<br /><br />\end{center}<br />\noindent<br />\textbf{P1} Considere el Problema de Valores de Contorno<br /><br />$$ y'' - y = f, \, 0<x<1 $$<br />$$ y(0)-y'(0)=\sqrt{2} $$<br />$$ y(1) - y'(1)=\sqrt{3} $$<br /><br />\begin{enumerate}<br /><br />\item Determine si existe funci\'on de Green cl\'asica o generalizada y las funciones $f$ admisibles para el problema.<br />\item Construya la representaci\'on de Green de la soluci\'on si es $f$ admisible.<br />\item Resolver el problema de contorno si $f(x)=(e-1)x-1$<br /><br />\end{enumerate}<br />\hfill \textbf{[2.5 ptos.]}<br />\\ \vspace{8pt}<br /><br /> \noindent<br />\textbf{P2} Determinar los valores y funciones propias asociadas al problema de Sturm-Liouville.<br />$$ y''+2y'+(\lambda +1)y=0 \quad 0<x<1$$<br />$$ y'(0)=y(1)=0 $$<br /><br />Adem\'as, definir la Serie de Fourier Generalizada de una funci\'on real $f$ que verifica<br /><br />$$ \int_{0}^{2}e^{2x}f^2(x)dx<\infty $$<br />Nota: Debe presentar todos sus c\'alculos.<br /><br />\hfill \textbf{[2 ptos.]}<br /><br />$ Este es el certamen 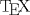$TEX: \TeX$ tual . Los 1.5 ptos. restantes corresponden a una tarea. Enjoy it!!!

mapaxe Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 27 2008, 03:50 PM Publicado: #2
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 181 Registrado: 15-September 07 Desde: mi propia Soul Society Miembro Nº: 10.218 Nacionalidad: Universidad: Sexo:	Certamen de la ctm!!!!!!!!!!!!!!!!!!! me tuvo bajoneado 5 horas mas encima que ya se hacer los ejercicios (aprendi despues del certamen xD) ya aqui va la solucion de la 2a) 2a) $TEX: <br />\[<br />\begin{gathered}<br /> y'' + 2y' + (\lambda + 1)y = 0 \hfill \\<br /> y'(0) = y(1) = 0 \hfill \\<br /> sol: \hfill \\<br /> Ly = - \lambda y \hfill \\<br /> By = 0 \hfill \\ <br />\end{gathered} <br />\]<br /><br />$ 1) de la ecuacion diferencial: $TEX: \[<br />\begin{gathered}<br /> y'' + 2y' + (\lambda + 1)y = (D^2 + 2D + \lambda + 1)y = 0 \hfill \\<br /> \Leftrightarrow (D + 1)^2 + \lambda y = 0;(D + 1) = e^{ - x} De^x \hfill \\<br /> \Leftrightarrow e^{ - x} De^x e^{ - x} De^x y + \lambda y = 0 \hfill \\<br /> \Leftrightarrow D^2 e^x y + \lambda e^x y = 0/c.v:z(x) = e^x y(x).......multiplicar...e^x \hfill \\<br /> \Leftrightarrow z'' + \lambda z = 0 \hfill \\ <br />\end{gathered} <br />\]$ 2) de las condiciones de borde: $TEX: \[<br />\begin{gathered}<br /> y(x) = e^{ - x} z(x) \Rightarrow y'(x) = - e^{ - x} z(x) + e^{ - x} z'(x) \hfill \\<br /> y'(0) = z(0) + z'(0) = 0 \hfill \\<br /> y(1) = e^{ - 1} z(1) = 0 \Rightarrow y(1) = z(1) = 0 \hfill \\ <br />\end{gathered} <br />\]<br />$ asi el problema Ly queda como uno Lz: $TEX: \[<br />\begin{gathered}<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> Lz = - \lambda z \hfill \\<br /> By = 0 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> \Leftrightarrow z'' + \lambda z = 0 \hfill \\<br /> z(0) + z'(0) = 0 \hfill \\<br /> z(1) = 0 \hfill \\ <br />\end{gathered} <br />\]<br />$ Debemos hallar los valores propios "lamda" para generar el las funciones propias, a lo largo de $TEX: \[<br />\mathbb{R}<br />\]$ sea una particion de $TEX: \[<br />\mathbb{R}<br />\]$ $TEX: \[<br />\mathbb{R} = \left] { - \infty ,0} \right[ \cup \left\{ {\left. 0 \right\}} \right. \cup \left] {0, + \infty } \right[<br />\]$ Caso 1) $TEX: \[<br />\lambda \in \mathbb{R}^ + /\lambda = w^2 ,w > 0<br />\]<br />$ $TEX: \[<br />z'' + w^2 z = 0 \Rightarrow z(x) = A\cos (wx) + B\sin (wx)<br />\]$ asi entonces: $TEX: \[<br />z'(x) = - wA\sin (wx) + wB\cos (wx)<br />\]$ de las Condiciones de contorno: $TEX: \[<br />\begin{gathered}<br /> z(0) + z'(0) = A + wB = 0 \hfill \\<br /> z(1) = A\cos (w) + B\sin (w) \hfill \\ <br />\end{gathered} <br />\]$ formamos el sgte sistema para el Problema de sturm-Liouville: $TEX: \[<br />\left( {\begin{array}{{20}c}<br /> 1 & w \\<br /> {\cos w} & {\sin w} \\<br /><br /> \end{array} } \right)\left( \begin{gathered}<br /> A \hfill \\<br /> B \hfill \\ <br />\end{gathered} \right) = \left( \begin{gathered}<br /> 0 \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right)<br />\]$ de la matriz de coeficientes tomamos el determinante: $TEX: \[<br />\begin{gathered}<br /> \det \left( {\begin{array}{{20}c}<br /> 1 & w \\<br /> {\cos w} & {\sin w} \\<br /><br /> \end{array} } \right) \ne 0 \hfill \\<br /> \Leftrightarrow \sin w - w\cos w \ne 0 \hfill \\<br /> \Leftrightarrow \tan w \ne w;w > 0 \Rightarrow Haciendo:w = 0 + n,n \in \mathbb{N} \hfill \\ <br />\end{gathered} <br />\]<br />$ podemos concluir que los valores propios en $TEX: \[<br />\mathbb{R}^ + <br />\]$ son: $TEX: \[<br />\lambda _n = n^2 <br />\]$ cuyas funciones propias son las generadas por : $TEX: \[<br />S_\lambda = \langle \left\{ {\left. {\cos (nx),\sin (nx)} \right\}} \right.\rangle <br />\]$ Caso 2: $TEX: \[<br />\lambda = 0<br />\]$ $TEX: \[<br />\begin{gathered}<br /> z'' = 0 \Rightarrow z(x) = A + Bx \hfill \\<br /> asi:z(0) + z'(0) = A + B = 0 \Rightarrow A = - B \hfill \\<br /> asa:z(1) = A + B = 0 \hfill \\<br /> \therefore \lambda = 0 \hfill \\ <br />\end{gathered} <br />\]$ no valor propio puesto que genera las funciones propias nulas (las cuales no existen) Caso 3: $TEX: \[<br />\lambda \in \mathbb{R}^ - /\lambda = - w^2 ,w > 0<br />\]<br /><br /><br />$ $TEX: \[<br />\begin{gathered}<br /> z'' - w^2 z = 0 \Rightarrow z(x) = A\cosh (wx) + B\sinh (wx) \to z'(x) = A\sinh (wx) + B\cosh (wx) \hfill \\<br /> asi:z(0) + z'(0) = 0 \hfill \\<br /> \Leftrightarrow A + B = 0 \hfill \\<br /> asa:z(1) = 0 \hfill \\<br /> \Leftrightarrow A\cosh (w) + B\sinh (w) = 0 \hfill \\ <br />\end{gathered} <br />\]<br /><br />$ creando el sistema lineal: $TEX: \[<br />\begin{gathered}<br /> \left( {\begin{array}{{20}c}<br /> 1 & 1 \\<br /> {\cosh w} & {\sinh w} \\<br /><br /> \end{array} } \right)\left( \begin{gathered}<br /> A \hfill \\<br /> B \hfill \\ <br />\end{gathered} \right) = \left( \begin{gathered}<br /> 0 \hfill \\<br /> 0 \hfill \\ <br />\end{gathered} \right) \hfill \\<br /> \Rightarrow \det \left( {\begin{array}{{20}c}<br /> 1 & 1 \\<br /> {\cosh w} & {\sinh w} \\<br /><br /> \end{array} } \right) = \sinh w - \cosh w \ne 0 \hfill \\<br /> \Leftrightarrow \frac{1}<br />{2}\left( {e^w - e^{ - w} - e^w - e^{ - w} } \right) \ne 0 \hfill \\<br /> \Leftrightarrow e^{ - w} \ne 0 \hfill \\ <br />\end{gathered} <br />\]$ lo cual es cierto siempre, lo cual tomaria a "lambda " como valor propio positivo distinto de cero pero . $TEX: \[<br /> \to \leftarrow <br />\]$ puesto que por hipotesis W es menor que 0 $TEX: \[<br />\therefore \lambda = - w^2 <br />\]$ no es un valor propio. en conclusion los valores propios al problema $TEX: \[<br />\begin{gathered}<br /> z'' + \lambda z = 0 \hfill \\<br /> z(0) + z'(0) = 0 \hfill \\<br /> z(1) = 0 \hfill \\ <br />\end{gathered} <br />\]<br /><br /><br /><br /><br /><br /><br /><br /><br />$ $TEX: \[<br />\lambda _n = n^2 <br />\]<br />$ para las funciones propias dadas: $TEX: \[<br />\begin{gathered}<br /> y(x) = e^{ - x} z(x) \hfill \\<br /> S_\lambda = \langle \left\{ {\left. {\cos (nx),\sin (nx)} \right\}} \right.\rangle \hfill \\ <br />\end{gathered} <br />\]<br />$ post: si ta mala esa * a la ** nomas xD. Mensaje modificado por mapaxe el Sep 27 2008, 05:10 PM -------------------- ] ex-estudiante Kingston college-concepcion ex-estudiante Cs fisicas y astronomicas- Udec Estudiante Ingenieria en construccion Excalibur Fan-club This is madness!!! Madness???? This is Spartaaaaaaaaaaaaaaaaaaa TE VOY A ROMPEEEEER BIEN EL ORTOOOO ACM1PT**

mapaxe Ver Perfil del Miembro Ver los mensajes de este miembro	Sep 27 2008, 05:23 PM Publicado: #3
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 181 Registrado: 15-September 07 Desde: mi propia Soul Society Miembro Nº: 10.218 Nacionalidad: Universidad: Sexo:	post: buen aporte señor espinoza , una lastima que para nosotros fue 0 aporte ayer jajajaa xD -------------------- ] ex-estudiante Kingston college-concepcion ex-estudiante Cs fisicas y astronomicas- Udec Estudiante Ingenieria en construccion Excalibur Fan-club This is madness!!! Madness???? This is Spartaaaaaaaaaaaaaaaaaaa TE VOY A ROMPEEEEER BIEN EL ORTOOOO ACM1PT

StebanoZ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2010, 01:04 AM Publicado: #4
Principiante Matemático Grupo: Usuario FMAT Mensajes: 5 Registrado: 17-March 09 Desde: la coruña. ..Hualpen. Miembro Nº: 45.131 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	si el profesor fue ruiz... no me extranaria... pero si me urgira... y caleta... gracias por el certamen.....

dariourib Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2010, 08:04 AM Publicado: #5
Maestro Matemático Grupo: Usuario FMAT Mensajes: 124 Registrado: 30-April 09 Miembro Nº: 49.927 Nacionalidad: Universidad: Sexo:	CITA(StebanoZ @ Oct 21 2010, 02:04 AM) si el profesor fue ruiz... no me extranaria... pero si me urgira... y caleta... gracias por el certamen..... Ruiz hace complemeanto de cálculo nomás... el profe de este certamen es Paiva, y es un curso de EDP a otro nivel xDD -------------------- Darío Uribe Rodríguez Estudiante de Sexto Año de Ingeniería Civil Industrial Universidad de Concepción Clases particulares para alumnos de Enseñanza Media y Superior dentro del Gran Concepción.

« Posterior · Ecuaciones Diferenciales Parciales I, Complemento de Cálculo · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 06:28 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.