Prueba Global de Bachi mate I - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Chile > Matemáticas 1

2 Páginas:

1 2 >

Reply to this topic

Start new topic

Prueba Global de Bachi mate I, :o

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 23 2008, 07:51 PM Publicado: #1
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> \boxed{{\text{Prueba Global de Mate I}}} \hfill \\<br /> \hfill \\<br /> {\text{Resuelva solo un problema entre 1 y 2}} \hfill \\<br /> \hfill \\<br /> 1.{\text{ Se requiere un potrero de forma rectangular}}{\text{, donde uno de los lados es el borde}} \hfill \\<br /> {\text{de un rio}}{\text{, para que los animales beban agua}}{\text{. Se dispone de 10}}{\text{.000 metros de alambre}} \hfill \\<br /> {\text{y el cerco debe ser de tres corridas de alambre}}{\text{. Determine cuales son las dimensiones}} \hfill \\<br /> {\text{del potrero de mayor area que se puede cercar el con alambre}} \hfill \\<br /> \hfill \\<br /> {\text{2}}{\text{. Determine cual punto de la curva }}y = \frac{1}<br />{x}{\text{ en el primer cuadrante esta mas cerca del }} \hfill \\<br /> {\text{origen}} \hfill \\<br /> \left( {{\text{Ayuda: la formula de la distancia entre 2 puntos}}} \right) \hfill \\<br /> \hfill \\<br /> {\text{Resuelva solo un problema entre 3 y 4}} \hfill \\<br /> \hfill \\<br /> {\text{3}}{\text{. Sean }}f,g:\mathbb{R} \to \mathbb{R}{\text{ diferenciables tales que }}f(0) = g(0){\text{ y que }}f'(x) \geqslant g'(x)\forall x \in \mathbb{R}. \hfill \\<br /> {\text{Demuestra que }}f(x) \geqslant g(x)\forall x>0. \hfill \\<br /> \hfill \\<br /> 4.{\text{ Existe }}x \in \mathbb{R}{\text{ tal que }}\cos \left( {\frac{{\pi x}}<br />{2}} \right) = x^3 ? \hfill \\<br /> \hfill \\<br /> \hfill \\ <br />\end{gathered} <br />\]<br />$ $TEX: \[<br />\begin{gathered}<br /> 5.{\text{ Elije dos de los siguientes limites y calculelos}} \hfill \\<br /> \hfill \\<br /> a)\mathop {\lim }\limits_{x \to 0} \frac{{x\sin x - x^2 \tan x}}<br />{{x^2 }} \hfill \\<br /> \hfill \\<br /> b)\mathop {\lim }\limits_{x \to \infty } \frac{{ - x^3 + 100x^2 + 1000}}<br />{{x^3 + x + 1}} \hfill \\<br /> \hfill \\<br /> C)\mathop {\lim }\limits_{x \to 0} \frac{{\tan 2x}}<br />{{\sin 3x}} \hfill \\<br /> \hfill \\<br /> 6.{\text{ Considera la funcion }}f:\mathbb{R} \to \mathbb{R}{\text{ definida por }}f(x) = \frac{{x + 2}}<br />{{x^2 + 3}}.{\text{ Grafica }}f{\text{ indicando}} \hfill \\<br /> {\text{intervalos de crecimiento}}{\text{, de decrecimiento}}{\text{, de concavidad}}{\text{, de convexidad}}{\text{, puntos de}} \hfill \\<br /> {\text{inflexion}}{\text{, maximos}}{\text{, minimos y asintotas}} \hfill \\ <br />\end{gathered} <br />\]<br />$ No pierdan su tiempo encontrando los pubntos de inflexion de la 6, queda un polinomio de grado 3 cuando se queria encontrarlos, asi que solo habia que hacer la grafica, a pura deduccion... mas rato me animare a postear la 1, 4 y 5.a que son las que elegi no responder en la prueba -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

LanderGuitar Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 11:32 AM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 568 Registrado: 8-July 08 Desde: Rancagua Miembro Nº: 29.447 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> \boxed{5 - c} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{\tan 2x}}<br />{{\sin 3x}} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{\tan 0}}<br />{{\sin 0}} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{0}<br />{0} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{\tan 2x}}<br />{{\sin 3x}} \hfill \\<br /> {\text{Ocupando L'hopital:}} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{2\sec ^2 2x}}<br />{{3\cos 3x}} \hfill \\<br /> \frac{{2\sec ^2 0}}<br />{{3\cos 0}} \hfill \\<br /> \boxed{\frac{2}<br />{3}} \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- $TEX: Educación 2020$ El 2% de los adolescentes no han fumado , si eres del "penoso" 98% que lo ha hecho, copia y pega esto en tu firma.

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 01:36 PM Publicado: #3
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(LanderGuitar @ Jul 25 2008, 12:23 PM) $TEX: \[<br />\begin{gathered}<br /> \boxed{5 - c} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{\tan 2x}}<br />{{\sin 3x}} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{\tan 0}}<br />{{\sin 0}} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{0}<br />{0} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{\tan 2x}}<br />{{\sin 3x}} \hfill \\<br /> {\text{Ocupando L'hopital:}} \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{2\sec ^2 2x}}<br />{{3\cos 3x}} \hfill \\<br /> \frac{{2\sec ^2 0}}<br />{{3\cos 0}} \hfill \\<br /> \boxed{\frac{2}<br />{3}} \hfill \\ <br />\end{gathered} <br />\]<br />$ no se puede ocupar L`hopital, no lo hemos visto aun ademas que es obvio que los limtes que se proponen no es la gracia resolverlos con l`hopital po xD $TEX: \[<br />\begin{gathered}<br /> \boxed{sp1} \hfill \\<br /> \hfill \\<br /> {\text{Sean X e Y las dimensiones del rectangulo}}{\text{, se sabe lo siguiente:}} \hfill \\<br /> \hfill \\<br /> 3\left( {2x + y} \right) = 10.000 \hfill \\<br /> y = \frac{{10.000}}<br />{3} - 2x \hfill \\<br /> \hfill \\<br /> {\text{Si }}A(x){\text{ fuese la funcion del area del rectangulo}} \hfill \\<br /> \hfill \\<br /> A(x) = xy \hfill \\<br /> A(x) = x\left( {\frac{{10.000}}<br />{3} - 2x} \right) \hfill \\<br /> A(x) = - 2x^2 + \frac{{10.000x}}<br />{3} \hfill \\<br /> \hfill \\<br /> {\text{Ahora se pueden tomar 2 caminos en lo que se llega a lo mismo}}{\text{, una es notar que}} \hfill \\<br /> A(x){\text{ es una funcion cuadratica con el coeficien }}a{\text{ negativo}}{\text{, por lo tanto su ramas se }} \hfill \\<br /> {\text{abren hacia abajo}}{\text{, por lo que su maximo son las coordenas del vertice}}{\text{, luego la solucion}} \hfill \\<br /> {\text{es directa}}{\text{. Y la otra forma}}{\text{, es la que hare}}{\text{, derivando }}A(x) \hfill \\ <br />\end{gathered} <br />\]<br />$ $TEX: \[<br />\begin{gathered}<br /> A'(x) = - 4x + \frac{{10.000}}<br />{3} \hfill \\<br /> \hfill \\<br /> - 4x + \frac{{10.000}}<br />{3} = 0 \Leftrightarrow x = \frac{{2.500}}<br />{3} \hfill \\<br /> \hfill \\<br /> {\text{Entonces es facil notar que en el punto }}\left( {\frac{{2.500}}<br />{3},A\left( {\frac{{2.500}}<br />{3}} \right)} \right){\text{ hay un maximo}}{\text{, ya que}} \hfill \\<br /> {\text{de }}\left( {{\text{ - }}\infty {\text{,}}\frac{{2.500}}<br />{3}} \right){\text{ }}A(x){\text{ crece y de }}\left( {\frac{{2.500}}<br />{3},\infty } \right){\text{ }}A(x){\text{ decrece}}{\text{. Con esto se determinan las}} \hfill \\<br /> {\text{dimensiones pedidas}} \hfill \\<br /> \hfill \\<br /> \boxed{{\text{sp4}}} \hfill \\<br /> \hfill \\<br /> {\text{Sea }}f:\mathbb{R} \to \mathbb{R};f(x) = \cos \left( {\frac{{\pi x}}<br />{2}} \right) - x^3 \hfill \\<br /> f{\text{ es continua por ser la resta de 2 funciones continuas}} \hfill \\<br /> \hfill \\<br /> f(0) = \cos 0 - 0 = 1 > 0 \hfill \\<br /> f(3) = \cos \left( {\frac{{3\pi }}<br />{2}} \right) - 3^3 = - 3^3 < 0 \hfill \\ <br />\end{gathered} <br />\]<br />$ $TEX: \[<br />\begin{gathered}<br /> {\text{Como }}f(0) \cdot f(3) < 0{\text{ por teorema de bolzano}} \Rightarrow \exists \xi \in \left( {{\text{0}}{\text{,3}}} \right) \subset \mathbb{R}{\text{ tal que}} \hfill \\<br /> \hfill \\<br /> f(\xi ) = 0 \Leftrightarrow 0 = \cos \left( {\frac{{\pi \xi }}<br />{2}} \right) - \xi ^3 \Leftrightarrow \boxed{\cos \left( {\frac{{\pi \xi }}<br />{2}} \right) = \xi ^3 } \hfill \\<br /> \hfill \\<br /> \boxed{sp5.a} \hfill \\<br /> \hfill \\<br /> \mathop {\lim }\limits_{x \to 0} \frac{{x\sin x - x^2 \tan x}}<br />{{x^2 }} = \mathop {\lim }\limits_{x \to 0} \frac{{\sin x}}<br />{x} - \tan x = 1 - 0 = \boxed1 \hfill \\<br /> \hfill \\<br /> \hfill \\ <br />\end{gathered} <br />\]<br />$ eso... si alguien tiene alguna duda con un ejercicio solo posteen Mensaje modificado por naxoobkn el Jul 25 2008, 06:24 PM -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

PXO11 Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 03:49 PM Publicado: #4
Matemático Grupo: Usuario FMAT Mensajes: 64 Registrado: 6-April 07 Miembro Nº: 4.985 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: <br />2) Sabemos que la distancia de un punto $(x,y)$ al origen viene dada por la funci\'on de 2 variables $f(x,y)=\sqrt{x^2+y^2}$ en particular la distancia al origen de un punto sobre la curva $f(x)=\dfrac{1}{x}$ viene dada por la funci\'on $f(x)=\sqrt{x^2+(\frac{1}{x})^2}$ analizemos m\'inimos de esta funci\'on sujeta a que $x>0$, primero que nada notemos que analizar m\'aximos y m\'inimos de la funci\'on $f(x)=\sqrt{x^2+(\frac{1}{x})^2}$ es equivalente a analizar m\'inimos de $f(x)=x^2+(\frac{1}{x})^2=x^2+\dfrac{1}{x^2}$ pues la ra\'iz cuadrada es una funci\'on estrictamente creciente en $[0,\infty]$ como $f'(x)=2x-\dfrac{2}{x^3}$ igualando a 0 se tiene\\$2x-\dfrac{2}{x^3}=0$\\$2x=\dfrac{2}{x^3}$\\$2x^4=2$\\$x^4=1$\\$x=1$, pues estamos considerando el primer cuadrante\\\\ Luego 1 es un punto cr\'itico de la funci\'on, adem\'as $f''(x)=2+\dfrac{6}{x^4}\Rightarrow f''(1)=2+\dfrac{6}{1^4}=8$ Luego por el criterio de la segunda derivada 1 es un m\'inimo por lo que el punto $(1,1)$ minimiza la distancia al origen.<br />$ Salu2. Mensaje modificado por PXO11 el Jul 25 2008, 05:10 PM

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 04:46 PM Publicado: #5
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> 3. \hfill \\<br /> \boxed{{\text{Solucion:}}} \hfill \\<br /> {\text{Consideremos la funcion :}} \hfill \\<br /> h\left( x \right) = f\left( x \right) - g\left( x \right) \hfill \\<br /> {\text{Diferenciable }}{\text{, puesto que esta conformada por la resta de dos funciones diferenciables}}{\text{.}} \hfill \\<br /> {\text{Asi:}} \hfill \\<br /> h'(x) = f'(x) - g'(x) \hfill \\<br /> \hfill \\<br /> {\text{Como por enunciado teniamos que }}{\text{.}} \hfill \\<br /> f'(x) \geqslant g'(x) \Rightarrow f'(x) - g'(x) \geqslant 0,\forall x \in \mathbb{R} \hfill \\<br /> {\text{Por lo tanto :}} \hfill \\<br /> h'(x) \geqslant 0,\forall x \in \mathbb{R}{\text{ }}\left( * \right) \hfill \\<br /> \hfill \\<br /> {\text{A la vez : }}h\left( 0 \right) = f\left( 0 \right) - g\left( 0 \right) = 0 \hfill \\<br /> \hfill \\<br /> {\text{Luego como }}h{\text{ es continua en }}\left[ {0,x} \right]{\text{ y diferenciable en }}\left( {0,x} \right){\text{ }}{\text{, }} \hfill \\<br /> {\text{asi que existe un }}c \in \left( {0,x} \right){\text{ }}{\text{, tal que }}{\text{.}} \hfill \\<br /> \hfill \\<br /> h'© = \frac{{h\left( 0 \right) - h\left( x \right)}}<br />{{0 - x}} = \frac{{f\left( x \right) - g\left( x \right)}}<br />{x} \hfill \\<br /> {\text{Por }}\left( * \right){\text{ }}{\text{, tendriamos que :}} \hfill \\<br /> h'© \geqslant 0 \Rightarrow \frac{{f\left( x \right) - g\left( x \right)}}<br />{x} \geqslant 0 \hfill \\<br /> {\text{Como }}x > 0 \hfill \\<br /> f(x) - g(x) \geqslant 0 \hfill \\<br /> f\left( x \right) \geqslant g(x){\text{ }}{\text{, }}\forall x > 0 \hfill \\<br /> \hfill \\<br /> {\text{Q}}{\text{.e}}{\text{.d}} \hfill \\<br /> \hfill \\<br /> {\text{Saludos}} \hfill \\ <br />\end{gathered} <br />\]$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 06:06 PM Publicado: #6
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Uchiha Itachi @ Jul 25 2008, 05:37 PM) $TEX: \[<br />\begin{gathered}<br /> 3. \hfill \\<br /> \boxed{{\text{Solucion:}}} \hfill \\<br /> {\text{Consideremos la funcion :}} \hfill \\<br /> h\left( x \right) = f\left( x \right) - g\left( x \right) \hfill \\<br /> {\text{Diferenciable }}{\text{, puesto que esta conformada por la resta de dos funciones diferenciables}}{\text{.}} \hfill \\<br /> {\text{Asi:}} \hfill \\<br /> h'(x) = f'(x) - g'(x) \hfill \\<br /> \hfill \\<br /> {\text{Como por enunciado teniamos que }}{\text{.}} \hfill \\<br /> f'(x) \geqslant g'(x) \Rightarrow f'(x) - g'(x) \geqslant 0,\forall x \in \mathbb{R} \hfill \\<br /> {\text{Por lo tanto :}} \hfill \\<br /> h'(x) \geqslant 0,\forall x \in \mathbb{R}{\text{ }}\left( * \right) \hfill \\<br /> \hfill \\<br /> {\text{A la vez : }}h\left( 0 \right) = f\left( 0 \right) - g\left( 0 \right) = 0 \hfill \\<br /> \hfill \\<br /> {\text{Luego como }}h{\text{ es continua en }}\left[ {0,x} \right]{\text{ y diferenciable en }}\left( {0,x} \right){\text{ }}{\text{, }} \hfill \\<br /> {\text{asi que existe un }}c \in \left( {0,x} \right){\text{ }}{\text{, tal que }}{\text{.}} \hfill \\<br /> \hfill \\<br /> h'© = \frac{{h\left( 0 \right) - h\left( x \right)}}<br />{{0 - x}} = \frac{{f\left( x \right) - g\left( x \right)}}<br />{x} \hfill \\<br /> {\text{Por }}\left( * \right){\text{ }}{\text{, tendriamos que :}} \hfill \\<br /> h'© \geqslant 0 \Rightarrow \frac{{f\left( x \right) - g\left( x \right)}}<br />{x} \geqslant 0 \hfill \\<br /> {\text{Como }}x > 0 \hfill \\<br /> f(x) - g(x) \geqslant 0 \hfill \\<br /> f\left( x \right) \geqslant g(x){\text{ }}{\text{, }}\forall x > 0 \hfill \\<br /> \hfill \\<br /> {\text{Q}}{\text{.e}}{\text{.d}} \hfill \\<br /> \hfill \\<br /> {\text{Saludos}} \hfill \\ <br />\end{gathered} <br />\]$ que bonito sale con el TVM, pero nota que es mas facil decir que h es creciente, o sea que si x>0 implica que h(x)>h(0), la solucion es directa de ahi -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

Guía Rojo Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 07:56 PM Publicado: #7
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 903 Registrado: 28-May 05 Desde: Santiago, Chile Miembro Nº: 69 Nacionalidad: Colegio/Liceo: Sexo:	CITA(naxoobkn @ Jul 23 2008, 08:42 PM) $TEX: \[<br />\begin{gathered}<br /> 5.b)\mathop {\lim }\limits_{x \to \infty } \frac{{ - x^3 + 100x^2 + 1000}}<br />{{x^3 + x + 1}} \hfill \\<br /> \hfill \\<br /> 5.c)\mathop {\lim }\limits_{x \to 0} \frac{{\tan 2x}}<br />{{\sin 3x}} \hfill \\<br />\end{gathered} <br />\]<br />$ $TEX: \noindent $\displaystyle\lim_{x\to \infty }\dfrac{-x^3+100x^2+1000}{x^3+x+1}=\displaystyle\lim_{x\to \infty }\dfrac{-1+\dfrac{100}{x}+\dfrac{1000}{x^2}}{1+\dfrac{1}{x^2}+\dfrac{1}{x^3}}=-1$\\<br />\\<br />\\<br />$\displaystyle\lim_{x\to 0}\dfrac{\tan 2x}{\sin 3x}=\displaystyle\lim_{x\to 0}\dfrac{\sin 2x}{2x}\cdot \dfrac{3x}{\sin 3x}\cdot \dfrac{2}{3\cos 2x}=\dfrac{2}{3}$$ -------------------- Bachiller en Ciencias (ex) Estudiante de Medicina Estudiante de Ingeniería Civil de Industrias, diploma en Ingeniería Matemática Pontificia Universidad Católica de Chile "El espíritu del matemático busca la belleza del pensamiento, y esa va de la mano con la filosofía." - Héctor Hardy Pastén Vásquez "¿Qué te hace pensar que si tienes dos elementos de un conjunto con otros dos elementos de otro conjunto se origina uno nuevo con cuatro? Es sólo el sentido común, no te imaginas que los elementos aparecen de la nada, pero resulta que con el sólo hecho de cuestionar el sentido común, se podría afirmar que cuando juntas cuatro elementos aparecen miles de elementos más. Y si lo demuestras, todo se basa en la razón." - Alonso Duque Vega, razonador por excelencia $TEX: $S=1+dfrac{1}{2}+dfrac{1}{3}+dfrac{1}{4}+dfrac{1}{5}+ldots$$ Esta serie converge! He encontrado una maravillosa demostración de esto, pero no cabe en la estrechez de esta firma...

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 25 2008, 08:09 PM Publicado: #8
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	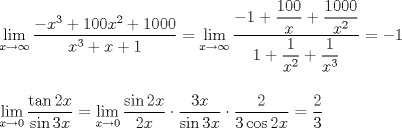CITA(Guía Rojo @ Jul 25 2008, 08:46 PM) $TEX: \noindent $\displaystyle\lim_{x\to \infty }\dfrac{-x^3+100x^2+1000}{x^3+x+1}=\displaystyle\lim_{x\to \infty }\dfrac{-1+\dfrac{100}{x}+\dfrac{1000}{x^2}}{1+\dfrac{1}{x^2}+\dfrac{1}{x^3}}=-1$\\<br />\\<br />\\<br />$\displaystyle\lim_{x\to 0}\dfrac{\tan 2x}{\sin 3x}=\displaystyle\lim_{x\to 0}\dfrac{\sin 2x}{2x}\cdot \dfrac{3x}{\sin 3x}\cdot \dfrac{2}{3\cos 2x}=\dfrac{2}{3}$$ no estaria demas decir que en la primera factorizaste por $TEX: $x^3$$ o que amplificaste por $TEX: $\dfrac{1}{x^3}$$ igual estaban sencillos los limites o sea, en comparacion con los que hay en el sector de propuestos -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

Lican Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 26 2008, 07:41 PM Publicado: #9
Dios Matemático Grupo: Usuario FMAT Mensajes: 384 Registrado: 3-June 07 Miembro Nº: 6.396 Nacionalidad: Universidad: Sexo:	estaba facil, pero la 6 era un asco, mucho atado .. estaba más dificil en comparación a otras globales.

NAITSABES EPILEF Ver Perfil del Miembro Ver los mensajes de este miembro	Jul 27 2008, 12:58 AM Publicado: #10
Maestro Matemático Grupo: Usuario FMAT Mensajes: 105 Registrado: 26-February 08 Desde: desde mi casa xD Miembro Nº: 15.916 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	oye nacho en la 6, es el denominador pero al cuadrado

« Posterior · Matemáticas 1 · Anterior »

2 Páginas:

1 2 >

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 09:10 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.