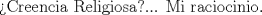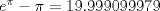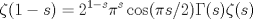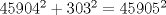Homomorfismo, isomorfismo y kernel

Foro fmat.cl > Programa Enseñanza Universitaria > Consultas > Banco de Problemas Resueltos > Álgebra > Álgebra Lineal

Homomorfismo, isomorfismo y kernel

Opciones

Amadís Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 26 2008, 06:20 PM Publicado: #1
Dios Matemático Grupo: Usuario FMAT Mensajes: 365 Registrado: 27-March 08 Desde: La Punta, Mostazal, VI Región, insondable universo Miembro Nº: 18.086 Universidad: Sexo:	$TEX: % MathType!MTEF!2!1!+-<br />% feaafiart1ev1aaatCvAUfeBSjuyZL2yd9gzLbvyNv2CaerbuLwBLn<br />% hiov2DGi1BTfMBaeXatLxBI9gBaerbd9wDYLwzYbItLDharqqtubsr<br />% 4rNCHbGeaGqiVu0Je9sqqrpepC0xbbL8F4rqqrFfpeea0xe9Lq-Jc9<br />% vqaqpepm0xbba9pwe9Q8fsY-rqaqpepae9pg0FirpepeKkFr0xfr-x<br />% fr-xb9adbaqaaeGaciGaaiaabeqaamaabaabaaGceaqabeaacaqGtb<br />% GaaeyzaiaabggacaqGGaGaamivaiaacQdacaWGsbWaaWbaaSqabeaa<br />% caaIZaaaaOGaeyOKH4QaamOuamaaCaaaleqabaGaaGynaaaakiaabc<br />% cacaqG0bGaaeyyaiaabYgacaqGGaGaaeyCaiaabwhacaqGLbGaaeOo<br />% aiaabccacaWGubGaaiikaiaadIhacaGGSaGaamyEaiaacYcacaWG6b<br />% Gaaiykaiabg2da9iaacIcacaWG4bGaey4kaSIaamyEaiaacYcacaWG<br />% 4bGaeyOeI0IaamyEaiaacYcacaaI0aGaamiEaiabgkHiTiaadMhaca<br />% GGSaWaaSaaaeaacaWG4baabaGaaGOmaaaacqGHRaWkdaWcaaqaaiaa<br />% dMhaaeaacaaIYaaaaiaacYcacaWG4bGaeyOeI0IaaG4maiaadMhaca<br />% GGPaaabaGaamyAaiaacMcacaqGebGaaeyzaiaabshacaqGLbGaaeOC<br />% aiaab2gacaqGPbGaaeOBaiaabggacaqGYbGaaeiiaiaabohacaqGPb<br />% GaaeiiaiaadsfacaqGGaGaae4yaiaabwhacaqGTbGaaeiCaiaabYga<br />% caqGLbGaaeiiaiaabogacaqGVbGaaeOBaiaabccacaqGZbGaaeyzai<br />% aabkhacaqGGaGaaeiAaiaab+gacaqGTbGaae4Baiaab2gacaqGVbGa<br />% aeOCaiaabAgacaqGPbGaae4Caiaab2gacaqGVbGaaeiiaiaabsgaca<br />% qGLbGaaeiiaiaabEgacaqGYbGaaeyDaiaabchacaqGVbGaae4Caiaa<br />% b6caaeaacaqGPbGaaeyAaiaabMcacaqGGaGaaeiraiaabwgacaqG0b<br />% GaaeyzaiaabkhacaqGTbGaaeyAaiaab6gacaqGHbGaaeOCaiaabcca<br />% caqGZbGaaeyAaiaabccacaqGubGaaeiiaiaabwgacaqGZbGaaeiiai<br />% aabMgacaqGZbGaae4Baiaab2gacaqGVbGaaeOCaiaabAgacaqGPbGa<br />% ae4Caiaab2gacaqGVbGaaeOlaaqaaiaabMgacaqGPbGaaeyAaiaabM<br />% cacaqGGaGaaeiraiaabwgacaqG0bGaaeyzaiaabkhacaqGTbGaaeyA<br />% aiaab6gacaqGHbGaaeOCaiaabccacaqGubWaaWbaaSqabeaacaqGTa<br />% Gaaeymaaaakiaac6caaeaacaWGPbGaamODaiaacMcacaqGibGaaeyy<br />% aiaabYgacaqGSbGaaeyyaiaabkhacaqGGaGaaeyzaiaabYgacaqGGa<br />% Gaae4saiaabwgacaqGYbGaaeOBaiaabwgacaqGSbGaaeiiaiaabsga<br />% caqGLbGaaeiiaiaabsfacaqGGaaaaaa!DB34!<br />\[<br />\begin{gathered}<br /> {\text{Sea }}T:R^3 \to R^5 {\text{ tal que: }}T(x,y,z) = (x + y,x - y,4x - y,\frac{x}<br />{2} + \frac{y}<br />{2},x - 3y) \hfill \\<br /> i){\text{Determinar si }}T{\text{ cumple con ser homomorfismo de grupos}}{\text{.}} \hfill \\<br /> {\text{ii) Determinar si T es isomorfismo}}{\text{.}} \hfill \\<br /> {\text{iii) Determinar T}}^{{\text{ - 1}}} . \hfill \\<br /> iv){\text{Hallar el Kernel de T }} \hfill \\ <br />\end{gathered} <br />\]$ -------------------- Soy luz, brisa, magia y dicha. ¡Que la Tierra cante silencios y que el mar bese con su furia mi piel! ¡De Alejandría a Sevilla y de Milán a Glasgow canta mi espada!

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 26 2008, 07:27 PM Publicado: #2
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> \bullet {\text{Demostraremos que es homomorfismo de grupo :}} \hfill \\<br /> {\text{Sea : }} \hfill \\<br /> u = \left( {a,b,c} \right) \in \mathbb{R}^3 \hfill \\<br /> v = \left( {d,e,f} \right) \in \mathbb{R}^3 \hfill \\<br /> {\text{Entonces :}} \hfill \\<br /> {\text{T}}\left( {u + v} \right) = {\text{T}}\left( {\left( {a,b,c} \right) + \left( {d,e,f} \right)} \right) \hfill \\<br /> {\text{T}}\left( {u + v} \right) = {\text{T}}\left( {a + d,b + e,c + f} \right) \hfill \\<br /> {\text{T}}\left( {u + v} \right) = \left( {a + d + b + e,a + d - b - e,4a + 4d - b - e,\frac{{a + d}}<br />{2} + \frac{{b + e}}<br />{2},a + d - 3b - 3e} \right) \hfill \\<br /> {\text{T}}\left( {u + v} \right) = \left( {a + b,a - b,4a - b,\frac{a}<br />{2} + \frac{b}<br />{2},a - 3b} \right) + \left( {d + e,d - e,4d - e,\frac{d}<br />{2} + \frac{e}<br />{2},d - 3e} \right) \hfill \\<br /> {\text{T}}\left( {u + v} \right) = {\text{T}}\left( {a,b,c} \right) + {\text{T}}\left( {d,e,f} \right) \hfill \\<br /> {\text{T}}\left( {u + v} \right) = {\text{T}}\left( u \right) + {\text{T}}\left( v \right) \hfill \\<br /> \hfill \\<br /> \therefore {\text{T es homomorfismo de grupo }}{\text{.}} \hfill \\ <br />\end{gathered} <br />\]$ $TEX: \[<br />\begin{gathered}<br /> {\text{mmm seguro que no aparece z en }}T\left( {x,y,z} \right){\text{ }}{\text{, porque de ser asi }}{\text{, cuando vemos si es}} \hfill \\<br /> {\text{isomorfismo o no }}{\text{, en la etapa de inyectividad }}{\text{, se cumple que }}x = y = 0{\text{ }}{\text{, pero z puede}} \hfill \\<br /> {\text{tomar cualquier valor }}{\text{, y se seguiria cumpliendo que : }}T\left( u \right) = \left\{ {e_{\mathbb{R}^5 } } \right\} \hfill \\<br /> \hfill \\<br /> {\text{Saludos!!}} \hfill \\ <br />\end{gathered} <br />\]$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

Uchiha Itachi Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 26 2008, 07:48 PM Publicado: #3
Staff FMAT Grupo: Super Moderador Mensajes: 4.857 Registrado: 2-January 08 Miembro Nº: 14.268 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> \bullet {\text{Para que T sea ismorfismo }}{\text{, debe ser homomorfismo biyectivo }}{\text{, por lo que :}} \hfill \\<br /> \circ {\text{Inyectividad : T es inyectivo}} \Leftrightarrow \ker \left( T \right) = \left\{ {e_{\mathbb{R}^5 } } \right\} \hfill \\<br /> {\text{Entonces :}} \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge T\left( u \right) = \left( {0,0,0,0,0} \right) \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge T\left( {a,b,c} \right) = (0,0,0,0,0) \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge \left( {a + b,a - b,4a - b,\frac{a}<br />{2} + \frac{b}<br />{2},a - 3b} \right) = \left( {0,0,0,0,0} \right) \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \left. {\underline {\, <br /> \begin{gathered}<br /> a + b = 0 \hfill \\<br /> a - b = 0 \hfill \\<br /> 4a - b = 0 \hfill \\<br /> a + b = 0 \hfill \\<br /> a - 3b = 0 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge \left[ {a = b = 0 \wedge c \in \mathbb{R}} \right] \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow \ker \left( T \right) = \left( {0,0,c} \right):c \in \mathbb{R} \hfill \\<br /> \ker \left( T \right) = \left\langle {\left( {0,0,1} \right)} \right\rangle \ne \left( {0,0,0} \right) \hfill \\<br /> \hfill \\<br /> \therefore {\text{ T no es inyectiva }}{\text{, por lo que no es isomorfismo de grupo }} \hfill \\<br /> \hfill \\<br /> {\text{Por favor que alguien confirme <img src="style_emoticons/default/smile.gif" style="vertical-align:middle" emoid=":)" border="0" alt="smile.gif" /> gracias }} \hfill \\<br /> \hfill \\<br /> {\text{Saludines}} \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- Candidato a doctor en Cs. De la ingeniería mención modelamiento matemático, DIM. Universidad de Chile Magíster en ciencias mención matemática, Profesor de estado en matemáticas y computación, Licenciado en educación matemáticas y computación, USACH

ediiii Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 21 2013, 07:01 PM Publicado: #4
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 581 Registrado: 23-September 09 Desde: santiago Miembro Nº: 59.245 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Uchiha Itachi @ Jun 26 2008, 09:48 PM) $TEX: \[<br />\begin{gathered}<br /> \bullet {\text{Para que T sea ismorfismo }}{\text{, debe ser homomorfismo biyectivo }}{\text{, por lo que :}} \hfill \\<br /> \circ {\text{Inyectividad : T es inyectivo}} \Leftrightarrow \ker \left( T \right) = \left\{ {e_{\mathbb{R}^5 } } \right\} \hfill \\<br /> {\text{Entonces :}} \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge T\left( u \right) = \left( {0,0,0,0,0} \right) \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge T\left( {a,b,c} \right) = (0,0,0,0,0) \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge \left( {a + b,a - b,4a - b,\frac{a}<br />{2} + \frac{b}<br />{2},a - 3b} \right) = \left( {0,0,0,0,0} \right) \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \left. {\underline {\, <br /> \begin{gathered}<br /> a + b = 0 \hfill \\<br /> a - b = 0 \hfill \\<br /> 4a - b = 0 \hfill \\<br /> a + b = 0 \hfill \\<br /> a - 3b = 0 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow u = \left( {a,b,c} \right) \in \mathbb{R}^3 \wedge \left[ {a = b = 0 \wedge c \in \mathbb{R}} \right] \hfill \\<br /> u \in \ker \left( T \right) \Leftrightarrow \ker \left( T \right) = \left( {0,0,c} \right):c \in \mathbb{R} \hfill \\<br /> \ker \left( T \right) = \left\langle {\left( {0,0,1} \right)} \right\rangle \ne \left( {0,0,0} \right) \hfill \\<br /> \hfill \\<br /> \therefore {\text{ T no es inyectiva }}{\text{, por lo que no es isomorfismo de grupo }} \hfill \\<br /> \hfill \\<br /> {\text{Por favor que alguien confirme <img src="style_emoticons/default/smile.gif" style="vertical-align:middle" emoid=":)" border="0" alt="smile.gif" /> gracias }} \hfill \\<br /> \hfill \\<br /> {\text{Saludines}} \hfill \\ <br />\end{gathered} <br />\]<br />$ Se que este tema tiene 5 años de edad XD... pero bueno... Me dio que no era isomorfismo, PERO, me dio que era inyectiva y no sobreyectiva =/... -------------------- $TEX: <!--fonto:Century Gothic--><span style="font-family:Century Gothic"><!--/fonto-->¿Creencia Religiosa?<!--fontc--></span><!--/fontc-->... Mi raciocinio.$

ediiii Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 21 2013, 11:29 PM Publicado: #6
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 581 Registrado: 23-September 09 Desde: santiago Miembro Nº: 59.245 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	Estimado, entiendo hasta donde dice "(0,0,c) : c pert. R", pero porque luego queda Ker(T)= (0,0,1), es por ponerle un valor cualquiera a c?... gracias =) -------------------- $TEX: <!--fonto:Century Gothic--><span style="font-family:Century Gothic"><!--/fonto-->¿Creencia Religiosa?<!--fontc--></span><!--/fontc-->... Mi raciocinio.$

ediiii Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 22 2013, 10:23 AM Publicado: #8
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 581 Registrado: 23-September 09 Desde: santiago Miembro Nº: 59.245 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(2.718281828 @ Aug 22 2013, 01:51 AM) aaah... lo que quiere decir es que el kernel es el espacio generado por (0,0,1), esto porque lo puedes ver asi: (0,0,c)=c(0,0,1), debido a que c es libre...} saludos. Perfect =)... gracias!!! -------------------- $TEX: <!--fonto:Century Gothic--><span style="font-family:Century Gothic"><!--/fonto-->¿Creencia Religiosa?<!--fontc--></span><!--/fontc-->... Mi raciocinio.$

« Posterior · Álgebra Lineal · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 3rd April 2025 - 09:24 PM