Entrenamiento Algebra v2.0 - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Sector Preparación Olimpiadas > Sector PreOlímpico > Álgebra > Material de Entrenamiento

6 Páginas:

« < 2 3 4 5 6 >

Reply to this topic

Start new topic

Entrenamiento Algebra v2.0, [Medio]

S. E. Puelma Moy... S. E. Puelma Moya Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 25 2008, 01:19 PM Publicado: #31
Dios Matemático Supremo Grupo: Administrador Mensajes: 2.706 Registrado: 13-May 05 Desde: Santiago de Chile Miembro Nº: 10 Nacionalidad: Colegio/Liceo: Sexo:	CITA(69___ @ Aug 16 2008, 12:20 AM) $TEX: \[<br />\begin{gathered}<br /> \boxed{Sp_{32} } \hfill \\<br /> \left( {x,y} \right) \in \mathbb{N},{\text{ }}es{\text{ }}decir,{\text{ }}las{\text{ }}soluciones{\text{ }}son{\text{ }}positivas. \hfill \\<br /> x^2 + 361 = y^2 \hfill \\<br /> 361 = y^2 - x^2 \hfill \\<br /> 361 = \left( {y - x} \right)\left( {y + x} \right) \hfill \\<br /> Notemos{\text{ }}que{\text{ }}361{\text{ }}es{\text{ }}primo{\text{ }}y{\text{ }}sus{\text{ }}divisores{\text{ }}positivos{\text{ }}son \hfill \\<br /> 1 \wedge 361,{\text{ }}luego{\text{ }}tenemos{\text{ }}los{\text{ }}siguientes{\text{ }}sistemas: \hfill \\<br /> (1) \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> (i){\text{ }}y + x = 361 \hfill \\<br /> (ii){\text{ }}y - x = 1 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> Sumamos{\text{ }}(i) \wedge (ii),{\text{ }}y{\text{ }}obtenemos \hfill \\<br /> 2y = 362 \Rightarrow \boxed{y = 181} \hfill \\<br /> Ahora{\text{ }}reemplazamos{\text{ }}en{\text{ }}(i){\text{ }}de{\text{ }}donde{\text{ }}se{\text{ }}obtiene{\text{ }}que{\text{ }} \hfill \\<br /> x = 180. \hfill \\<br /> Ahora{\text{ }}bien,{\text{ }}trabajamos{\text{ }}con{\text{ }}el{\text{ }}sistema{\text{ }}(2) \hfill \\<br /> {\text{(2)}} \hfill \\<br /> \left. {\underline {\, <br /> \begin{gathered}<br /> (i){\text{ }}y + x = 1 \hfill \\<br /> (ii){\text{ }}y - x = 361 \hfill \\ <br />\end{gathered} \,}}\! \right\| \hfill \\<br /> Sumamos{\text{ }}(i) \wedge (ii),{\text{ }}y{\text{ }}obtenemos \hfill \\<br /> 2y = 362 \Rightarrow \boxed{y = 181} \hfill \\<br /> Ahora{\text{ }}reemplazamos{\text{ }}en{\text{ }}(i){\text{ }}de{\text{ }}donde{\text{ }}se{\text{ }}obtiene{\text{ }}que{\text{ }} \hfill \\<br /> x = 180,{\text{ }}y{\text{ }}como{\text{ }}en{\text{ }}(1){\text{ }}y{\text{ }}(2){\text{ }}obtuvimos{\text{ }}las{\text{ }}mismas{\text{ }}soluciones,{\text{ }}y{\text{ }}todas \hfill \\<br /> positivas,{\text{ }}concluimos{\text{ }}que{\text{ }}\left( {x,y} \right) = \left( {180,181} \right) \in \mathbb{N} \hfill \\ <br />\end{gathered} <br />\]$ Si recuerdo bien, hay un propuesto de makmat que se resuelve de la misma manera. Con respecto a mi solucion, no me convencio mucho desde un principio al resolverlo, ya que llegaba a las mismas soluciones, pero será. Salu2 PD:Buenos los problemas, sirven para preparacion para ONM. Segunda solución donde tengo algo que decir (en general, comentaré cuando encuentre soluciones incorrectas, o bien cuando encuentre soluciones mucho más largas de lo necesario, como fue mi comentario anterior). El primer caso está bien resuelto, pero en el segundo caso tienes que x=-180, por lo tanto no hay solución con los requisitos pedidos. Si se pidieran todas las soluciones enteras, no olvides que debes considerar todas las factorizaciones, tanto con factores positivos como con factores negativos. Un saludo -------------------- Sebastián Elías Puelma Moya Administrador FMAT

S. E. Puelma Moy... S. E. Puelma Moya Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 25 2008, 01:23 PM Publicado: #32
Dios Matemático Supremo Grupo: Administrador Mensajes: 2.706 Registrado: 13-May 05 Desde: Santiago de Chile Miembro Nº: 10 Nacionalidad: Colegio/Liceo: Sexo:	CITA(69___ @ Aug 16 2008, 01:56 AM) Perdona MisTer_BurNS por robarte la solucion $TEX: \[<br />\begin{gathered}<br /> \boxed{Sp_{26} } \hfill \\<br /> \left. {\underline {\, <br /> {Solucion{\text{ }}1} \,}}\! \right\| \hfill \\<br /> \sqrt {1000000 \bullet 1000001 \bullet 1000002 \bullet 1000003 + 1} \hfill \\<br /> = \sqrt {2000\left( {2000 + 3} \right)\left( {2000 + 2} \right)\left( {2000 + 1} \right) + 1} \hfill \\<br /> = \sqrt {\left( {2000^2 + 3 \bullet 2000} \right)\left( {2000^2 + 3 \bullet 2000 + 2} \right) + 1} \hfill \\<br /> = \sqrt {\left( {2000^2 + 3 \bullet 2000} \right)^2 + 2\left( {2000^2 + 3 \bullet 2000} \right) + 1} \hfill \\<br /> = \sqrt {\left( {\left( {2000^2 + 3 \bullet 2000} \right) + 1} \right)^2 } \hfill \\<br /> = \left( {2000^2 + 3 \bullet 2000} \right) + 1 \hfill \\<br /> = 4.006.001 \hfill \\<br /> By{\text{ }}MisTer\_BurNS \hfill \\ <br />\end{gathered} <br />\]$ Salu2 Si bien estabas "robando" la solución de MisTer_BurNS, observa qué ha sucedido entre la primera y la segunda línea... creo que hay algo por corregir. El problema con 2000 x 2001 x 2002 x 2003 + 1 lo puedes encontrar (si mi memoria no falla) en la primera prueba del CMAT 2003 (es decir, en el origen mismo del CMAT). Sin dudas es un ejercicio que no puede faltar en un entrenamiento básico. -------------------- Sebastián Elías Puelma Moya Administrador FMAT

DoomH~ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 25 2008, 08:22 PM Publicado: #33
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.429 Registrado: 6-October 07 Miembro Nº: 10.987	CITA(xsebastian @ Oct 25 2008, 02:23 PM) Si bien estabas "robando" la solución de MisTer_BurNS, observa qué ha sucedido entre la primera y la segunda línea... creo que hay algo por corregir. La verdad, no es por discrepar, pero lo que hice fue solo mostrar la solucion, mi idea nunca fue robarla, sino que "copie" la solucion solamente. Cuando vi el problema, se me ocurrio la idea de usar una Variable Auxiliar, y factorizando de manera adecuada formar un cuadrado de Binomio para extraer la raiz. CITA(xsebastian @ Oct 25 2008, 02:23 PM) El problema con 2000 x 2001 x 2002 x 2003 + 1 lo puedes encontrar (si mi memoria no falla) en la primera prueba del CMAT 2003 (es decir, en el origen mismo del CMAT). Sin dudas es un ejercicio que no puede faltar en un entrenamiento básico. He visto este problema, es bonito, si recuerdo bien, la solucion fue dada por Caetano->( ) Salu2, y gracias por darte el tiempo de revisar las soluciones. -------------------- CHAO.

S. E. Puelma Moy... S. E. Puelma Moya Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 27 2008, 02:13 PM Publicado: #34
Dios Matemático Supremo Grupo: Administrador Mensajes: 2.706 Registrado: 13-May 05 Desde: Santiago de Chile Miembro Nº: 10 Nacionalidad: Colegio/Liceo: Sexo:	Al principio, tú usaste el verbo "robar", por eso yo seguí usando ese verbo. Sin embargo, no te estoy tratando de "ladrón de soluciones" porque (en este caso) no corresponde. La observación que quise hacer, es la siguiente: En el problema, se debe trabajar con la expresión n(n+1)(n+2)(n+3)+1, cuando n=1000000 Hasta la primera línea trabajaste con n=1000000, pero a partir de la segunda línea trabajaste con n=2000 (es decir, el problema de dicho CMAT) Lo único que debes corregir es el error de tipeo Un saludo, y sigue entrenando , en lo posible aquí estamos para guiar su entrenamiento -------------------- Sebastián Elías Puelma Moya Administrador FMAT

DoomH~ Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2008, 11:05 AM Publicado: #35
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.429 Registrado: 6-October 07 Miembro Nº: 10.987	Me acordé que quedaban artos problemas sin resolver, asi que ahora sigo. $TEX: \[<br />\begin{gathered}<br /> \boxed{Sp_{16} } \hfill \\<br /> {\text{Sea }}k{\text{ el cuociente de la division}} \hfill \\<br /> k = \frac{{a^{128} - b^{128} }}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)\left( {a^{16} + b^{16} } \right)\left( {a^{32} + b^{32} } \right)\left( {a^{64} + b^{64} } \right)}} \hfill \\<br /> k = \frac{{\left( {a^{64} - b^{64} } \right)\left( {a^{64} + b^{64} } \right)}}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)\left( {a^{16} + b^{16} } \right)\left( {a^{32} + b^{32} } \right)\left( {a^{64} + b^{64} } \right)}} \hfill \\<br /> k = \frac{{a^{64} - b^{64} }}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)\left( {a^{16} + b^{16} } \right)\left( {a^{32} + b^{32} } \right)}} \hfill \\<br /> k = \frac{{\left( {a^{32} - b^{32} } \right)\left( {a^{32} + b^{32} } \right)}}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)\left( {a^{16} + b^{16} } \right)\left( {a^{32} + b^{32} } \right)}} \hfill \\<br /> k = \frac{{a^{32} - b^{32} }}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)\left( {a^{16} + b^{16} } \right)}} \hfill \\<br /> k = \frac{{\left( {a^{16} - b^{16} } \right)\left( {a^{16} + b^{16} } \right)}}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)\left( {a^{16} + b^{16} } \right)}} \hfill \\<br /> k = \frac{{a^{16} - b^{16} }}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)}} \hfill \\<br /> k = \frac{{\left( {a^8 - b^8 } \right)\left( {a^8 + b^8 } \right)}}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)\left( {a^8 + b^8 } \right)}} \hfill \\<br /> k = \frac{{a^8 - b^8 }}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)}} \hfill \\<br /> k = \frac{{\left( {a^4 - b^4 } \right)\left( {a^4 + b^4 } \right)}}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)\left( {a^4 + b^4 } \right)}} \hfill \\<br /> k = \frac{{a^4 - b^4 }}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)}} \hfill \\<br /> k = \frac{{\left( {a^2 - b^2 } \right)\left( {a^2 + b^2 } \right)}}<br />{{\left( {a + b} \right)\left( {a^2 + b^2 } \right)}} \hfill \\<br /> k = \frac{{a^2 - b^2 }}<br />{{\left( {a + b} \right)}} \hfill \\<br /> k = \frac{{\left( {a - b} \right)\left( {a + b} \right)}}<br />{{\left( {a + b} \right)}} \hfill \\<br /> k = a - b \hfill \\<br /> \therefore {\text{ El cuociente de la division del enunciado es }}a - b. \hfill \\ <br />\end{gathered} <br />\]$ Era solo Algebra, nada mas. Salu2 -------------------- CHAO.

negroo Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2008, 11:37 AM Publicado: #36
Matemático Grupo: Usuario FMAT Mensajes: 32 Registrado: 8-November 08 Desde: chile Miembro Nº: 38.115 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: Determine todos pares de enteros $(m,n)$ tales que $m\cdot n \ge 0$ y :<br />$m^3 + n^3 +99mn =33^3$ y esto nos recuerda a la factorizacion: $a^3+b^3+c^3 -3abc =(a+b+c)(a^2+b^2+c^2-ab-bc-ca)$. Ahora $0=m^3+n^3-33^3-3(m)(n)(-33)=(m+n-33)(m^2+n^2+33^2-mn+33m+33n)$.Ahora sirven todos los pares$(m,n)$. Tales que $m+n=33$ con m y n enteros no negativos ($mn\ge 0$).Ahora veamos el caso $m^2+n^2+33^2=mn-33m-33n$ pero: $a^2+b^2+c^2 \ge ab+bc+ca$ con igualdad si solo si $a=b=c$ (no importa si son positivos o negativos) entonces: $m=n=-33$ es la otra solucion.Por lo tanto todos los pares $(m,n)$ son:<br />$(-33,-33)$ y los tales que $m+n=33$ con m y n enteros no negativos.$

negroo Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2008, 11:52 AM Publicado: #37
Matemático Grupo: Usuario FMAT Mensajes: 32 Registrado: 8-November 08 Desde: chile Miembro Nº: 38.115 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: Sean a,b,c los lados de un triangulo. Demostrar que : $a^3+b^3+3abc > c^3$.<br />Ahora: Hay que demostrar que $a^3+b^3-c^3+3abc=(a+b-c)(a^2+b^2+c^2+ab-bc-ca)>0$.Por desigualdad triangular $a+b>c$. falta demostrar que $a^2+b^2+c^2>bc+ca-ab$.Pero: $a^2+b^2+c^2 \ge ab+bc+ca>bc+ca-ab $. ahora: $a+b-c>0$ y $a^2+b^2+c^2+ab-bc-ca>0$. Multiplicando se llega a lo pedido$ Mensaje modificado por negroo el Dec 5 2008, 11:52 AM

negroo Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2008, 12:08 PM Publicado: #38
Matemático Grupo: Usuario FMAT Mensajes: 32 Registrado: 8-November 08 Desde: chile Miembro Nº: 38.115 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: Sean $x,y,z$ Reales tales que $x+y+z=0$. Calcule los posibles valores de:<br />$\dfrac{x^2+y^2+z^2}{2(xy+yz+zx)}$. Notemos que $0=(x+y+z)^2=x^2+y^2+z^2+2(xy+yz+zx) $. Entonces: $2(xy+yz+zx)=-x^2-y^2-z^2$.Por lo tanto: $\dfrac{x^2+y^2+z^2}{2(xy+yz+zx)}=\dfrac{x^2+y^2+z^2}{-x^2-y^2-z^2}=-1$. Analicemos cuando queda indefinida Si:$ -x^2-y^2-z^2 =0$ ó $x^2+y^2+z^2=0$ Necesariamente $x=y=z=0$ ya que un cuadrado es mayor o igual a cero.$ Mensaje modificado por negroo el Dec 7 2008, 07:05 PM

negroo Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2008, 12:40 PM Publicado: #39
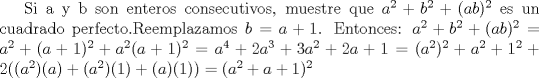Matemático Grupo: Usuario FMAT Mensajes: 32 Registrado: 8-November 08 Desde: chile Miembro Nº: 38.115 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: Si a y b son enteros consecutivos, muestre que $a^2+b^2+(ab)^2$ es un cuadrado perfecto.Reemplazamos $b=a+1$. Entonces: $a^2+b^2+(ab)^2=a^2+(a+1)^2+a^2(a+1)^2=a^4+2a^3+3a^2+2a+1=(a^2)^2+a^2+1^2+2((a^2)(a)+(a^2)(1)+(a<br />)(1))=(a^2+a+1)^2$$

negroo Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 5 2008, 01:05 PM Publicado: #40
Matemático Grupo: Usuario FMAT Mensajes: 32 Registrado: 8-November 08 Desde: chile Miembro Nº: 38.115 Nacionalidad: Colegio/Liceo: Sexo:	$TEX: Racionalizar:$\dfrac{1}{2\sqrt[3]{2}-5\sqrt[3]{5}}=\dfrac{(1)}{(2\sqrt[3]{2}-5\sqrt[3]{5})}\dfrac{((2\sqrt[3]{2})^2+(2\sqrt[3]{2})(5\sqrt[3]{5})+(5\sqrt[3]{5})^2)}{((2\sqrt[3]{2})^2+(2\sqrt[3]{2})(5\sqrt[3]{5})+(5\sqrt[3]{5})^2)}=\dfrac{4\sqrt[3]{4}+25\sqrt[3]{25}+10\sqrt[3]{10}}{(2\sqrt[3]{2})^3-(5\sqrt[3]{5})^3}=\dfrac{4\sqrt[3]{4}+25\sqrt[3]{25}+10\sqrt[3]{10}}{-609}$. Usé que $a^3-b^3=(a-b)(a^2+ab+b^2)$$

« Posterior · Material de Entrenamiento · Anterior »

6 Páginas:

« < 2 3 4 5 6 >

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 05:19 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.