Entrenamiento Algebra v2.0 - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Sector Preparación Olimpiadas > Sector PreOlímpico > Álgebra > Material de Entrenamiento

6 Páginas:

< 1 2 3 4 5 > »

Reply to this topic

Start new topic

Entrenamiento Algebra v2.0, [Medio]

Soul_Hunter Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 19 2008, 12:10 AM Publicado: #21
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 665 Registrado: 12-June 07 Desde: the city of the fallen angels Miembro Nº: 6.649 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent $\boxed{P_{11}}\\[5mm] \text{La suma de dos números es 21 y su producto es -7. Calcule:}$ <br />\begin{itemize}<br />\item \text{La suma de sus inversos}\\[5mm] $\dfrac1x+\dfrac1y=\dfrac{x+y}{xy}=\dfrac{21}{-7}=-3$<br />\item \text{La suma de sus cuartas potencias}\\[5mm] \text{Definamos:}\\[5mm] $\sigma_1=x+y=21\\ \sigma_2=xy=-7\\ S_n=x^n+y^n=\sigma_1S_{n-1}-\sigma_2S_{n-2}\\[5mm] \text{Entonces:}\\[5mm] S_0=x^0+y^0=2\\ S_1=x+y=21\\ S_2=x^2+y^2=21\cdot21+7\cdot2=455\\S_3=x^3+y^3=21\cdot455+7\cdot21=9702\\ S_4=x^4+y^4=21\cdot9702+7\cdot455=206927$<br />\end{itemize}$ Salu2 Mensaje modificado por Apolonio el Aug 19 2008, 07:17 PM --------------------

Soul_Hunter Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 19 2008, 12:33 AM Publicado: #22
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 665 Registrado: 12-June 07 Desde: the city of the fallen angels Miembro Nº: 6.649 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent \boxed{P_{51}} \\[5mm] Resuelva el sistema de ecuaciones\\[5mm] \begin{equation}\begin{aligned}2x_1+x_2+x_3+x_4+x_5&=6\\ x_1+2x_2+x_3+x_4+x_5&=12\\ x_1+x_2+2x_3+x_4+x_5&=24\\ x_1+x_2+x_3+2x_4+x_5&=48\\ x_1+x_2+x_3+x_4+2x_5&=96\end{aligned}\end{equation}\\[5mm] \text{Sumemos todas las igualdades:}\\[5mm] \begin{equation}\begin{aligned}6x_1+6x_2+6x_3+6x_4+6x_5&=186\qquad\Big/ \cdot \frac16\\ x_1+x_2+x_3+x_4+x_5&=31\end{aligned}\end{equation}\\ \text{Volvamos a la primera ecuación:}\\[5mm] \begin{equation}\begin{aligned}2x_1+x_2+x_3+x_4+x_5&=6\\ x_1+(x_1+x_2+x_3+x_4+x_5)&=6\qquad\Big/\text{reemplazamos}\\ x_1+31&=6\\ x_1&=-25\end{aligned}\end{equation}\\[5mm] \text{Análogamente...}\\[5mm] \begin{equation}\begin{aligned} x_2&=12-31=-19\\ x_3&=24-31=-7\\ x_4&=48-31=17\\ x_5&=96-31=65\end{aligned}\end{equation}$ Salu2 PS: lo que es tener biología dos veces en un día... --------------------

Soul_Hunter Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 19 2008, 12:44 AM Publicado: #23
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 665 Registrado: 12-June 07 Desde: the city of the fallen angels Miembro Nº: 6.649 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	El último de la noche... $TEX: \noindent \boxed{P_3}\\[5mm] \textbf{Factorice} $a^4+4b^4$ \\[5mm] \text{Sumemos un conveniente cero}\\[5mm] $a^4+4b^4+(4a^2b^2-4a^2b^2)=(a^2+2b^2)^2-4a^2b^2$\\[5mm] \text{Factorizamos la diferencia de cuadrados como "suma por diferencia"}\\[5mm] $(a^2+2b^2)^2-4a^2b^2=(a^2+2b^2+2ab)(a^2+2b^2-2ab)$$ Buenas noches --------------------

Soul_Hunter Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 21 2008, 10:00 PM Publicado: #24
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 665 Registrado: 12-June 07 Desde: the city of the fallen angels Miembro Nº: 6.649 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \noindent \boxed{P_{23}}\\[5mm]<br />\textbf{Probar que} $\sqrt[3]{2+\sqrt5}+\sqrt[3]{2-\sqrt5}$ \textbf{es un número racional}\\[5mm]<br />Es evidente que si nos ponemos a elevar toda la expresión al cubo llegaremos a algo más desagradable aun, por eso es mejor buscar un número que al cubo nos dé algo similar a $2\pm\sqrt5$. Por ello debemos notar que:<br />$$\dfrac{(1\pm\sqrt5)^3}{8}=2\pm\sqrt5$$ con lo que ahora sólo nos resta reemplazar este valor en la expresión inicial\\[5mm] $\sqrt[3]{2+\sqrt5}+\sqrt[3]{2-\sqrt5}=\sqrt[3]{\dfrac{(1+\sqrt5)^3}{8}}+\sqrt[3]{\dfrac{(1-\sqrt5)^3}{8}}=\dfrac{1+\sqrt5}{2}+\dfrac{1-\sqrt5}{2}=1$ \\[5mm] y como el 1 es un número racional, hemos demostrado lo pedido.$ --------------------

FrY Ver Perfil del Miembro Ver los mensajes de este miembro	Aug 21 2008, 11:15 PM Publicado: #25
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 509 Registrado: 15-June 08 Desde: ??? Miembro Nº: 27.257 Nacionalidad: Sexo:	Excelente guía Mensaje modificado por FrY el Dec 29 2008, 03:30 PM --------------------

DoomH~ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 10:27 PM Publicado: #26
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.429 Registrado: 6-October 07 Miembro Nº: 10.987	$TEX: \[<br />\begin{gathered}<br /> \boxed{P_{49} } \hfill \\<br /> \left( {\sqrt 5 + \sqrt 6 + \sqrt 7 } \right)\left( {\sqrt 5 + \sqrt 6 - \sqrt 7 } \right)\left( {\sqrt 5 - \sqrt 6 + \sqrt 7 } \right)\left( { - \sqrt 5 + \sqrt 6 + \sqrt 7 } \right) \hfill \\<br /> {\text{Sea }}x = \sqrt 5 ,{\text{ }}y = \sqrt 6 \wedge z = \sqrt 7 ,{\text{ luego}} \hfill \\<br /> \left( {x + y + z} \right)\left( {x + y - z} \right)\left( {x - y + z} \right)\left( { - x + y + z} \right) \hfill \\<br /> = \left[ {\left( {x + y + z} \right)\left( {x + y - z} \right)} \right]\left[ {\left( {x - y + z} \right)\left( { - x + y + z} \right)} \right] \hfill \\<br /> = \left( {x^2 + xy - xz + xy + y^2 - yz + xz + yz - z^2 } \right)\left[ {\left( {x - y + z} \right)\left( { - x + y + z} \right)} \right] \hfill \\<br /> = \left( {x^2 + 2xy + y^2 - z^2 } \right)\left( { - x^2 + xy + xz + yx - y^2 - yz - xz + yz + z^2 } \right) \hfill \\<br /> = \left( {x^2 + 2xy + y^2 - z^2 } \right)\left( { - x^2 + 2xy - y^2 + z^2 } \right) \hfill \\<br /> = \left( {x^2 + 2xy + y^2 - z^2 } \right)\left( {2xy - y^2 + z^2 - x^2 } \right) \hfill \\<br /> {\text{Reemplazando nuevamente con las variables originales}} \hfill \\<br /> \left( {\sqrt {5^2 } + 2\sqrt 5 \sqrt 6 + \sqrt {6^2 } - \sqrt {7^2 } } \right)\left( {2\sqrt 5 \sqrt 6 - \sqrt {6^2 } + \sqrt {7^2 } - \sqrt {5^2 } } \right) \hfill \\<br /> = \left( {5 + 2\sqrt {30} + 6 - 7} \right)\left( {2\sqrt {30} - 6 + 7 - 5} \right) \hfill \\<br /> = \left( {2\sqrt {30} + 5 + 6 - 7} \right)\left( {2\sqrt {30} - 6 + 7 - 5} \right) \hfill \\<br /> = \left( {2\sqrt {30} + 4} \right)\left( {2\sqrt {30} - 4} \right) \hfill \\<br /> {\text{Como esta ultima expresion}}{\text{, equivale a una Suma por su Diferencia}}{\text{, se tiene }} \hfill \\<br /> {\text{que}} \hfill \\<br /> \left( {2\sqrt {30} + 4} \right)\left( {2\sqrt {30} - 4} \right) \hfill \\<br /> = 4 \bullet 30 - 16 \hfill \\<br /> = 120 - 16 \hfill \\<br /> = 104 \hfill \\ <br />\end{gathered} <br />\]$ Salu2 -------------------- CHAO.

DoomH~ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 10:36 PM Publicado: #27
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.429 Registrado: 6-October 07 Miembro Nº: 10.987	$TEX: \[<br />\begin{gathered}<br /> \boxed{P_{24} } \hfill \\<br /> 123456789^2 - 123456790 \times 123456788 \hfill \\<br /> {\text{Sea }}k = 123456789{\text{ }}{\text{, luego}} \hfill \\<br /> k^2 - \left( {k + 1} \right)\left( {k - 1} \right) \hfill \\<br /> = k^2 - \left( {k^2 - 1} \right) \hfill \\<br /> = k^2 - k^2 + 1 \hfill \\<br /> = 1 \hfill \\ <br />\end{gathered} <br />\]$ Salu2 -------------------- CHAO.

DoomH~ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 21 2008, 10:45 PM Publicado: #28
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.429 Registrado: 6-October 07 Miembro Nº: 10.987	$TEX: \[<br />\begin{gathered}<br /> \boxed{P_9 } \hfill \\<br /> x + \frac{1}<br />{x} = 5/()^2 \hfill \\<br /> x^2 + 2x \bullet \frac{1}<br />{x} + \frac{1}<br />{{x^2 }} = 25 \hfill \\<br /> x^2 + 2 + \frac{1}<br />{{x^2 }} = 25 \hfill \\<br /> \boxed{x^2 + \frac{1}<br />{{x^2 }} = 23} \hfill \\ <br />\end{gathered} <br />\]$ Salu2 -------------------- CHAO.

DoomH~ Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 23 2008, 09:13 AM Publicado: #29
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 1.429 Registrado: 6-October 07 Miembro Nº: 10.987	Problema 25 resuelto aquí: http://www.fmat.cl/index.php?showtopic=303...mp;#entry211719 Salu2 -------------------- CHAO.

S. E. Puelma Moy... S. E. Puelma Moya Ver Perfil del Miembro Ver los mensajes de este miembro	Oct 25 2008, 01:13 PM Publicado: #30
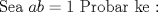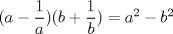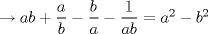Dios Matemático Supremo Grupo: Administrador Mensajes: 2.706 Registrado: 13-May 05 Desde: Santiago de Chile Miembro Nº: 10 Nacionalidad: Colegio/Liceo: Sexo:	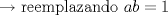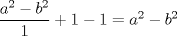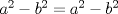CITA(gussy @ Aug 15 2008, 09:19 PM) $TEX: \fbox{Pregunta 7}$ $TEX: Sea $ab = 1$ Probar ke :$ $TEX: $(a-\dfrac{1}{a})(b+\dfrac{1}{b})=a^2-b^2$$ $TEX: $\rightarrow ab+\dfrac{a}{b}-\dfrac{b}{a}-\dfrac{1}{ab} = a^2-b^2$$ $TEX: $\dfrac{a^2-b^2}{ab}+ab-\dfrac{1}{ab}=a^2-b^2$$ $TEX: $\rightarrow$ reemplazando $ab=1$$ $TEX: $\dfrac{a^2-b^2}{1}+1-1=a^2-b^2$$ $TEX: $a^2-b^2=a^2-b^2$$ Estoy revisando todas las soluciones de este tema, el primero que voy a comentar es esta solución. Está correcta. Sin embargo, se hace más fácil con la siguiente observación: si ab=1, entonces 1/a = .......... -------------------- Sebastián Elías Puelma Moya Administrador FMAT

« Posterior · Material de Entrenamiento · Anterior »

6 Páginas:

< 1 2 3 4 5 > »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 6th March 2025 - 10:18 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.