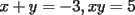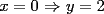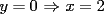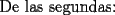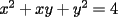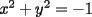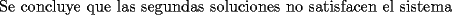|
|
|
|
|
|
|
  |
 Mar 20 2006, 11:43 PM Mar 20 2006, 11:43 PM
Publicado:
#1
|
|
|
Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad:  Sexo:  |
 Saludos -------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!)
Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)   |
|
|
|
 Apr 16 2006, 10:21 AM Apr 16 2006, 10:21 AM
Publicado:
#2
|
|
|
Maestro Matemático Grupo: Usuario FMAT Mensajes: 73 Registrado: 13-April 06 Miembro Nº: 841 |
Idea:
|
|
|
|
 Apr 27 2006, 08:51 AM Apr 27 2006, 08:51 AM
Publicado:
#3
|
|
|
Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad:  Sexo:  |
CITA(hpoincare @ Apr 16 2006, 11:21 AM) Lo entregado hasta ahora es basicamente la solucion al problema, sin embargo aun falta concluir y por ende aun este problema no esta resuelto hasta que alguien entregue el final de este problemita... Saludos PD: Como ya fue mostrado antes, muchas veces los cambios de variables suelen ser utiles en los sistemas de ecuaciones... -------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!)
Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)   |
|
|
|
 Jun 18 2006, 11:05 PM Jun 18 2006, 11:05 PM
Publicado:
#4
|
|
 Dios Matemático Grupo: Usuario FMAT Mensajes: 228 Registrado: 8-August 05 Desde: Santiago de Chile Miembro Nº: 194 |
|
|
|
|
 Jun 19 2006, 12:57 PM Jun 19 2006, 12:57 PM
Publicado:
#5
|
|
 Staff FMAT Grupo: Super Moderador Mensajes: 8.124 Registrado: 21-May 06 Miembro Nº: 1.156 Nacionalidad:  Sexo:  |
Bueno acá va
![TEX: \[<br />\begin{gathered}<br /> x^2 + xy + y^2 = 4 \hfill \\<br /> x + xy + y = 2 \Leftrightarrow y(x + 1) = 2 - x \Leftrightarrow y = \frac{{2 - x}}<br />{{x + 1}} \hfill \\<br /> x^2 + x\left( {\frac{{2 - x}}<br />{{x + 1}}} \right) + \left( {\frac{{2 - x}}<br />{{x + 1}}} \right)^2 = 4 \Leftrightarrow x^2 + \frac{{2 - x}}<br />{{x + 1}}\left( {x + \frac{{2 - x}}<br />{{x + 1}}} \right) = 4 \hfill \\<br /> x^2 + \frac{{2 - x}}<br />{{x + 1}}\left( {\frac{{x^2 + x + 2 - x}}<br />{{x + 1}}} \right) = 4 \hfill \\<br /> x^2 - \frac{{(x - 2)(x^2 + 2)}}<br />{{x^2 + 2x + 1}} = 4 \Leftrightarrow x^4 + 2x^3 + x^2 - x^3 - 2x + 2x^2 = 4x^2 + 8x \hfill \\<br /> x^4 + x^3 - x^2 - 10x = 0 \Leftrightarrow x(x^3 + x^2 - x - 10) = 0 \Leftrightarrow x = 0 \hfill \\<br /> x^3 + x^2 - x - 10 = 0 \Leftrightarrow (x - 2)(x^2 + 3x + 5) = 0 \Leftrightarrow x = 2 \hfill \\ <br />\end{gathered} <br />\]](./tex/5b144a03d28761f71c97f45fbd3a7ba5.png) Solución productiva: ![TEX: \[<br />(0,2)<br />\]](./tex/b8fe82a2668e9147ce5a805b22fa1fa5.png) y y ![TEX: \[<br />(2,0)<br />\]](./tex/58eb49a503f00e519a91b491b907f43e.png)
|
|
|
|
 Dec 6 2006, 11:18 PM Dec 6 2006, 11:18 PM
Publicado:
#6
|
|
 Matemático Grupo: Usuario FMAT Mensajes: 68 Registrado: 9-August 05 Desde: Rancagua Miembro Nº: 206 Nacionalidad:  Sexo:  |
A ver... mi primer post usando latex =P.
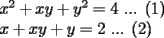 ![TEX: (1)+(2)<br /><br />$x^2+2xy+y^2+x+y=6$<br /><br />$(x+y)^2+(x+y)-6=0$<br /><br />$[(x+y)+3][(x+y)-2]=0$](./tex/155190.gif) 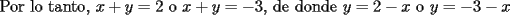  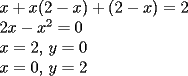 Ja nee!! |
|
|
|
 Jun 12 2008, 09:35 PM Jun 12 2008, 09:35 PM
Publicado:
#7
|
|
|
Doctor en Matemáticas Grupo: Usuario FMAT Mensajes: 153 Registrado: 27-March 08 Desde: calle santa maria 221 Miembro Nº: 18.096 Nacionalidad:  Colegio/Liceo:  Sexo:  |
yo creo x + y =2-4 entonces x + y = -2 -------------------- manuxxx
|
|
|
|
  |
1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))
0 miembro(s):
| Versión Lo-Fi | Fecha y Hora actual: 19th October 2025 - 11:56 AM |










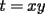 .
.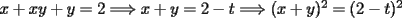
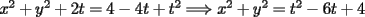
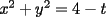
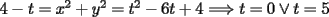
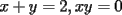 o bien
o bien