Sistema Diabólico - Foro fmat.cl

Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Álgebra > Desigualdades e Inecuaciones

Sistema Diabólico, Un problema para el olvido

Opciones

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	May 25 2008, 03:57 PM Publicado: #1
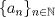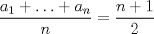Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	Sea $TEX: $\displaystyle \{a_{n}\}_{n \in \mathbb{N}}$$ una sucesión de números reales. Supongamos que dicha sucesión satisface la identidad $TEX: $\displaystyle \frac{a_{1} + \ldots + a_{n}}{n} = \frac{n+1}{2}$$ para cada 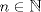$TEX: $n \in \mathbb{N}$$ . Determine (bajo tales condiciones) el conjunto solución del sistema de desigualdades siguiente: 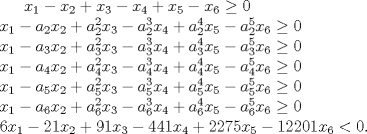$TEX: <br />$x_{1} - x_{2} + x_{3} - x_{4} + x_{5} - x_{6} \geq 0$\\<br />$x_{1} - a_{2}x_{2} + a_{2}^{2}x_{3} - a_{2}^{3}x_{4} + a_{2}^{4}x_{5} - a_{2}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{3}x_{2} + a_{3}^{2}x_{3} - a_{3}^{3}x_{4} + a_{3}^{4}x_{5} - a_{3}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{4}x_{2} + a_{4}^{2}x_{3} - a_{4}^{3}x_{4} + a_{4}^{4}x_{5} - a_{4}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{5}x_{2} + a_{5}^{2}x_{3} - a_{5}^{3}x_{4} + a_{5}^{4}x_{5} - a_{5}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{6}x_{2} + a_{6}^{2}x_{3} - a_{6}^{3}x_{4} + a_{6}^{4}x_{5} - a_{6}^{5}x_{6}\geq 0$\\<br />$6x_{1} - 21x_{2} + 91x_{3} - 441x_{4} + 2275x_{5} - 12201x_{6} < 0.$<br />$ Una propuesta original de un servidor de ustedes. Obviamente, hay una forma rápida de llegar a la solución. -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

Rurouni Kenshin Ver Perfil del Miembro Ver los mensajes de este miembro	May 25 2008, 04:22 PM Publicado: #2
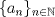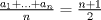Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad: Sexo:	CITA(coquitao @ May 25 2008, 03:51 PM) Sea $TEX: $\{a_{n}\}_{n \in \mathbb{N}}$$ una sucesión de números reales. Supongamos que dicha sucesión satisface la identidad $TEX: $\frac{a_{1} + \ldots + a_{n}}{n} = \frac{n+1}{2}$$ para cada $TEX: $n \in \mathbb{N}$$ . Determine (bajo tales condiciones) el conjunto solución del sistema de desigualdades siguiente: 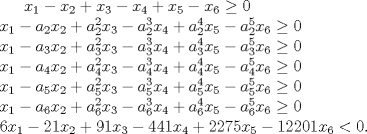$TEX: <br />$x_{1} - x_{2} + x_{3} - x_{4} + x_{5} - x_{6} \geq 0$\\<br />$x_{1} - a_{2}x_{2} + a_{2}^{2}x_{3} - a_{2}^{3}x_{4} + a_{2}^{4}x_{5} - a_{2}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{3}x_{2} + a_{3}^{2}x_{3} - a_{3}^{3}x_{4} + a_{3}^{4}x_{5} - a_{3}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{4}x_{2} + a_{4}^{2}x_{3} - a_{4}^{3}x_{4} + a_{4}^{4}x_{5} - a_{4}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{5}x_{2} + a_{5}^{2}x_{3} - a_{5}^{3}x_{4} + a_{5}^{4}x_{5} - a_{5}^{5}x_{6} \geq 0$\\<br />$x_{1} - a_{6}x_{2} + a_{6}^{2}x_{3} - a_{6}^{3}x_{4} + a_{6}^{4}x_{5} - a_{6}^{5}x_{6}\geq 0$\\<br />$6x_{1} - 21x_{2} + 91x_{3} - 441x_{4} + 2275x_{5} - 12201x_{6} < 0.$<br />$ Una propuesta original de un servidor de Ustedes. Obviamente, hay una forma rápida de llegar a la solución. 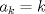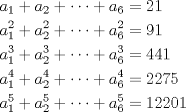Si notamos que $TEX: $a_1=1, a_2=2,...,a_6=6$$ (en general $TEX: $a_k=k$$ , cuya deduccion queda para el atento lector), tendremos tambien que $TEX: \begin{equation}<br />\begin{aligned}<br />a_1+a_2+\cdots +a_6&=21\\<br />a_1^2+a_2^2+\cdots +a_6^2&=91\\<br />a_1^3+a_2^3+\cdots +a_6^3&=441\\<br />a_1^4+a_2^4+\cdots +a_6^4&=2275\\<br />a_1^5+a_2^5+\cdots +a_6^5&=12201<br />\end{aligned}<br />\end{equation}$ Luego si multiplicamos la ultima ecuacion por -1 y en la primera ecuacion usamos que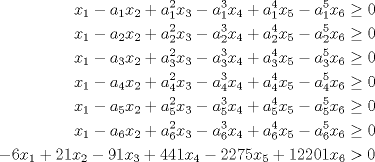 $TEX: $a_1=1$$ , nos quedara asi: $TEX: \begin{equation}<br />\begin{aligned}<br />x_{1} - a_1x_{2} + a_1^2x_{3} - a_1^3x_{4} + a_1^4x_{5} - a_1^5x_{6} &\geq 0\\<br />x_{1} - a_{2}x_{2} + a_{2}^{2}x_{3} - a_{2}^{3}x_{4} + a_{2}^{4}x_{5} - a_{2}^{5}x_{6} &\geq 0\\<br />x_{1} - a_{3}x_{2} + a_{3}^{2}x_{3} - a_{3}^{3}x_{4} + a_{3}^{4}x_{5} - a_{3}^{5}x_{6} &\geq 0\\<br />x_{1} - a_{4}x_{2} + a_{4}^{2}x_{3} - a_{4}^{3}x_{4} + a_{4}^{4}x_{5} - a_{4}^{5}x_{6} &\geq 0\\<br />x_{1} - a_{5}x_{2} + a_{5}^{2}x_{3} - a_{5}^{3}x_{4} + a_{5}^{4}x_{5} - a_{5}^{5}x_{6} &\geq 0\\<br />x_{1} - a_{6}x_{2} + a_{6}^{2}x_{3} - a_{6}^{3}x_{4} + a_{6}^{4}x_{5} - a_{6}^{5}x_{6} &\geq 0\\<br />-6x_{1} + 21x_{2} - 91x_{3} + 441x_{4} - 2275x_{5} + 12201x_{6} &> 0<br />\end{aligned}<br />\end{equation}$ Finalmente si sumamos todas las inecuaciones llegaremos a que 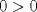$TEX: $0>0$$ (lo cual no es verdadero y por ende el sistema no tiene soluciones). En otras palabras, 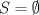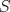$TEX: $S=\emptyset$$ ( $TEX: $S$$ es el conjunto solución) Saludos -------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!) Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)

coquitao Ver Perfil del Miembro Ver los mensajes de este miembro	May 26 2008, 02:06 PM Publicado: #3
Dios Matemático Supremo Grupo: Super Moderador Mensajes: 2.065 Registrado: 25-May 08 Desde: Pelotillehue Miembro Nº: 24.463	¡Fabuloso! ¿Puede dar alguien una solución más breve aún? Yo creo que sí. ¡Vamos por ella! -------------------- "Please forget everything you have learned in school; for you haven't learned it... Please keep in mind at all times the corresponding portions of your school curriculum; for you haven't actually forgotten them." -- E. Landau

xdanielx Ver Perfil del Miembro Ver los mensajes de este miembro	May 18 2009, 07:59 PM Publicado: #4
Dios Matemático Supremo Grupo: Baneado Mensajes: 3.360 Registrado: 11-March 08 Miembro Nº: 16.617	grande kenshin

« Posterior · Desigualdades e Inecuaciones · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 22nd December 2024 - 09:47 AM