Desafio IX - Foro fmat.cl

Portal Matemático

¿Qué es FMAT?

Foro fmat.cl > Programa Enseñanza Universitaria > Ejercitación > Desafíos > Problemas Resueltos

Reply to this topic

Start new topic

Desafio IX

Rurouni Kenshin Ver Perfil del Miembro Ver los mensajes de este miembro	Mar 6 2006, 09:35 PM Publicado: #1
Webmaster Grupo: Administrador Mensajes: 6.692 Registrado: 13-May 05 Desde: Santiago Centro Miembro Nº: 2 Nacionalidad: Sexo:	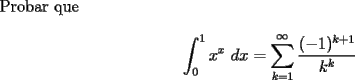$TEX: \noindent Probar que\\<br />$$\int_0^{1} x^x\ dx=\sum_{k=1}^{\infty}\frac{(-1)^{k+1}}{k^k}$$$ Suerte -------------------- Colegios/Liceos/Universidades en Fmat (Integrate!!!!) Videos PSU de Funciones (Y tú, ¿Aun estas aproblemado con Funciones?)

Mauro Ver Perfil del Miembro Ver los mensajes de este miembro	Jun 27 2006, 10:21 PM Publicado: #2
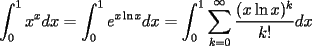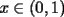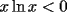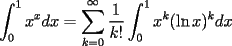Principiante Matemático Grupo: Usuario FMAT Mensajes: 11 Registrado: 26-January 06 Desde: Seattle, WA Miembro Nº: 513	Bonito Problema. Aquí va su solución: $TEX: $\displaystyle\int_{0}^{1} x^x dx = \int_{0}^{1}e^{x\ln x}dx = \int_{0}^{1} \sum_{k=0}^{\infty} \frac{(x\ln x)^k}{k!} dx$$ Dado que $TEX: $x\in (0,1)$$ se tiene que $TEX: $x\ln x<0$$ . Por teorema de convergencia monótona sigue que: $TEX: $\displaystyle\int_{0}^{1} x^x dx = \sum_{k=0}^{\infty} \frac{1}{k!} \int_{0}^{1} x^k(\ln x)^k dx$$ Calculemos dicha integral usando integración por partes: 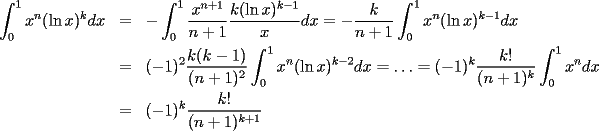$TEX: <br />\begin{eqnarray}<br />\int_{0}^{1}x^n(\ln x)^k dx &=&<br />-\int_{0}^{1}\frac{x^{n+1}}{n+1}\frac{k(\ln x)^{k-1}}{x}dx = -\frac{k}{n+1}\int_{0}^{1} x^n(\ln x)^{k-1}dx \\ <br />&=& (-1)^2\frac{k(k-1)}{(n+1)^2}\int_{0}^{1} x^n(\ln x)^{k-2}dx<br />= \ldots = (-1)^k \frac{k!}{(n+1)^k} \int_{0}^{1} x^ndx \\<br />&=& (-1)^k \frac{k!}{(n+1)^{k+1}}<br />\end{eqnarray}<br />$ Con lo que se concluye mediante un simple cambio de índice en la sumatoria.

master_c	Jun 12 2013, 02:22 PM Publicado: #3
Invitado	CITA(Kenshin @ Mar 6 2006, 09:35 PM) $TEX: \noindent Probar que\\<br />$$\int_0^{1} x^x\ dx=\sum_{k=1}^{\infty}\frac{(-1)^{k+1}}{k^k}$$$ Suerte esto es conocido cmo http://en.wikipedia.org/wiki/Sophomore's_dream

« Posterior · Problemas Resueltos · Anterior »

Reply to this topic

Start new topic

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 04:02 PM

Powered By IP.Board 2.2.1 © 2007 IPS, Inc.