4º control de matematicas I Programa de Bachillerato

Foro fmat.cl > Programa Enseñanza Universitaria > Comunidades Universitarias > Universidad de Chile > Matemáticas 1

4º control de matematicas I Programa de Bachillerato

Opciones

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Apr 21 2008, 07:44 PM Publicado: #1
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: \[<br />\begin{gathered}<br /> {\text{Cuarto control de Matematicas I}} \hfill \\<br /> {\text{Programa de Bachillerato}} \hfill \\<br /> \hfill \\<br /> {\text{Tiempo:20 minutos}} \hfill \\<br /> {\text{Elija solo un problema}} \hfill \\<br /> \hfill \\<br /> {\text{1}}{\text{. Encuentre todos los numeros}}{\text{, }}x \in \mathbb{R}{\text{ tales que:}} \hfill \\<br /> \hfill \\<br /> \left\| x \right\| < x^2 \hfill \\<br /> \hfill \\<br /> 2.{\text{Considera el conjunto:}} \hfill \\<br /> \hfill \\<br /> A = \left\{ {\frac{{n + 3}}<br />{{n + 1}}/n \in \mathbb{N}} \right\} \hfill \\<br /> \hfill \\<br /> {\text{Es A acotado? En caso afirmativo muestra una cota inferior y una inferior}} \hfill \\ <br />\end{gathered} <br />\]<br />$ mantienen la tonica, no estan dificiles la pregunta 1 se encuentra en este -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

user1 Ver Perfil del Miembro Ver los mensajes de este miembro	Apr 21 2008, 10:44 PM Publicado: #2
Dios Matemático Supremo Grupo: Usuario FMAT Mensajes: 619 Registrado: 2-April 07 Desde: :D Miembro Nº: 4.889	Ejercicio 2 $TEX: \[<br />\begin{gathered}<br /> {\text{Sea el conjunto }}A = \left\{ {\frac{{n + 3}}<br />{{n + 1}}{\text{ con }}n \in \mathbb{N}} \right\} \hfill \\<br /> \hfill \\<br /> {\text{Si sabemos que }}n \in \mathbb{N},{\text{ entonces }}n \geqslant 1,{\text{ asi resulta:}} \hfill \\<br /> \hfill \\<br /> n \geqslant 1{\text{ }}{\raise0.5ex\hbox{$\scriptstyle {}$}<br />\kern-0.1em/\kern-0.15em<br />\lower0.25ex\hbox{$\scriptstyle {}$}} + 2 \hfill \\<br /> n + 2 \geqslant 3{\text{ }}{\raise0.5ex\hbox{$\scriptstyle {}$}<br />\kern-0.1em/\kern-0.15em<br />\lower0.25ex\hbox{$\scriptstyle {}$}} + n \hfill \\<br /> 2n + 2 \geqslant n + 3{\text{ }} \hfill \\<br /> 2\left( {n + 1} \right) \geqslant n + 3{\text{ }}{\raise0.5ex\hbox{$\scriptstyle {}$}<br />\kern-0.1em/\kern-0.15em<br />\lower0.25ex\hbox{$\scriptstyle {}$}} \cdot \frac{1}<br />{{n + 1}} \hfill \\<br /> \frac{{n + 3}}<br />{{n + 1}} \leqslant 2 \hfill \\<br /> \hfill \\<br /> {\text{Veamos el otro}}{\text{, caso}}{\text{. Si tenemos que 0}} \leqslant {\text{2}}{\text{, entonces:}} \hfill \\<br /> \hfill \\<br /> {\text{0}} \leqslant {\text{2 }}{\raise0.5ex\hbox{$\scriptstyle {}$}<br />\kern-0.1em/\kern-0.15em<br />\lower0.25ex\hbox{$\scriptstyle {}$}} + \left( {n + 1} \right) \hfill \\<br /> n + 1 \leqslant 3 + n{\text{ }}{\raise0.5ex\hbox{$\scriptstyle {}$}<br />\kern-0.1em/\kern-0.15em<br />\lower0.25ex\hbox{$\scriptstyle {}$}}\frac{1}<br />{{n + 1}} \hfill \\<br /> 1 \leqslant \frac{{3 + n}}<br />{{n + 1}} \hfill \\<br /> \hfill \\<br /> {\text{Luego}}{\text{, por transitividad:}} \hfill \\<br /> \hfill \\<br /> 1 \leqslant \frac{{3 + n}}<br />{{n + 1}} \leqslant 2 \hfill \\<br /> \hfill \\<br /> {\text{Por lo tanto}}{\text{, 1 es cota inferior de }}A{\text{ e }}\inf \left\{ A \right\} = 1.{\text{ Por otro }} \hfill \\<br /> {\text{lado}}{\text{, todos los numeros mayores o iguales a 2 son cotas superiores de A}} \hfill \\<br /> {\text{por lo que }}\sup \left\{ A \right\} = 2. \hfill \\ <br />\end{gathered} <br />\]<br />$ Saludos

Lican Ver Perfil del Miembro Ver los mensajes de este miembro	Apr 27 2008, 04:04 AM Publicado: #3
Dios Matemático Grupo: Usuario FMAT Mensajes: 384 Registrado: 3-June 07 Miembro Nº: 6.396 Nacionalidad: Universidad: Sexo:	quien es tu profe de matematicas naxoo?

Naxoo Ver Perfil del Miembro Ver los mensajes de este miembro	Apr 27 2008, 01:09 PM Publicado: #4
Staff FMAT Grupo: Moderador Mensajes: 2.604 Registrado: 2-March 07 Desde: Somewhere over the rainbow Miembro Nº: 4.244 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	CITA(Lican @ Apr 27 2008, 04:58 AM) quien es tu profe de matematicas naxoo? Reyes, soy bachi-C en la 2 como solo nos preguntan que sea acotado solo basta con que encontremos cota inf y cota sup, propongo dos formas mas de encontrar cada cota $TEX: \[<br />\begin{gathered}<br /> \left. {\underline {\, <br /> {{\text{cota inferior}}} \,}}\! \right\| \hfill \\<br /> i)n > - 3 \hfill \\<br /> n + 3 > 0/ \cdot \frac{1}<br />{{n + 1}} \hfill \\<br /> \frac{{n + 3}}<br />{{n + 1}} > 0 \hfill \\<br /> \hfill \\<br /> ii)n + 3 > n + 1/ \cdot \frac{1}<br />{{n + 1}} \hfill \\<br /> \frac{{n + 3}}<br />{{n + 1}} > 1 \hfill \\<br /> \hfill \\<br /> \left. {\underline {\, <br /> {{\text{cota superior}}} \,}}\! \right\| \hfill \\<br /> \hfill \\<br /> i)n + 3 < 3n + 3/ \cdot \frac{1}<br />{{n + 1}} \hfill \\<br /> \frac{{n + 3}}<br />{{n + 1}} < 3 \hfill \\<br /> \hfill \\<br /> ii){\text{ la de ahora esta mas truculenta}}{\text{, notemos que}} \hfill \\ <br />\end{gathered} <br />\]<br />$ $TEX: \[<br />\begin{gathered}<br /> \frac{{n + 3}}<br />{{n + 1}} = 1 + \frac{2}<br />{{n + 1}} \hfill \\<br /> ahora: \hfill \\<br /> \hfill \\<br /> n + 1 > 1/ \cdot \frac{1}<br />{{n + 1}} \hfill \\<br /> 1 > \frac{1}<br />{{n + 1}}/ \cdot 2 \hfill \\<br /> 2 > \frac{2}<br />{{n + 1}}/ + 1 \hfill \\<br /> 3 > \frac{2}<br />{{n + 1}} + 1 = \frac{{n + 3}}<br />{{n + 1}} \Leftrightarrow \frac{{n + 3}}<br />{{n + 1}} < 3 \hfill \\<br /> \hfill \\ <br />\end{gathered} <br />\]<br />$ -------------------- INRIA - Francia, Sophia Antipolis Biocore Team Ingeniero Civil en Biotecnología Ingeniería Civil Químico “(…) los elementos que él (¿o Él?) [Dios] mismo nos ha dado (raciocinio, sensibilidad, intuición) no son en absoluto suficientes como para garantizarnos ni su existencia ni su no existencia. Gracias a una corazonada puedo creer en Dios y acertar o no creer en Dios y también acertar" Mario Benedetti $TEX: \[\iiint\limits_\Omega {\left( {\nabla \cdot \vec F} \right)dV} = \iint\limits_{\partial \Omega } {\left( {\vec F \cdot \hat n} \right)}dS\]<br />$

Mau_map Ver Perfil del Miembro Ver los mensajes de este miembro	Dec 7 2009, 10:08 AM Publicado: #5
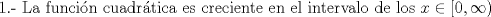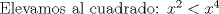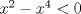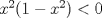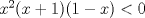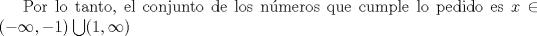Dios Matemático Grupo: Usuario FMAT Mensajes: 247 Registrado: 11-December 08 Miembro Nº: 40.674 Nacionalidad: Colegio/Liceo: Universidad: Sexo:	$TEX: 1.- La función cuadrática es creciente en el intervalo de los $x\in [0,\infty)$$ $TEX: Elevamos al cuadrado: $x^2<x^4$$ $TEX: $x^2-x^4<0$$ $TEX: $x^2(1-x^2)<0$$ $TEX: $x^2(x+1)(1-x)<0$$ $TEX: Por lo tanto, el conjunto de los números que cumple lo pedido es $x\in (-\infty,-1)\bigcup(1,\infty)$$ $TEX: O sea $x\in R-[-1,1]$$ Saludos

METABOLIC Ver Perfil del Miembro Ver los mensajes de este miembro	Jan 4 2010, 01:12 PM Publicado: #6
Principiante Matemático Grupo: Usuario FMAT Mensajes: 5 Registrado: 19-May 09 Miembro Nº: 51.679 Nacionalidad: Universidad: Sexo:	ESTA INTEDEZANTE EL CONTROL EJALE

« Posterior · Matemáticas 1 · Anterior »

1 usuario(s) está(n) leyendo esta discusión (1 invitado(s) y 0 usuario(s) anónimo(s))

0 miembro(s):

Modo de Ver: Estándar · Cambiar a: Lineal+ · Cambiar a: Outline

Suscribirse · Enviar por correo · Imprimir · Suscribirse a este foro

Versión Lo-Fi

Fecha y Hora actual: 23rd November 2024 - 06:52 PM